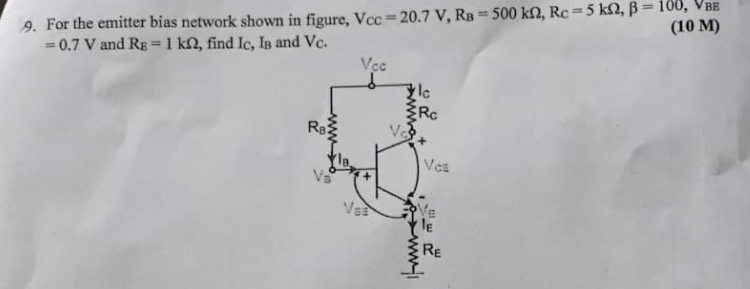
For the emitter bias network shown in figure, VCC = 20.7 V, RB = 500 kΩ, RC = 5 kΩ, β = 100, VBE = 0.7 V and RE = 1 kΩ, find Ic, IB and Vc.

Steps to Solve

1. Calculate Base Voltage ($V_B$)

Using the voltage divider rule, calculate the base voltage:

$$ V_B = V_{CC} \times \frac{R_B}{R_B + R_C} $$

Given that ( V_{CC} = 20.7,V ), ( R_B = 500,k\Omega ), and ( R_C = 5,k\Omega ):

$$ V_B = 20.7,V \times \frac{500,k\Omega}{500,k\Omega + 5,k\Omega} = 20.7,V \times \frac{500}{505} $$

2. Calculate Emitter Voltage ($V_E$)

The emitter voltage can be calculated using:

$$ V_E = V_B - V_{BE} $$

With ( V_B ) from the previous calculation and ( V_{BE} = 0.7,V ):

$$ V_E = V_B - 0.7,V $$

3. Calculate Emitter Current ($I_E$)

Using Ohm’s law to find emitter current through ( R_E ):

$$ I_E = \frac{V_E}{R_E} $$

Given ( R_E = 1,k\Omega ):

4. Calculate Collector Current ($I_C$)

Using the relationship between emitter current and collector current:

$$ I_C \approx I_E $$

Assuming ( \beta ) is large, we approximate ( I_C \approx I_E ).

5. Calculate Base Current ($I_B$)

The relationship between collector current and base current is given by:

$$ I_C = \beta I_B $$

Thus,

$$ I_B = \frac{I_C}{\beta} $$

6. Calculate Collector Voltage ($V_C$)

Finally, use Kirchhoff’s voltage law to find the collector voltage:

$$ V_C = V_{CC} - I_C \times R_C $$

Where ( R_C = 5,k\Omega ).