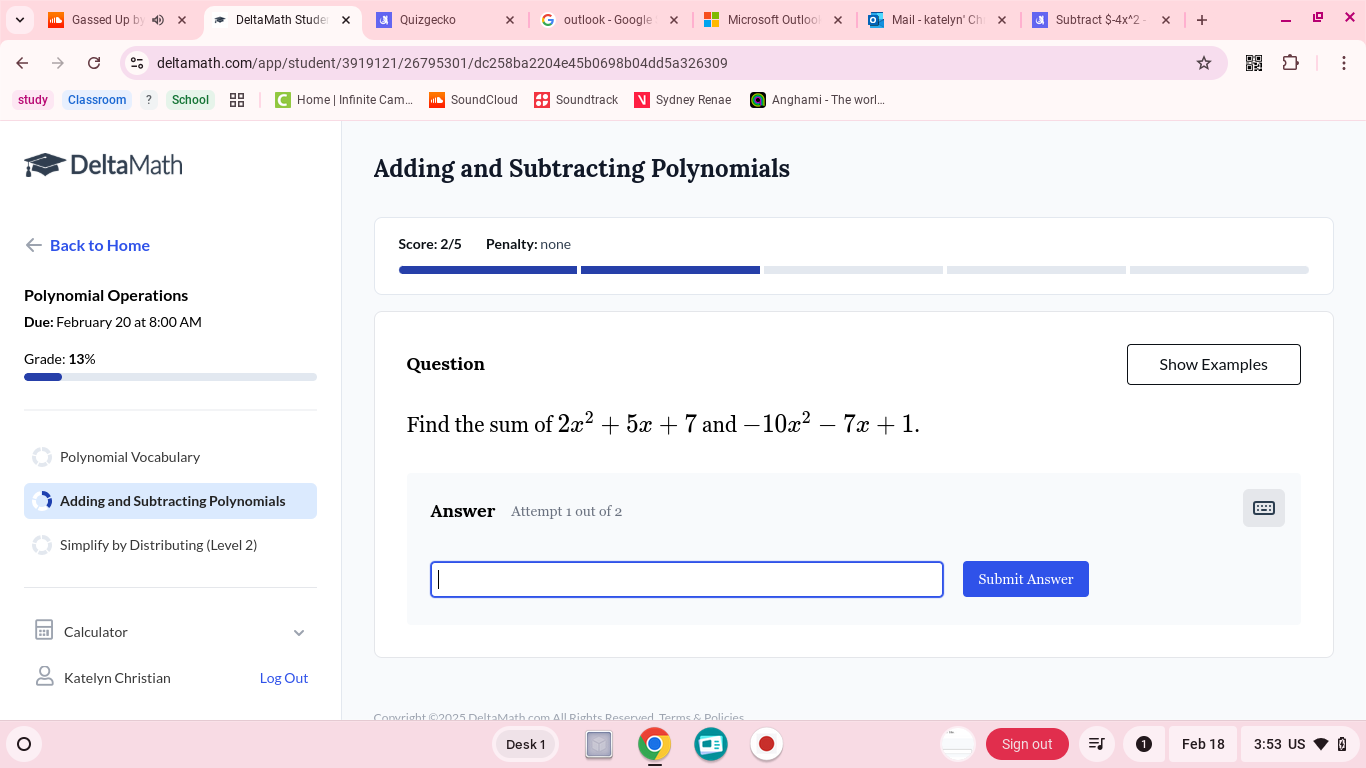
Find the sum of 2x² + 5x + 7 and -10x² - 7x + 1.
Understand the Problem
The question asks us to find the sum of two polynomials. This involves combining like terms, meaning terms with the same variable and exponent. We need to add the coefficients of the x² terms, the x terms, and the constant terms separately to find the resulting polynomial.
Answer
$-8x^2 - 2x + 8$
Answer for screen readers
$-8x^2 - 2x + 8$
Steps to Solve
- Identify like terms
The like terms are the $x^2$ terms, the $x$ terms, and the constant terms. In this case: $2x^2$ and $-10x^2$ are like terms. $5x$ and $-7x$ are like terms. $7$ and $1$ are like terms.
- Add the $x^2$ terms
Add the coefficients of the $x^2$ terms: $2 + (-10) = -8$. So, the sum of the $x^2$ terms is $-8x^2$.
- Add the $x$ terms
Add the coefficients of the $x$ terms: $5 + (-7) = -2$. So, the sum of the $x$ terms is $-2x$.
- Add the constant terms
Add the constant terms: $7 + 1 = 8$.
- Combine the results
Combine the sums of the $x^2$ terms, the $x$ terms, and the constant terms to form the resulting polynomial: $-8x^2 - 2x + 8$.
$-8x^2 - 2x + 8$
More Information
Polynomial addition involves combining like terms. Make sure to only add terms with the same variable and exponent.
Tips
A common mistake is to add terms that are not like terms. For example, adding the coefficient of the $x^2$ term to the coefficient of the $x$ term. Another common mistake is sign errors when adding negative coefficients.
AI-generated content may contain errors. Please verify critical information