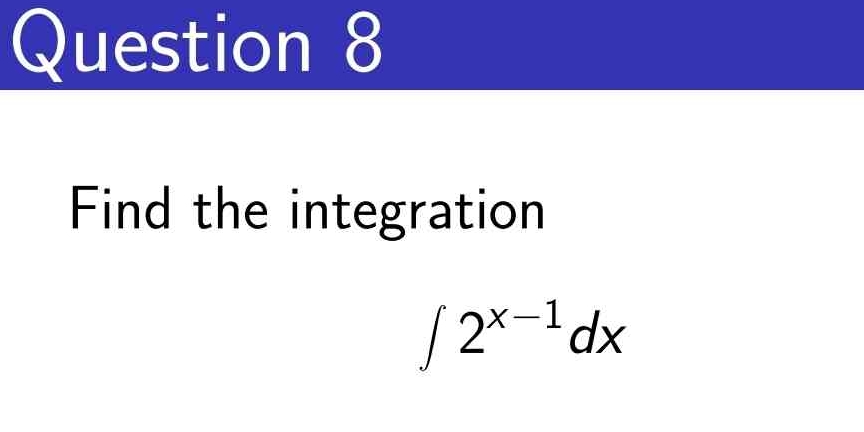
Find the integral of 2^(x-1) dx
Understand the Problem
The question asks to find the indefinite integral of the function 2^(x-1) with respect to x. This involves applying integration rules for exponential functions.
Answer
$\frac{2^x}{2\ln(2)} + C$
Answer for screen readers
$\frac{2^x}{2\ln(2)} + C$
Steps to Solve
-
Rewrite the integrand using exponent rules We can rewrite $2^{x-1}$ as $2^x \cdot 2^{-1}$, which simplifies to $\frac{1}{2} \cdot 2^x$.
-
Apply the constant multiple rule The integral of a constant times a function is the constant times the integral of the function: $$ \int \frac{1}{2} \cdot 2^x , dx = \frac{1}{2} \int 2^x , dx $$
-
Integrate the exponential function The integral of $a^x$ with respect to $x$ is $\frac{a^x}{\ln(a)} + C$, where $C$ is the constant of integration. In our case, $a = 2$, so $$ \int 2^x , dx = \frac{2^x}{\ln(2)} + C $$
-
Substitute back into the expression Substitute the result from step 3 back into the expression from step 2: $$ \frac{1}{2} \int 2^x , dx = \frac{1}{2} \cdot \frac{2^x}{\ln(2)} + C = \frac{2^x}{2\ln(2)} + C $$
$\frac{2^x}{2\ln(2)} + C$
More Information
The integral represents a family of functions whose derivative is $2^{x-1}$. The constant $C$ accounts for the fact that the derivative of a constant is zero, so there are infinitely many possible constant terms.
Tips
A common mistake is forgetting to divide by the natural logarithm of the base, $\ln(2)$, when integrating an exponential function. Also, it is important to remember the constant of integration, $C$, in indefinite integrals.
AI-generated content may contain errors. Please verify critical information