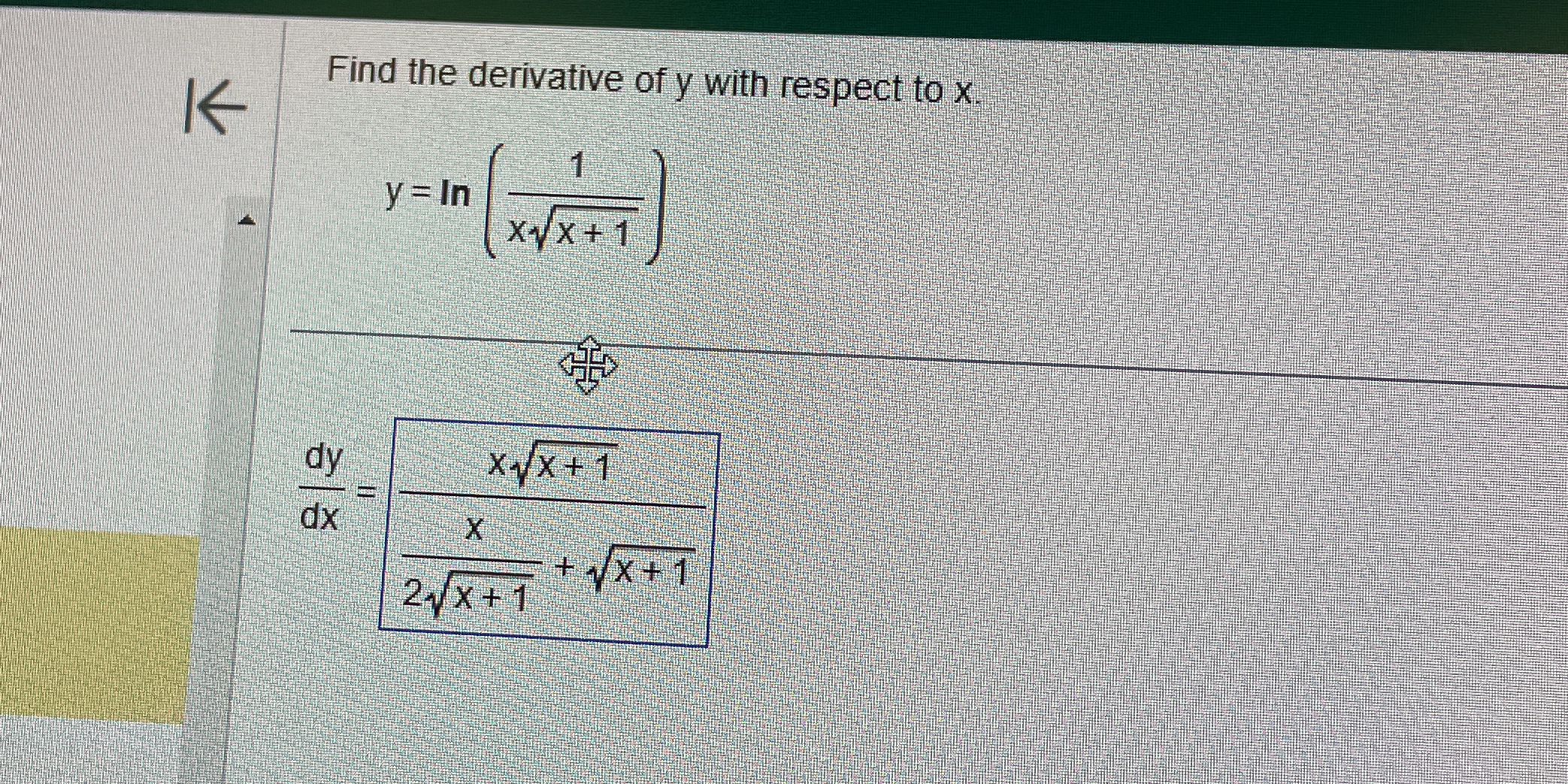
Find the derivative of y with respect to x, where y = ln(1 / (sqrt(x) / (x + 1))).

Steps to Solve

1. Rewrite the function
   Start with the function for clarification:
   $$ y = \ln \left(\frac{1}{\frac{\sqrt{x}}{x + 1}}\right) $$
   This can be rewritten using properties of logarithms:
   $$ y = \ln(1) - \ln\left(\frac{\sqrt{x}}{x + 1}\right) $$
   Since $ \ln(1) = 0$:
   $$ y = -\ln\left(\frac{\sqrt{x}}{x + 1}\right) $$

2. Apply the logarithm rule
   Use the quotient rule of logarithms:
   $$ y = - \left(\ln(\sqrt{x}) - \ln(x + 1)\right) $$
   This can be further simplified:
   $$ y = - \left(\frac{1}{2} \ln(x) - \ln(x + 1)\right) $$
   Thus,
   $$ y = -\frac{1}{2} \ln(x) + \ln(x + 1) $$

3. Differentiate the function
   Now, differentiate $y$ with respect to $x$:
   Using the derivative rules:
   $$ \frac{dy}{dx} = -\frac{1}{2} \cdot \frac{1}{x} + \frac{1}{x + 1} $$

4. Combine the fractions
   To combine the two terms, find a common denominator:
   $$ \frac{dy}{dx} = -\frac{1}{2x} + \frac{1}{x + 1} = \frac{-\frac{1}{2x}(x + 1) + \frac{1}{x + 1}(2x)}{(x + 1)} $$
   Simplify:
   $$ \frac{dy}{dx} = \frac{-\frac{x + 1}{2x} + \frac{2x}{x + 1}}{(x + 1)} $$
   Which can be further simplified for the final expression.