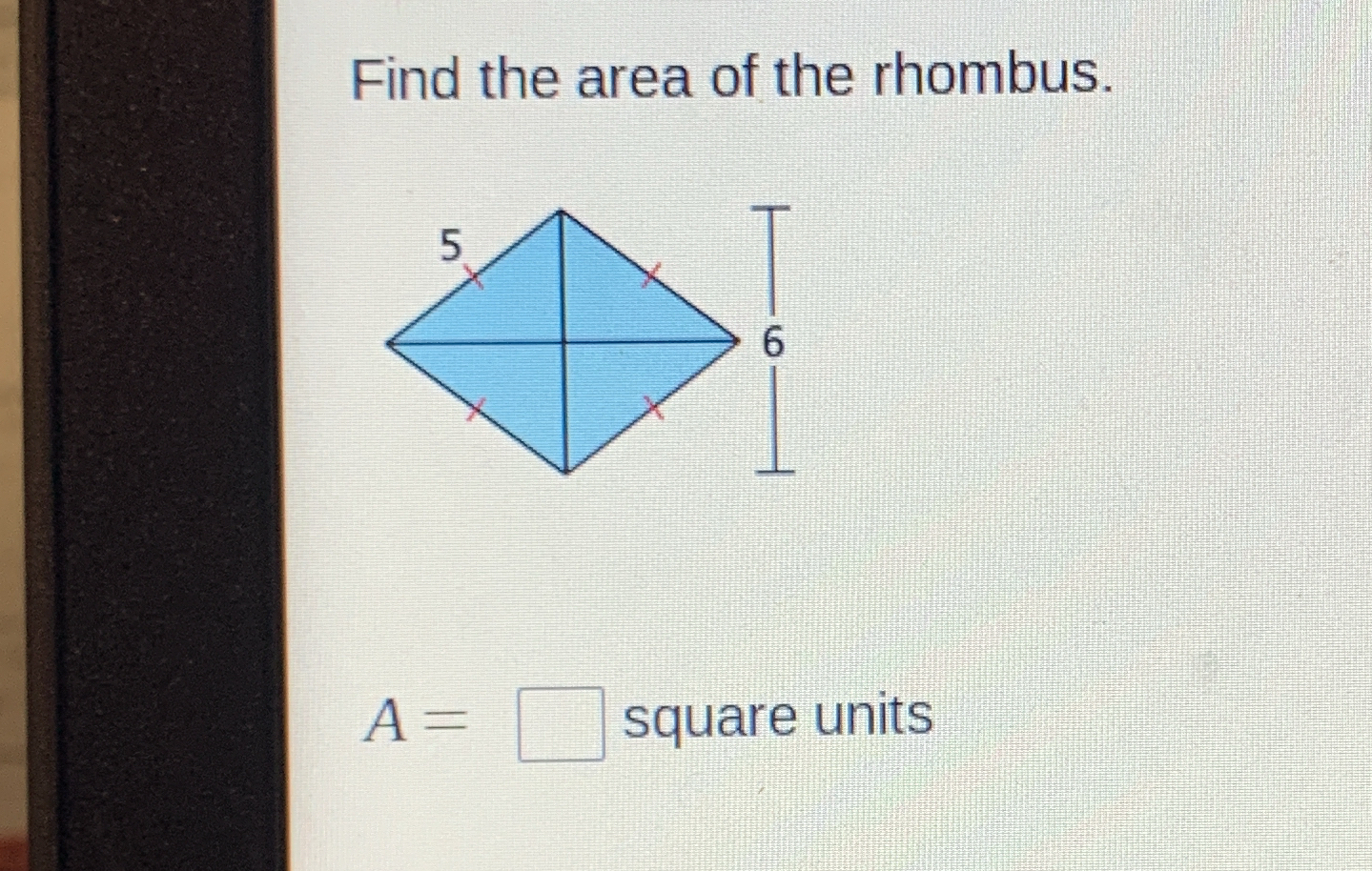
Find the area of the rhombus.
Understand the Problem
The question is asking us to find the area of a rhombus given its diagonals' lengths. The area of a rhombus can be calculated using the formula A = (d1 * d2) / 2, where d1 and d2 are the lengths of the diagonals.
Answer
The area of the rhombus is \( A = 15 \) square units.
Answer for screen readers
The area of the rhombus is ( A = 15 ) square units.
Steps to Solve
- Identify the lengths of the diagonals From the image, we see the lengths of the diagonals are given as 5 units and 6 units. We'll denote these lengths as:
- ( d_1 = 5 )
- ( d_2 = 6 )
-
Apply the area formula To find the area ( A ) of the rhombus, use the formula: $$ A = \frac{d_1 \times d_2}{2} $$
-
Substitute the values into the formula Plugging in the values of the diagonals: $$ A = \frac{5 \times 6}{2} $$
-
Calculate the product and divide First, calculate the product: $$ 5 \times 6 = 30 $$ Then divide by 2: $$ A = \frac{30}{2} = 15 $$
The area of the rhombus is ( A = 15 ) square units.
More Information
The area of a rhombus can also be found using the lengths of its diagonals, which makes it useful for problems where you have diagonal measurements instead of side lengths.
Tips
- Confusing the lengths of the diagonals. Always ensure that you're correctly identifying each diagonal's length.
- Forgetting to divide by 2 in the area formula. This is crucial for getting the correct area.
AI-generated content may contain errors. Please verify critical information