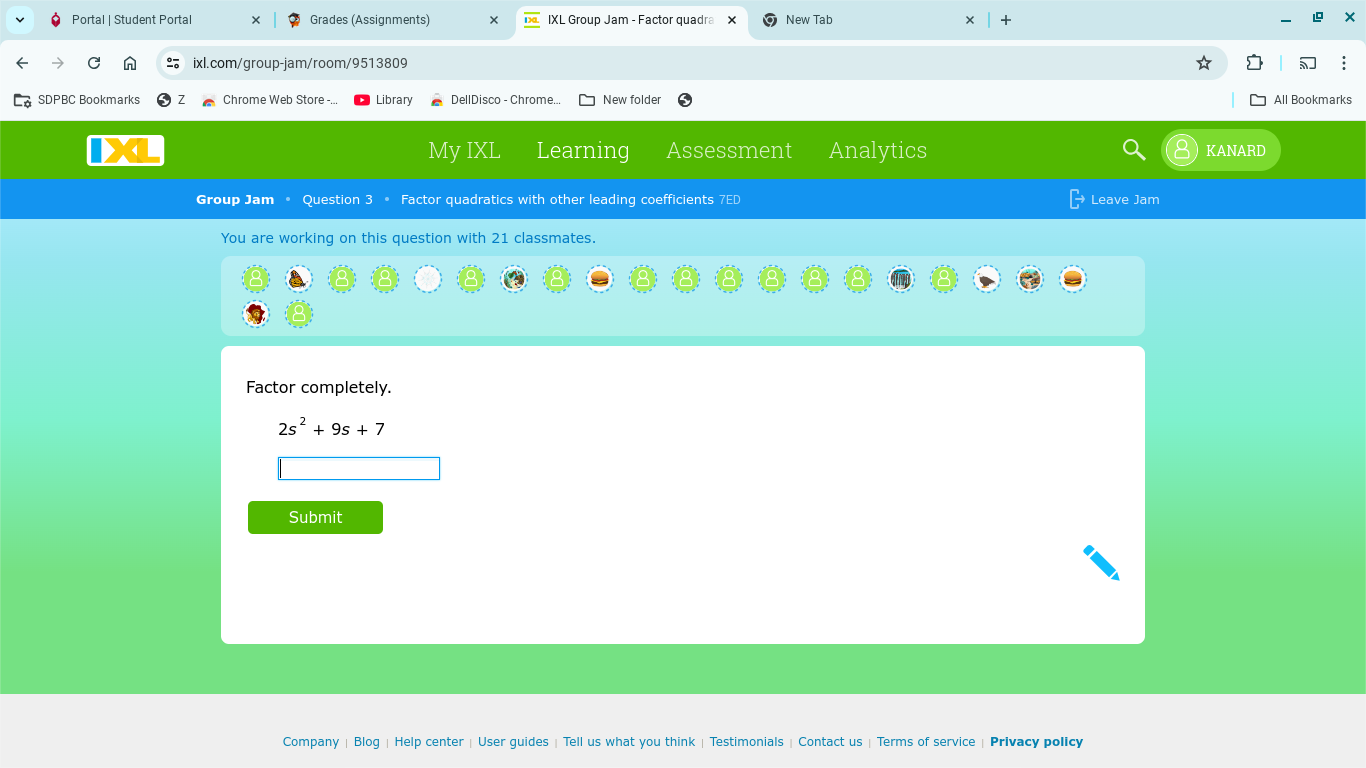
Factor completely. 2s² + 9s + 7
Understand the Problem
The question asks for the complete factorization of the quadratic expression 2s² + 9s + 7.
Answer
The factorization is $(2s + 7)(s + 1)$.
Answer for screen readers
The complete factorization of the quadratic expression $2s^2 + 9s + 7$ is $$(2s + 7)(s + 1)$$.
Steps to Solve
- Identify the coefficients of the quadratic expression
In the expression $2s^2 + 9s + 7$, the coefficients are:
- ( a = 2 ) (coefficient of $s^2$)
- ( b = 9 ) (coefficient of $s$)
- ( c = 7 ) (constant term)
- Calculate the product of ( a ) and ( c )
Multiply the coefficients ( a ) and ( c ): $$ ac = 2 \times 7 = 14 $$
- Find two numbers that multiply to ( ac ) and add to ( b )
We need to find two numbers that multiply to ( 14 ) and add to ( 9 ).
The numbers are ( 7 ) and ( 2 ):
- ( 7 \times 2 = 14 )
- ( 7 + 2 = 9 )
- Rewrite the middle term using the two numbers
Rewrite the expression by splitting the middle term: $$ 2s^2 + 7s + 2s + 7 $$
- Factor by grouping
Group the first two terms and the last two terms: $$ (2s^2 + 7s) + (2s + 7) $$
Factor out the common factors: $$ s(2s + 7) + 1(2s + 7) $$
- Combine the like terms
Factor out the common binomial factor: $$ (2s + 7)(s + 1) $$
The complete factorization of the quadratic expression $2s^2 + 9s + 7$ is $$(2s + 7)(s + 1)$$.
More Information
Factoring quadratics is an essential concept in algebra. Understanding how to identify coefficients, find suitable pairs of numbers, and apply grouping can greatly enhance problem-solving skills.
Tips
- Forgetting to check if the factors multiply correctly to ( ac ) and add to ( b ).
- Not grouping the terms correctly or overlooking common factors.
AI-generated content may contain errors. Please verify critical information