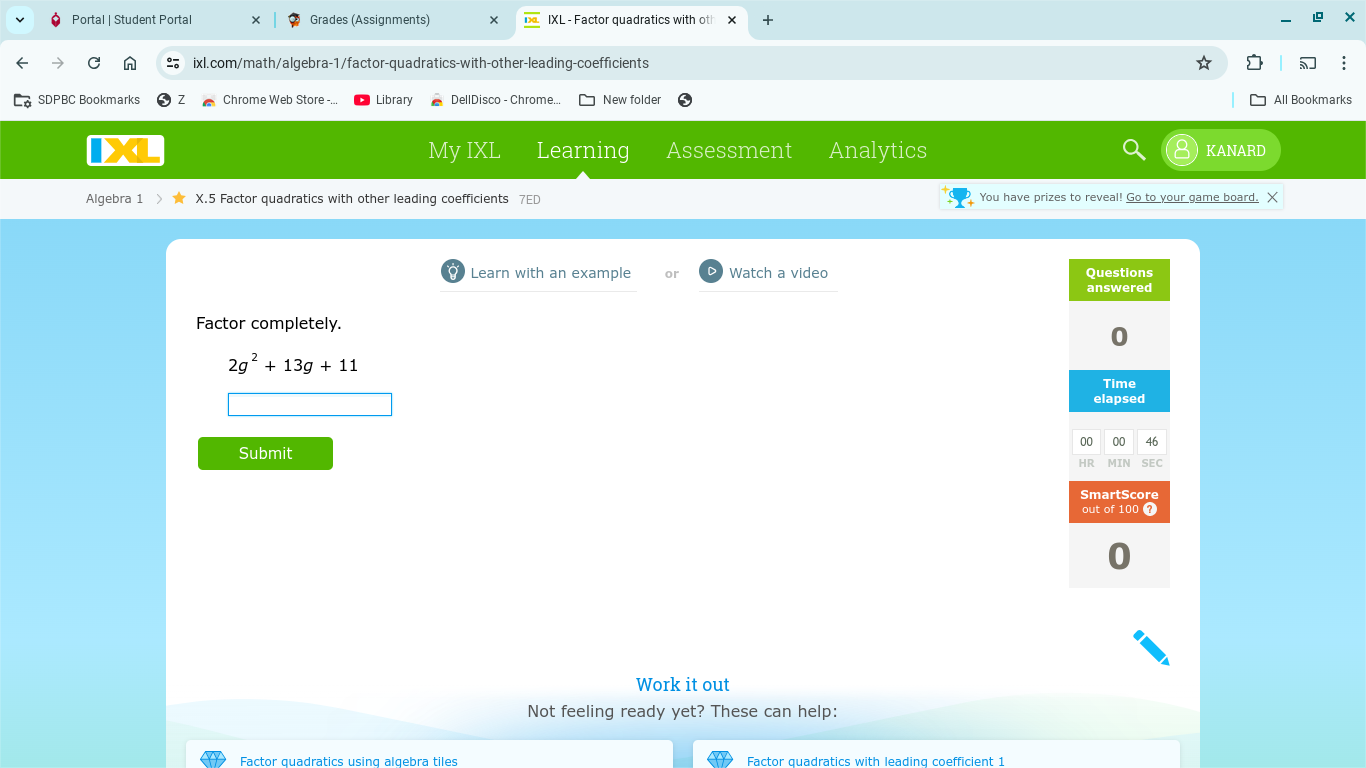
Factor completely: 2g² + 13g + 11.

Understand the Problem

The question is asking to factor the quadratic expression completely, specifically the expression 2g² + 13g + 11.

Answer

The factored form is $(g + 1)(2g + 11)$.

Steps to Solve

Identify the quadratic expression The given expression is $2g^2 + 13g + 11$. We want to factor this completely.
Multiply the leading coefficient by the constant term Multiply the coefficient of $g^2$ (which is 2) by the constant term (which is 11): $$ 2 \times 11 = 22 $$
Find two numbers that multiply and add We need to find two numbers that multiply to 22 (from the previous step) and add to 13 (the coefficient of $g$). The numbers are 2 and 11 because: $$ 2 \times 11 = 22 $$ $$ 2 + 11 = 13 $$
Rewrite the middle term using the two numbers Rewrite $13g$ in the expression $2g^2 + 13g + 11$ as $2g + 11g$: $$ 2g^2 + 2g + 11g + 11 $$
Group the terms Group the terms into pairs: $$ (2g^2 + 2g) + (11g + 11) $$
Factor out the common factors Factor out the common factors from each pair:

From the first group, factor out $2g$: $$ 2g(g + 1) $$
From the second group, factor out 11: $$ 11(g + 1) $$

Now the expression looks like this: $$ 2g(g + 1) + 11(g + 1) $$

Factor out the common binomial Now factor out the common binomial factor $(g + 1)$: $$ (g + 1)(2g + 11) $$

The factored form of the expression $2g^2 + 13g + 11$ is $(g + 1)(2g + 11)$.

More Information

Factoring quadratics is a fundamental skill in algebra. The process helps simplify expressions and solve equations. Quadratic expressions can often be factored into two linear expressions, which can then be used to find roots or solutions to equations.

Tips

Forgetting to multiply the leading coefficient by the constant term.
Misidentifying the two numbers that add up to the middle coefficient.
Not factoring completely or making mistakes in grouping terms.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!