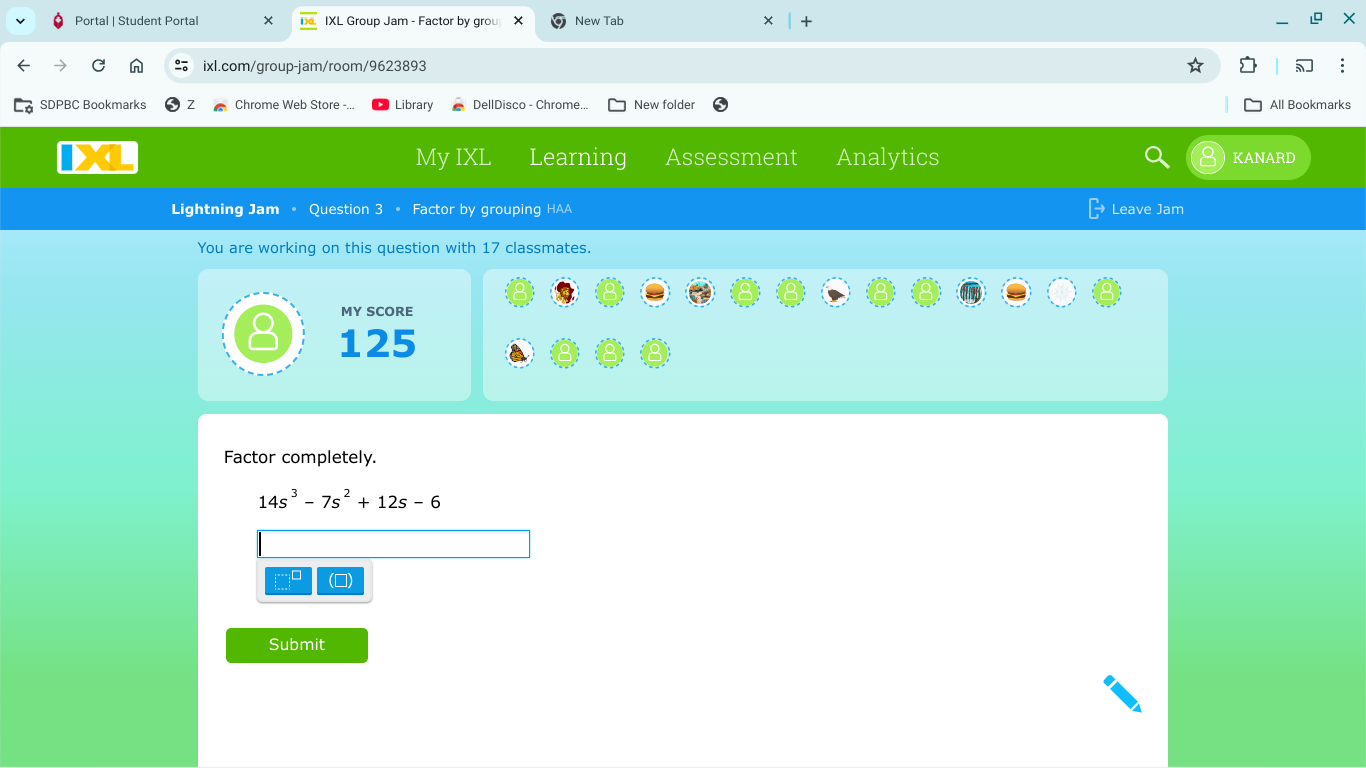
Factor completely. 14s^3 - 7s^2 + 12s - 6
Understand the Problem
The question is asking for a complete factorization of the polynomial expression given, specifically the expression 14s^3 - 7s^2 + 12s - 6. The goal will be to simplify this expression into a product of polynomials.
Answer
$$(2s - 1)(7s^2 + 6)$$
Answer for screen readers
The complete factorization of the polynomial $14s^3 - 7s^2 + 12s - 6$ is:
$$(2s - 1)(7s^2 + 6)$$
Steps to Solve
-
Group the terms for factoring Start by grouping the terms in pairs to prepare for factoring by grouping: $$ (14s^3 - 7s^2) + (12s - 6) $$
-
Factor out the common factors in each group Factor out the greatest common factor (GCF) from each group: From the first group: $7s^2(2s - 1)$
From the second group: $6(2s - 1)$
Now rewrite the expression: $$ 7s^2(2s - 1) + 6(2s - 1) $$
-
Factor out the common binomial factor Notice that $(2s - 1)$ is a common factor: $$ (2s - 1)(7s^2 + 6) $$
-
Check if the quadratic can be factored further The term $7s^2 + 6$ does not factor further over the integers. Therefore, this is the complete factorization.
The complete factorization of the polynomial $14s^3 - 7s^2 + 12s - 6$ is:
$$(2s - 1)(7s^2 + 6)$$
More Information
Factoring polynomials is a common process in algebra, where expressions are simplified into products of simpler polynomials or numbers. In this expression, we used factoring by grouping, which is effective when dealing with polynomials of four terms.
Tips
- Failing to identify common factors in the grouping step.
- Not checking if quadratic terms can be factored further.
- Forgetting to write the final factored form clearly.
AI-generated content may contain errors. Please verify critical information