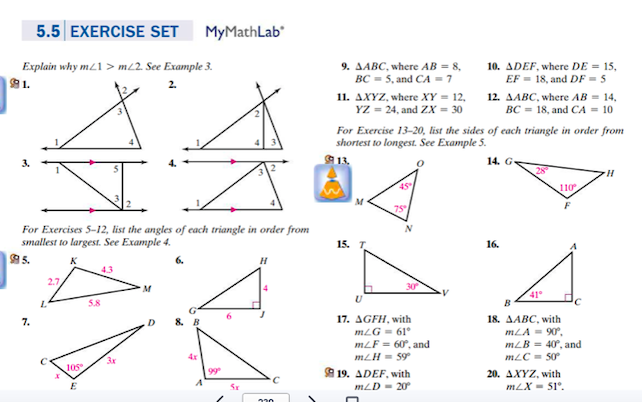
Explain why m∠1 > m∠2. See Example 3.
Understand the Problem
The question involves geometry and triangles, specifically asking to explain a relationship between angles and side lengths in a triangle. It's a request to understand properties of triangles and their angles based on given criteria.
Answer
$m∠1 > m∠2$ because the side opposite $m∠1$ is longer than the side opposite $m∠2$.
Answer for screen readers
$m∠1 > m∠2$ because the side opposite angle ( m∠1 ) is longer than the side opposite angle ( m∠2 ).
Steps to Solve
-
Identify the triangle and given relationships
In the provided triangle, we have segments marked as ( m∠1 ) and ( m∠2 ). We need to identify the relationship between these angles and the sides opposite them. -
Understanding the Angle-Side Relationship
According to the properties of triangles, in any triangle, the larger angle is opposite the longer side. We denote the sides opposite ( m∠1 ) and ( m∠2 ) as ( a ) and ( b ), respectively. Thus, if ( a > b ), it follows that ( m∠1 > m∠2 ). -
Applying the Triangle Angle Inequality
If we have sides ( a ) and ( b ) opposite angles ( m∠1 ) and ( m∠2 ), and we can determine from the diagram that ( a > b ), we can conclude that: $$ m∠1 > m∠2 $$
$m∠1 > m∠2$ because the side opposite angle ( m∠1 ) is longer than the side opposite angle ( m∠2 ).
More Information
This relationship reflects a fundamental theorem in triangle geometry known as the Angle-Side Relationship, which states that in triangle geometry, the length of a side is directly related to the size of the opposite angle.
Tips
- Confusing the relationship by assuming that if ( m∠1 ) is greater, then it must correspond to a shorter side. Remember that the opposite side length dictates the angle's magnitude.
- Misreading the diagram; ensure to check which angle corresponds to which side before making conclusions.
AI-generated content may contain errors. Please verify critical information