Expand and simplify 5(5x - 3) - 2(b + 5)
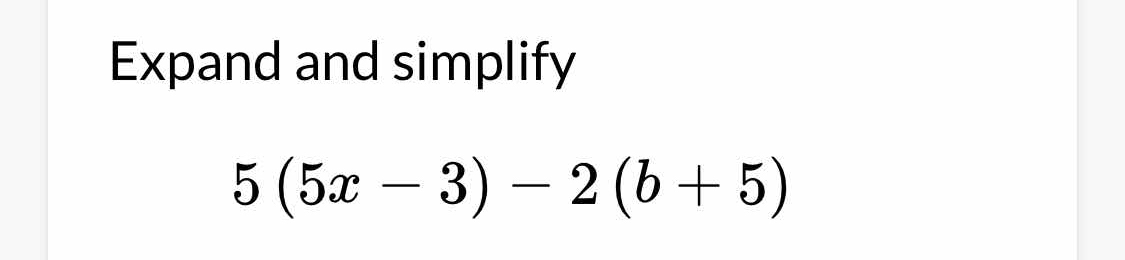
Understand the Problem
The question requires expanding and simplifying the given algebraic expression. This involves distributing the constants outside the parentheses to the terms inside, and then combining like terms to get the simplified expression.
Answer
$25x - 2b - 25$
Answer for screen readers
$25x - 2b - 25$
Steps to Solve
- Distribute the 5 into the first parenthesis
Multiply each term inside the first parenthesis by 5:
$5 * (5x - 3) = (5 * 5x) - (5 * 3) = 25x - 15$
- Distribute the -2 into the second parenthesis
Multiply each term inside the second parenthesis by -2:
$-2 * (b + 5) = (-2 * b) + (-2 * 5) = -2b - 10$
- Combine the results
Combine the results from step 1 and step 2:
$(25x - 15) + (-2b - 10) = 25x - 15 - 2b - 10$
- Combine like terms
Combine the constant terms: $-15 - 10 = -25$
- Final Answer
The simplified expression is: $25x - 2b - 25$
$25x - 2b - 25$
More Information
The original expression simplifies to $25x - 2b - 25$. There are no like terms to further simplify the result.
Tips
A common mistake is to forget to distribute the negative sign in the second term. For example, distributing -2 into $(b+5)$ should result in $-2b - 10$, but sometimes people incorrectly write $-2b + 10$. Another frequent mistake involves incorrectly performing the multiplication or addition/subtraction of the constants.
AI-generated content may contain errors. Please verify critical information