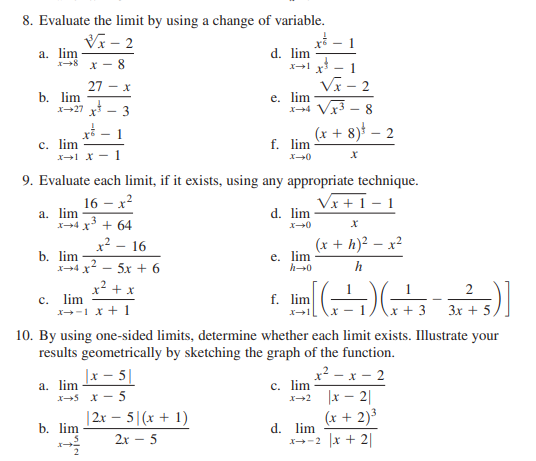
Evaluate the following limits: 1. lim (x->8) (x^(1/3) - 2) / (x - 8) 2. lim (x->27) (27 - x) / (x^(1/3) - 3) 3. lim (x->1) (x^(1/6) - 1) / (x - 1) 4. lim (x->1) (x^(1/6) - 1) / (x^... Evaluate the following limits: 1. lim (x->8) (x^(1/3) - 2) / (x - 8) 2. lim (x->27) (27 - x) / (x^(1/3) - 3) 3. lim (x->1) (x^(1/6) - 1) / (x - 1) 4. lim (x->1) (x^(1/6) - 1) / (x^(1/3) - 1) 5. lim (x->4) (sqrt(x) - 2) / (sqrt(x^3) - 8) 6. lim (x->0) ((x + 8)^(1/3) - 2) / x 7. lim (x->4) (16 - x^2) / (x^3 + 64) 8. lim (x->4) (x^2 - 16) / (x^2 - 5x + 6) 9. lim (x->-1) (x^2 + x) / (x + 1) 10. lim (x->0) (sqrt(x + 1) - 1) / x 11. lim (h->0) ((x + h)^2 - x^2) / h 12. lim (x->1) (1/(x - 1)) * (1/(x + 3) - 2/(3x + 5)) 13. lim (x->5) |x - 5| / (x - 5) 14. lim (x->5/2) |2x - 5| * (x + 1) / (2x - 5) 15. lim (x->2) (x^2 - x - 2) / |x - 2| 16. lim (x->-2) (x + 2)^3 / |x + 2| Read more

Steps to Solve

Here's a breakdown of how to solve each limit problem:

8a. $\lim_{x \to 8} \frac{\sqrt[3]{x} - 2}{x - 8}$

1. Change of variable Let $u = \sqrt[3]{x}$. Then $x = u^3$. As $x \to 8$, $u \to \sqrt[3]{8} = 2$. So we have: $$ \lim_{u \to 2} \frac{u - 2}{u^3 - 8} $$

2. Factor the denominator $u^3 - 8 = (u-2)(u^2 + 2u + 4)$. Thus,

$$ \lim_{u \to 2} \frac{u - 2}{(u - 2)(u^2 + 2u + 4)} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 2} \frac{1}{u^2 + 2u + 4} = \frac{1}{2^2 + 2(2) + 4} = \frac{1}{4 + 4 + 4} = \frac{1}{12} $$

8b. $\lim_{x \to 27} \frac{27 - x}{x^{\frac{1}{3}} - 3}$

1. Change of variable Let $u = x^{\frac{1}{3}}$. Then $x = u^3$. As $x \to 27$, $u \to \sqrt[3]{27} = 3$. So we have: $$ \lim_{u \to 3} \frac{27 - u^3}{u - 3} $$

2. Factor the numerator $27 - u^3 = (3 - u)(9 + 3u + u^2)$. Thus,

$$ \lim_{u \to 3} \frac{(3 - u)(9 + 3u + u^2)}{u - 3} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 3} \frac{-(u - 3)(9 + 3u + u^2)}{u - 3} = \lim_{u \to 3} -(9 + 3u + u^2) = -(9 + 3(3) + 3^2) = -(9 + 9 + 9) = -27 $$

8c. $\lim_{x \to 1} \frac{x^{\frac{1}{6}} - 1}{x - 1}$

1. Change of variable Let $u = x^{\frac{1}{6}}$. Then $x = u^6$. As $x \to 1$, $u \to \sqrt[6]{1} = 1$. $$ \lim_{u \to 1} \frac{u - 1}{u^6 - 1} $$

2. Factor the denominator $u^6 - 1 = (u - 1)(u^5 + u^4 + u^3 + u^2 + u + 1)$. Thus, $$ \lim_{u \to 1} \frac{u - 1}{(u - 1)(u^5 + u^4 + u^3 + u^2 + u + 1)} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 1} \frac{1}{u^5 + u^4 + u^3 + u^2 + u + 1} = \frac{1}{1 + 1 + 1 + 1 + 1 + 1} = \frac{1}{6} $$

8d. $\lim_{x \to 1} \frac{x^{\frac{1}{6}} - 1}{x^{\frac{1}{3}} - 1}$

1. Change of variable Let $u = x^{\frac{1}{6}}$. Then $x^{\frac{1}{3}} = u^2$.As $x \to 1$, $u \to \sqrt[6]{1} = 1$. $$ \lim_{u \to 1} \frac{u - 1}{u^2 - 1} $$

2. Factor the denominator $u^2 - 1 = (u - 1)(u + 1)$. Thus, $$ \lim_{u \to 1} \frac{u - 1}{(u - 1)(u + 1)} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 1} \frac{1}{u + 1} = \frac{1}{1 + 1} = \frac{1}{2} $$

8e. $\lim_{x \to 4} \frac{\sqrt{x} - 2}{\sqrt{x^3} - 8}$

1. Change of variable Let $u = \sqrt{x}$. Then $x = u^2$. As $x \to 4$, $u \to \sqrt{4} = 2$. Also $\sqrt{x^3} = (\sqrt{x})^3 = u^3$. So we have: $$ \lim_{u \to 2} \frac{u - 2}{u^3 - 8} $$

2. Factor the denominator $u^3 - 8 = (u - 2)(u^2 + 2u + 4)$. Then, $$ \lim_{u \to 2} \frac{u - 2}{(u - 2)(u^2 + 2u + 4)} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 2} \frac{1}{u^2 + 2u + 4} = \frac{1}{2^2 + 2(2) + 4} = \frac{1}{4 + 4 + 4} = \frac{1}{12} $$

8f. $\lim_{x \to 0} \frac{(x + 8)^{\frac{1}{3}} - 2}{x}$

1. Change of variable Let $u = (x + 8)^{\frac{1}{3}}$. Then $x = u^3 - 8$. As $x \to 0$, $u \to \sqrt[3]{0 + 8} = 2$. $$ \lim_{u \to 2} \frac{u - 2}{u^3 - 8} $$

2. Factor the denominator $u^3 - 8 = (u - 2)(u^2 + 2u + 4)$. Then, $$ \lim_{u \to 2} \frac{u - 2}{(u - 2)(u^2 + 2u + 4)} $$

3. Simplify and evaluate the limit $$ \lim_{u \to 2} \frac{1}{u^2 + 2u + 4} = \frac{1}{2^2 + 2(2) + 4} = \frac{1}{4 + 4 + 4} = \frac{1}{12} $$

9a. $\lim_{x \to 4} \frac{16 - x^2}{x^3 + 64}$

1. Factor numerator and denominator $16 - x^2 = (4 - x)(4 + x)$ $x^3 + 64 = (x + 4)(x^2 - 4x + 16)$. Thus, $$ \lim_{x \to 4} \frac{(4 - x)(4 + x)}{(x + 4)(x^2 - 4x + 16)} = \lim_{x \to 4} \frac{(4 - x)}{x^2 - 4x + 16} $$

2. Evaluate the limit $$ \frac{4 - 4}{4^2 - 4(4) + 16} = \frac{0}{16 - 16 + 16} = \frac{0}{16} = 0 $$

9b. $\lim_{x \to 4} \frac{x^2 - 16}{x^2 - 5x + 6}$

1. Factor numerator and denominator $x^2 - 16 = (x - 4)(x + 4)$ $x^2 - 5x + 6 = (x - 2)(x - 3)$. $$ \lim_{x \to 4} \frac{(x - 4)(x + 4)}{(x - 2)(x - 3)} $$

2. Evaluate the limit $$ \frac{(4 - 4)(4 + 4)}{(4 - 2)(4 - 3)} = \frac{0 \cdot 8}{2 \cdot 1} = \frac{0}{2} = 0 $$

9c. $\lim_{x \to -1} \frac{x^2 + x}{x + 1}$

1. Factor the numerator $x^2 + x = x(x + 1)$. Thus, $$ \lim_{x \to -1} \frac{x(x + 1)}{x + 1} $$

2. Simplify and evaluate the limit

$$ \lim_{x \to -1} x = -1 $$

9d. $\lim_{x \to 0} \frac{\sqrt{x + 1} - 1}{x}$

1. Rationalize the numerator Multiply by $\frac{\sqrt{x + 1} + 1}{\sqrt{x + 1} + 1}$: $$ \lim_{x \to 0} \frac{(\sqrt{x + 1} - 1)(\sqrt{x + 1} + 1)}{x(\sqrt{x + 1} + 1)} = \lim_{x \to 0} \frac{(x + 1) - 1}{x(\sqrt{x + 1} + 1)} $$

2. Simplify and evaluate the limit $$ \lim_{x \to 0} \frac{x}{x(\sqrt{x + 1} + 1)} = \lim_{x \to 0} \frac{1}{\sqrt{x + 1} + 1} = \frac{1}{\sqrt{0 + 1} + 1} = \frac{1}{1 + 1} = \frac{1}{2} $$

9e. $\lim_{h \to 0} \frac{(x + h)^2 - x^2}{h}$

1. Expand the numerator $(x + h)^2 - x^2 = x^2 + 2xh + h^2 - x^2 = 2xh + h^2$. $$ \lim_{h \to 0} \frac{2xh + h^2}{h} $$

2. Simplify and evaluate the limit $$ \lim_{h \to 0} \frac{h(2x + h)}{h} = \lim_{h \to 0} (2x + h) = 2x + 0 = 2x $$

9f. $\lim_{x \to 1} [(\frac{1}{x - 1}) * (\frac{1}{x + 3} - \frac{2}{3x + 5})]$

1. Simplify the expression inside the brackets: Find a common denominator for the fractions:

$$ \frac{1}{x + 3} - \frac{2}{3x + 5} = \frac{(3x + 5) - 2(x + 3)}{(x + 3)(3x + 5)} = \frac{3x + 5 - 2x - 6}{(x + 3)(3x + 5)} = \frac{x - 1}{(x + 3)(3x + 5)} $$ Thus, $$ \lim_{x \to 1} \frac{1}{x - 1} \cdot \frac{x - 1}{(x + 3)(3x + 5)} $$

2. Simplify and evaluate the limit $$ \lim_{x \to 1} \frac{1}{(x + 3)(3x + 5)} = \frac{1}{(1 + 3)(3(1) + 5)} = \frac{1}{(4)(8)} = \frac{1}{32} $$

10a. $\lim_{x \to 5} \frac{|x - 5|}{x - 5}$

1. Evaluate the left-hand limit $$ \lim_{x \to 5^-} \frac{|x - 5|}{x - 5} = \lim_{x \to 5^-} \frac{-(x - 5)}{x - 5} = -1 $$

2. Evaluate the right-hand limit $$ \lim_{x \to 5^+} \frac{|x - 5|}{x - 5} = \lim_{x \to 5^+} \frac{x - 5}{x - 5} = 1 $$

3. Compare the one-sided limits Since the left-hand limit and right-hand limit are not equal, the limit does not exist.

10b. $\lim_{x \to \frac{5}{2}} \frac{|2x - 5|(x + 1)}{2x - 5}$

1. Evaluate the left-hand limit When $x \to \frac{5}{2}^-$, $2x - 5 < 0$, so $|2x - 5| = -(2x - 5)$. $$ \lim_{x \to \frac{5}{2}^-} \frac{|2x - 5|(x + 1)}{2x - 5} = \lim_{x \to \frac{5}{2}^-} \frac{-(2x - 5)(x + 1)}{2x - 5} = \lim_{x \to \frac{5}{2}^-} -(x + 1) = -(\frac{5}{2} + 1) = -\frac{7}{2} $$

2. Evaluate the right-hand limit When $x \to \frac{5}{2}^+$, $2x - 5 > 0$, so $|2x - 5| = (2x - 5)$. $$ \lim_{x \to \frac{5}{2}^+} \frac{|2x - 5|(x + 1)}{2x - 5} = \lim_{x \to \frac{5}{2}^+} \frac{(2x - 5)(x + 1)}{2x - 5} = \lim_{x \to \frac{5}{2}^+} (x + 1) = \frac{5}{2} + 1 = \frac{7}{2} $$

3. Compare the one-sided limits Since the left-hand limit and right-hand limit are not equal, the limit does not exist.

10c. $\lim_{x \to 2} \frac{x^2 - x - 2}{|x - 2|}$

1. Factor the numerator $x^2 - x - 2 = (x - 2)(x + 1)$. Thus, $$ \lim_{x \to 2} \frac{(x - 2)(x + 1)}{|x - 2|} $$

2. Evaluate the left-hand limit When $x \to 2^-$, $x - 2 < 0$, so $|x - 2| = -(x - 2)$. $$ \lim_{x \to 2^-} \frac{(x - 2)(x + 1)}{-(x - 2)} = \lim_{x \to 2^-} -(x + 1) = -(2 + 1) = -3 $$

3. Evaluate the right-hand limit When $x \to 2^+$, $x - 2 > 0$, so $|x - 2| = (x - 2)$. $$ \lim_{x \to 2^+} \frac{(x - 2)(x + 1)}{x - 2} = \lim_{x \to 2^+} (x + 1) = 2 + 1 = 3 $$

4. Compare the one-sided limits Since the left-hand limit and right-hand limit are not equal, the limit does not exist.

10d. $\lim_{x \to -2} \frac{(x + 2)^3}{|x + 2|}$

1. Evaluate the left-hand limit When $x \to -2^-$, $x + 2 < 0$, so $|x + 2| = -(x + 2)$. $$ \lim_{x \to -2^-} \frac{(x + 2)^3}{-(x + 2)} = \lim_{x \to -2^-} -(x + 2)^2 = -( -2 + 2)^2 = 0 $$

2. Evaluate the right-hand limit When $x \to -2^+$, $x + 2 > 0$, so $|x + 2| = (x + 2)$. $$ \lim_{x \to -2^+} \frac{(x + 2)^3}{(x + 2)} = \lim_{x \to -2^+} (x + 2)^2 = (-2 + 2)^2 = 0 $$

3. Compare the one-sided limits

Since the left-hand limit and right-hand limit are equal to 0, the limit exists and is equal to 0.