Evaluate: (0.18905^4) / ((0.1314^4)/29 + (0.02534^4)/59)
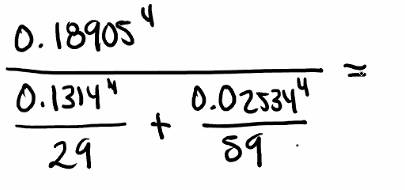
Understand the Problem
The question is asking to evaluate the following mathematical expression: (0.18905^4) / ((0.1314^4)/29 + (0.02534^4)/59). We need to simplify this calculation to arrive at an answer.
Answer
$123.11$
Answer for screen readers
$123.11$
Steps to Solve
- Calculate $0.18905^4$
Calculate the value of $0.18905$ raised to the power of $4$.
$0.18905^4 \approx 0.0012757$
- Calculate $0.1314^4$
Calculate the value of $0.1314$ raised to the power of $4$.
$0.1314^4 \approx 0.0003003$
- Calculate $\frac{0.1314^4}{29}$
Divide the result from step 2 by $29$.
$\frac{0.0003003}{29} \approx 0.000010355$
- Calculate $0.02534^4$
Calculate the value of $0.02534$ raised to the power of $4$.
$0.02534^4 \approx 0.000000412$
- Calculate $\frac{0.02534^4}{59}$
Divide the result from step 4 by $59$.
$\frac{0.000000412}{59} \approx 0.00000000698$
- Calculate $\frac{0.1314^4}{29} + \frac{0.02534^4}{59}$
Add the results from step 3 and step 5.
$0.000010355 + 0.00000000698 \approx 0.000010362$
- Calculate $\frac{0.18905^4}{\frac{0.1314^4}{29} + \frac{0.02534^4}{59}}$
Divide the result from step 1 by the result from step 6.
$\frac{0.0012757}{0.000010362} \approx 123.11$
$123.11$
More Information
The final answer is approximately $123.11$.
Tips
- Rounding too early can lead to inaccurate results. It is best to keep as many decimal places as possible until the final step.
- Forgetting the order of operations (PEMDAS/BODMAS) can lead to incorrect calculations.
AI-generated content may contain errors. Please verify critical information