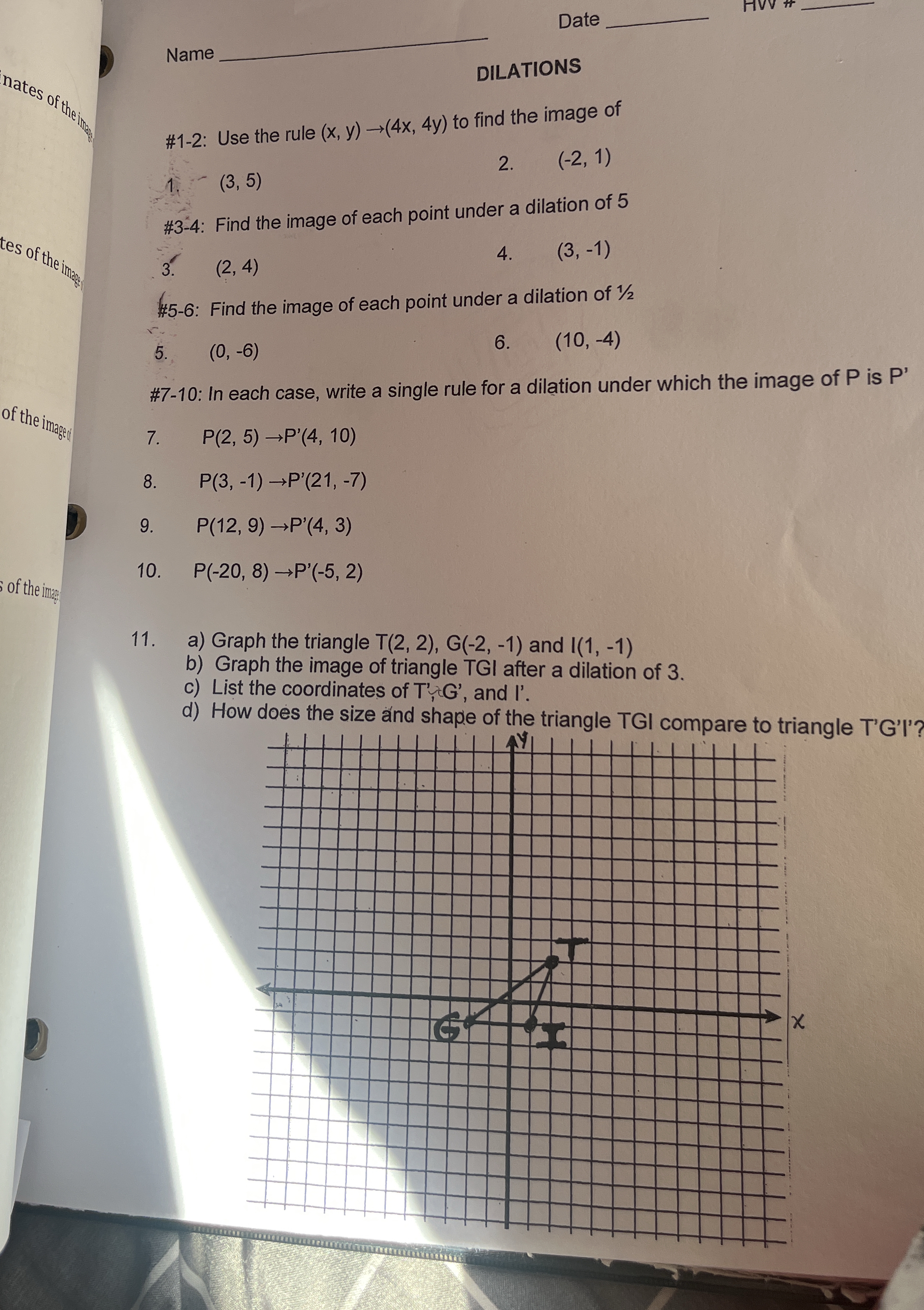
Dilation homework assignment questions.
Understand the Problem
The image shows a homework assignment on dilations. The student needs to perform dilations on given points and triangles according to specified rules and factors. This involves applying the dilation rule (x, y) -> (kx, ky), where k is the dilation factor, to find the new coordinates of the points and comparing the size and shape of the original and dilated triangles.
Answer
1. (12, 20) 2. (-8, 4) 3. (10, 20) 4. (15, -5) 5. (0, -3) 6. (5, -2) 7. (x, y) -> (2x, 2y) 8. (x, y) -> (7x, 7y) 9. (x, y) -> ($ \frac{1}{3} $x, $ \frac{1}{3} $y) 10. (x, y) -> ($ \frac{1}{4} $x, $ \frac{1}{4} $y) 11. c) T'(6, 6), G'(-6, -3), I'(3, -3) d) Larger size, same shape.
Answer for screen readers
- (12, 20)
- (-8, 4)
- (10, 20)
- (15, -5)
- (0, -3)
- (5, -2)
- (x, y) -> (2x, 2y)
- (x, y) -> (7x, 7y)
- (x, y) -> ($ \frac{1}{3} $x, $ \frac{1}{3} $y)
- (x, y) -> ($ \frac{1}{4} $x, $ \frac{1}{4} $y)
c) T'(6, 6), G'(-6, -3), I'(3, -3) d) The size of triangle T'G'I' is larger, but the shape is the same.
Steps to Solve
- Apply the dilation rule (x, y) -> (4x, 4y) to point (3, 5)
Multiply each coordinate of the point (3, 5) by 4:
$ (4 \cdot 3, 4 \cdot 5) = (12, 20) $
- Apply the dilation rule (x, y) -> (4x, 4y) to point (-2, 1)
Multiply each coordinate of the point (-2, 1) by 4:
$ (4 \cdot -2, 4 \cdot 1) = (-8, 4) $
- Apply a dilation of 5 to point (2, 4)
Multiply each coordinate of the point (2, 4) by 5:
$ (5 \cdot 2, 5 \cdot 4) = (10, 20) $
- Apply a dilation of 5 to point (3, -1)
Multiply each coordinate of the point (3, -1) by 5:
$ (5 \cdot 3, 5 \cdot -1) = (15, -5) $
- Apply a dilation of 1/2 to point (0, -6)
Multiply each coordinate of the point (0, -6) by 1/2:
$ (\frac{1}{2} \cdot 0, \frac{1}{2} \cdot -6) = (0, -3) $
- Apply a dilation of 1/2 to point (10, -4)
Multiply each coordinate of the point (10, -4) by 1/2:
$ (\frac{1}{2} \cdot 10, \frac{1}{2} \cdot -4) = (5, -2) $
- Find the dilation rule for P(2, 5) -> P'(4, 10)
Divide the coordinates of P' by the coordinates of P: $ \frac{4}{2} = 2 $ and $ \frac{10}{5} = 2 $ The dilation rule is (x, y) -> (2x, 2y).
- Find the dilation rule for P(3, -1) -> P'(21, -7)
Divide the coordinates of P' by the coordinates of P: $ \frac{21}{3} = 7 $ and $ \frac{-7}{-1} = 7 $ The dilation rule is (x, y) -> (7x, 7y).
- Find the dilation rule for P(12, 9) -> P'(4, 3)
Divide the coordinates of P' by the coordinates of P: $ \frac{4}{12} = \frac{1}{3} $ and $ \frac{3}{9} = \frac{1}{3} $ The dilation rule is (x, y) -> ($ \frac{1}{3} $x, $ \frac{1}{3} $y).
- Find the dilation rule for P(-20, 8) -> P'(-5, 2)
Divide the coordinates of P' by the coordinates of P: $ \frac{-5}{-20} = \frac{1}{4} $ and $ \frac{2}{8} = \frac{1}{4} $ The dilation rule is (x, y) -> ($ \frac{1}{4} $x, $ \frac{1}{4} $y).
- Graph triangle TGI and its dilation
a) Graph the triangle T(2, 2), G(-2, -1), and I(1, -1). (Refer to the image provided earlier which already contains the graph)
b) Graph the image of triangle TGI after a dilation of 3.
c) Calculate the new coordinates:
- T'(3 * 2, 3 * 2) = T'(6, 6)
- G'(3 * -2, 3 * -1) = G'(-6, -3)
- I'(3 * 1, 3 * -1) = I'(3, -3) d) Compare the size and shape: The size of triangle T'G'I' is larger than triangle TGI. The shape remains the same; they are similar triangles.
- (12, 20)
- (-8, 4)
- (10, 20)
- (15, -5)
- (0, -3)
- (5, -2)
- (x, y) -> (2x, 2y)
- (x, y) -> (7x, 7y)
- (x, y) -> ($ \frac{1}{3} $x, $ \frac{1}{3} $y)
- (x, y) -> ($ \frac{1}{4} $x, $ \frac{1}{4} $y)
c) T'(6, 6), G'(-6, -3), I'(3, -3) d) The size of triangle T'G'I' is larger, but the shape is the same.
More Information
Dilation is a transformation that produces an image that is the same shape as the original, but is a different size. A dilation stretches or shrinks the original figure. The dilation is described by its scale factor (or dilation factor) and the center of the dilation. The scale factor is the ratio of the side length of the image to the corresponding side length of the original figure.
Tips
- Forgetting to multiply both the x and y coordinates by the dilation factor.
- Incorrectly applying the dilation factor (e.g., multiplying instead of dividing when the dilation factor is a fraction for problems like #9 and #10).
- Making arithmetic errors when calculating the new coordinates.
AI-generated content may contain errors. Please verify critical information