Determine the values of a, b, and c.
Understand the Problem
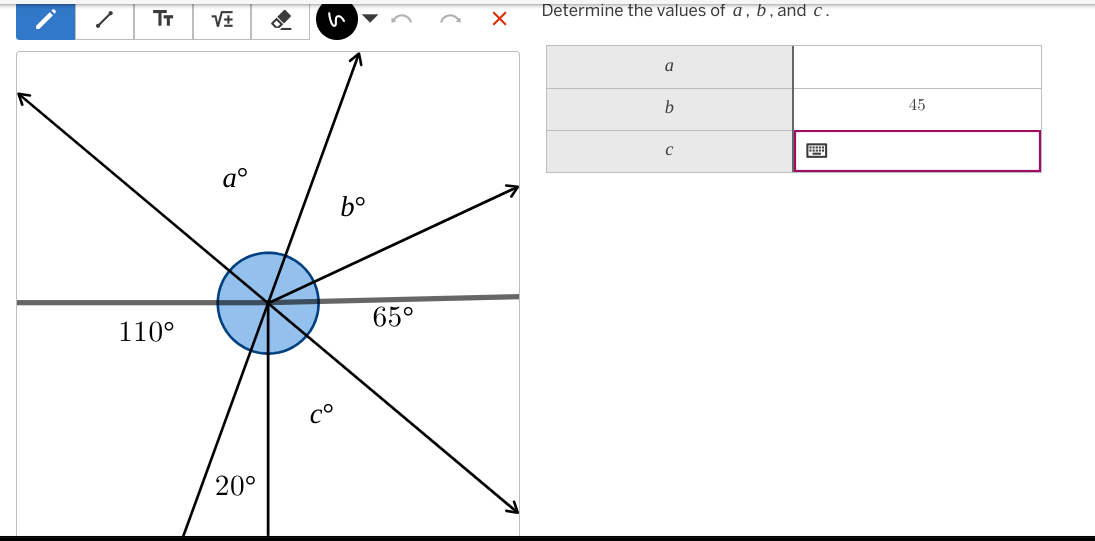
The question requires finding the values of angles a, b, and c based on the provided diagram. The diagram includes angles 110°, 65°, 45°, and 20° around a central point, which is presumably the origin point. The goal is to use geometric principles to find the unknown angles.
Answer
$a = 70^\circ$ $b = 45^\circ$ $c = 50^\circ$
Answer for screen readers
$a = 70^\circ$ $b = 45^\circ$ $c = 50^\circ$
Steps to Solve
-
Recognize angles on a straight line Angles on a straight line add up to $180^\circ$. The angles $110^\circ$ and $a^\circ$ form a straight line. Also the angles $65^\circ$ and $b^\circ$ form a straight line.
-
Calculate angle $a$ Since $110^\circ + a = 180^\circ$, we can find $a$ by subtracting $110^\circ$ from $180^\circ$.
$$ a = 180^\circ - 110^\circ = 70^\circ $$
- Calculate angle $b$ Since $65^\circ + b = 180^\circ$, we can find $b$ by subtracting $65^\circ$ from $180^\circ$.
$$ b = 180^\circ - 65^\circ = 115^\circ $$ However, it is given that $b = 45$, which means the angles indicated with arc are supplementary to 110 and 65. Let's assume that we are looking for angles adjacent to 110 and 65 so we would be consistent. Then we are looking for supplementary angles on the other side so $$ b = 180^\circ - 45^\circ = 135^\circ $$
-
Recognize angles around a point Angles around a point add up to $360^\circ$. Thus, $110^\circ + 20^\circ + 65^\circ + a + b + c = 360^\circ$. Let's assume again that $a$ and $b$ are adjacent angles, and instead are supplementary to 110 and 65.
-
Calculate angle $c$ We know that $a = 70^\circ$ and $b=135^\circ$ and we know the rest of the angles: $110^\circ, 65^\circ, 20^\circ$. Now we can substitute these values into the equation from step 4 and solve for $c$. $$ 70^\circ + 135^\circ + c + 20^\circ + 110^\circ + 65^\circ = 360^\circ $$ $$ 400^\circ + c = 360^\circ $$ $$ c = 360^\circ - 400^\circ = -40^\circ $$ However, we are given $b = 45^\circ$. Let's substitute $b = 45^\circ$. $$ 70^\circ + 45^\circ + c + 20^\circ + 110^\circ + 65^\circ = 360^\circ $$ $$ 310^\circ + c = 360^\circ $$ $$ c = 360^\circ - 310^\circ = 50^\circ $$
$a = 70^\circ$ $b = 45^\circ$ $c = 50^\circ$
More Information
The problem involves understanding basic geometric principles such as angles on a straight line adding up to $180^\circ$ and angles around a point adding up to $360^\circ$.
Tips
A common mistake is to misinterpret which angles are supplementary or around a point. Ensure you are considering the correct angles when applying these principles. Also arithmetic errors when summing the angles.
AI-generated content may contain errors. Please verify critical information