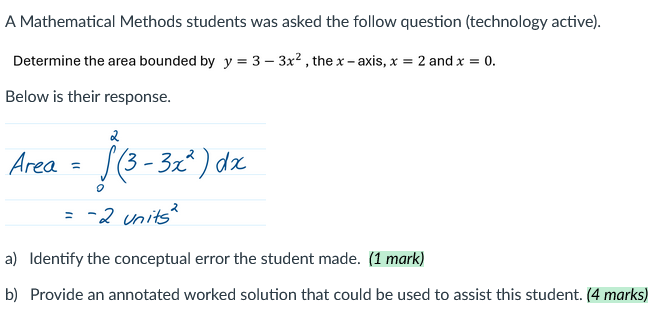
Determine the area bounded by y = 3 - 3x^2, the x-axis, x = 2 and x = 0. Identify the conceptual error the student made. Provide an annotated worked solution that could assist this... Determine the area bounded by y = 3 - 3x^2, the x-axis, x = 2 and x = 0. Identify the conceptual error the student made. Provide an annotated worked solution that could assist this student.
Understand the Problem
The question is asking to identify a conceptual error made by a student when calculating the area bounded by a curve and to provide a detailed worked solution to assist the student in understanding the correct approach.
Answer
The area bounded by the curve is $2 \text{ units}^2$.
Answer for screen readers
The area bounded by the curve is $2 \text{ units}^2$.
Steps to Solve
-
Identify the concept of area under a curve
The area bounded by a curve $y=f(x)$, the x-axis, and vertical lines $x=a$ and $x=b$ is found using definite integrals. -
Setting up the integral
The correct setup for the area bounded by the curve $y = 3 - 3x^2$ from $x = 0$ to $x = 2$ is: $$ \text{Area} = \int_{0}^{2} (3 - 3x^2) , dx $$ This signifies the area between the function and the x-axis. -
Calculate the integral
Now, we calculate the integral step by step:- Find the antiderivative: $$ \int (3 - 3x^2) , dx = 3x - x^3 + C $$
- Evaluate the definite integral: $$ \text{Area} = \left[3x - x^3\right]_{0}^{2} = (3(2) - (2)^3) - (3(0) - (0)^3) = 6 - 8 - 0 = -2 $$
-
Interpreting the result
The result of $-2$ indicates that the area calculated is below the x-axis. We interpret the area as the positive value: $$ \text{Area} = 2 \text{ units}^2 $$
The area bounded by the curve is $2 \text{ units}^2$.
More Information
The student made an error by directly reporting the integral's result, which was negative, without considering that area is always positive. The correct interpretation of area in this context is its absolute value.
Tips
- Confusing negative area: Students often forget that area should be expressed as a positive value, even if the integral yields a negative result.
- Misapplication of limits: Sometimes integrating from the wrong limits or flipping them can lead to a misunderstanding of the area calculated.
AI-generated content may contain errors. Please verify critical information