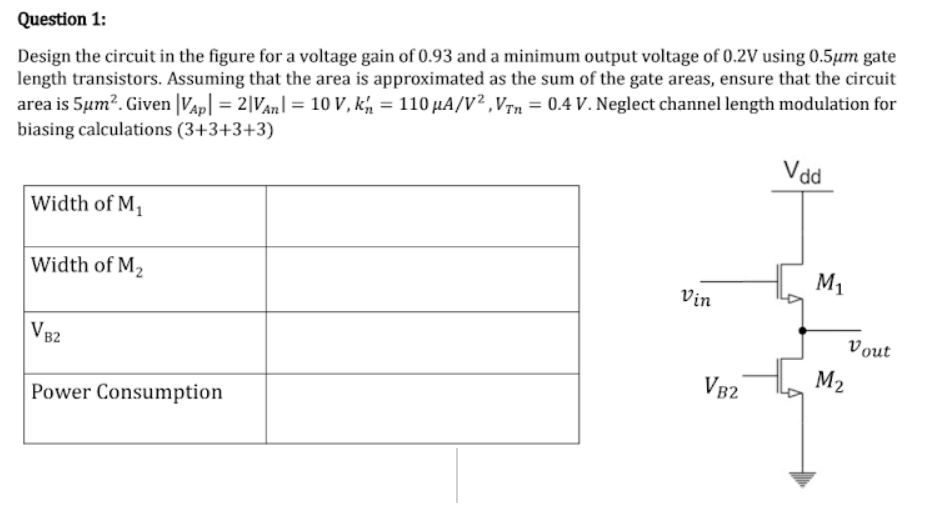
Design the circuit in the figure for a voltage gain of 0.93 and a minimum output voltage of 0.2V using 0.5µm gate length transistors. Assuming that the area is approximated as the... Design the circuit in the figure for a voltage gain of 0.93 and a minimum output voltage of 0.2V using 0.5µm gate length transistors. Assuming that the area is approximated as the sum of the gate areas, ensure that the circuit area is 5µm². Given |VAp| = 2|VAn| = 10 V, k'n = 110 μA/V², VTn = 0.4 V. Neglect channel length modulation for biasing calculations. Find the width of M1, width of M2, VB2, and the power consumption. Read more

Steps to Solve

1. Determine the required overdrive voltage for M2 -The minimum output voltage is given as $0.2V$. This implies that $V_{DS2} = 0.2V$ and $V_{DS2} = V_{GS2} - V_{Tn}$. We can find $V_{GS2}$ since $V_{GS2} = V_{B2}$.

   • Therefore, $V_{B2} = V_{DS2} + V_{Tn} = 0.2V + 0.4V = 0.6V$.

2. Find the drain current $I_D$ -The voltage gain is $0.93$. The voltage gain equation is given by: $A_v = \frac{g_{m1}}{g_{m1} + g_{m2}}$ -Where $g_{m1} = \sqrt{2k'p(\frac{W_1}{L})I_D}$ and $g{m2} = \sqrt{2k'_n(\frac{W_2}{L})I_D}$ -Substitute these into the gain equation and solve: $0.93 = \frac{\sqrt{2k'_p(\frac{W_1}{L})I_D}}{\sqrt{2k'_p(\frac{W_1}{L})I_D} + \sqrt{2k'_n(\frac{W_2}{L})I_D}} = \frac{\sqrt{k'_p(\frac{W_1}{L})}}{\sqrt{k'_p(\frac{W_1}{L})} + \sqrt{k'_n(\frac{W_2}{L})}}$ -Square both sides: $0.8649 = \frac{k'_p(\frac{W_1}{L})}{k'_p(\frac{W_1}{L}) + k'_n(\frac{W_2}{L})}$ -Rearrange to isolate $\frac{W_1}{W_2}$: $0.8649[k'_p(\frac{W_1}{L}) + k'_n(\frac{W_2}{L})] = k'_p(\frac{W_1}{L})$ $0.8649 k'_p(\frac{W_1}{L}) + 0.8649 k'_n(\frac{W_2}{L}) = k'_p(\frac{W_1}{L})$ $0.8649 k'_n(\frac{W_2}{L}) = k'_p(\frac{W_1}{L}) - 0.8649 k'_p(\frac{W_1}{L})$ $0.8649 k'_n(\frac{W_2}{L}) = 0.1351 k'_p(\frac{W_1}{L})$ $\frac{W_1}{W_2} = \frac{0.8649}{0.1351} \frac{k'_n}{k'_p} = 6.402 \frac{k'n}{k'p}$ -Since $|V{Ap}|=2|V{An}|$, $k'_p = \frac{k'_n}{2}$ $\frac{W_1}{W_2} = 6.402 \times 2 = 12.804$ $W_1=12.804W_2$

3. Determine Widths W1 and W2 from the area constraint -The area constraint is given by $A = (W_1 + W_2)L = 5 \mu m^2$, and $L = 0.5 \mu m$. -Therefore, $W_1 + W_2 = \frac{5 \mu m^2}{0.5 \mu m}= 10 \mu m$. -Substitute the relationship between W1 and W2 to find the value of W2: $12.804W_2 + W_2 = 10 \mu m$ $13.804W_2 = 10 \mu m$ $W_2 = \frac{10}{13.804} = 0.724 \mu m$ -Now find W1: $W_1 = 12.804 \times 0.724 = 9.273 \mu m$

4. Determine $I_D$ -$I_D = \frac{1}{2}k'n (\frac{W_2}{L})(V{GS2} - V_{Tn})^2$ $I_D = \frac{1}{2} \times 110 \mu A/V^2 \times (\frac{0.724 \mu m}{0.5 \mu m})(0.6V - 0.4V)^2 = 3.1868 \mu A$

5. Determine $V_{DD}$ -$V_{out} = V_{DD} - |V_{GS1}|$ -$V_{GS1} = |(-V_{SG1})| = |-(V_{DD} - V_{out})| = |V_{TP}| + \sqrt{\frac{2I_D}{k'p(W_1/L)}}$ -Substitute for $V{out}=0.2V$: $V_{DD} - 0.2 = 0.4 + \sqrt{\frac{2(3.1868 \mu A)}{\frac{110}{2} \mu A/V^2 (\frac{9.273 \mu m}{0.5 \mu m})}}$ $V_{DD} - 0.2 = 0.4 + \sqrt{\frac{6.3736}{1019.915}} = 0.4 + 0.079$ $V_{DD} = 0.679 V$ -$V_{DD} \approx 0.68 V$

6. Calculate Power Consumption

   -$P = I_D \times V_{DD} = 3.1868 \mu A \times 0.679 V = 2.16 \mu W$