Create very difficult numerical and application based questions from the image given.

Steps to Solve

1. Understanding the Universal Law of Gravitation

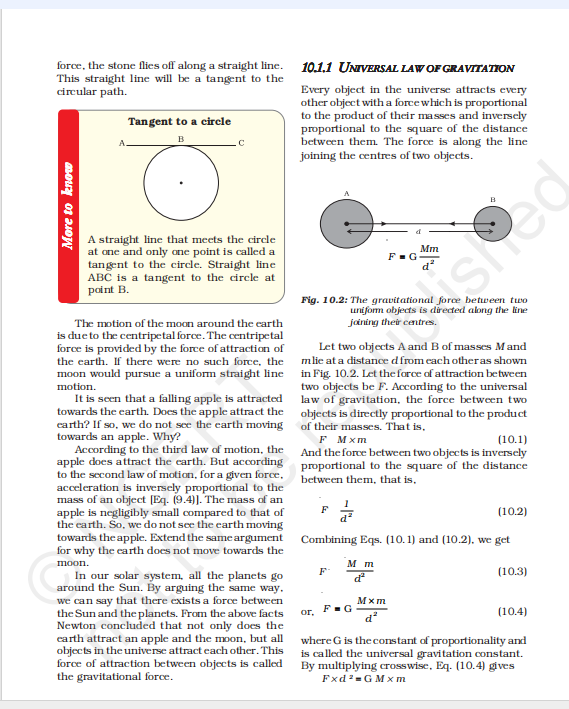
The Universal Law of Gravitation states that every two objects attract each other with a force ($F$) given by the equation: $$ F = \frac{G \cdot M \cdot m}{d^2} $$ where $G$ is the gravitational constant, $M$ and $m$ are the masses of the two objects, and $d$ is the distance between their centers.

2. Setting Up a Problem

Let’s create a problem using the universal law. Consider two masses:

• Mass $M = 5 , \text{kg}$
• Mass $m = 10 , \text{kg}$

They are located $d = 2 , \text{m}$ apart.

3. Calculating the Gravitational Force

Using the formula, calculate the gravitational force between these two masses. The gravitational constant $G$ is approximately: $$ G \approx 6.674 \times 10^{-11} , \text{N m}^2/\text{kg}^2 $$

Plugging the values into the equation: $$ F = \frac{(6.674 \times 10^{-11}) \cdot 5 \cdot 10}{(2^2)} $$

4. Performing the Calculation

First, calculate the denominator: $$ 2^2 = 4 $$

Then, substitute back into the equation: $$ F = \frac{(6.674 \times 10^{-11}) \cdot 5 \cdot 10}{4} $$

5. Final Calculation

Now, compute the product: $$ F = \frac{(6.674 \times 10^{-11}) \cdot 50}{4} $$ $$ F = \frac{3.337 \times 10^{-9}}{4} $$ Finally, $$ F = 8.3425 \times 10^{-10} , \text{N} $$