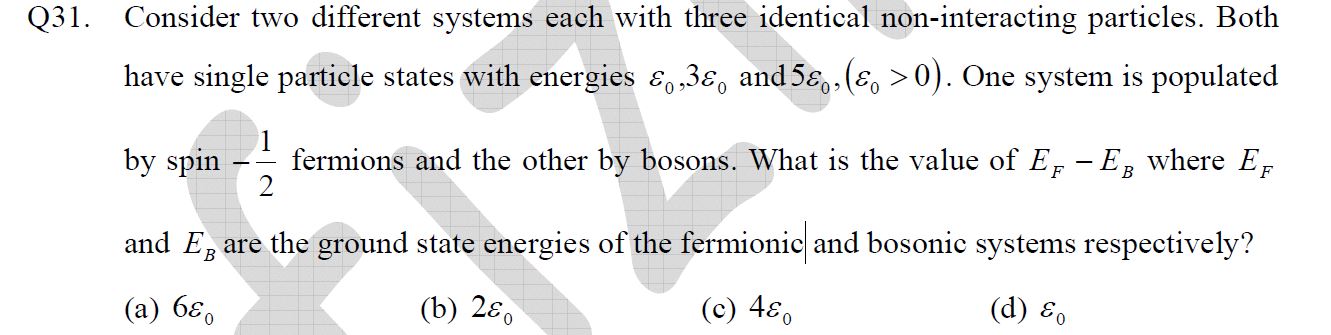
Consider two different systems each with three identical non-interacting particles. Both have single particle states with energies ε0, 3ε0 and 5ε0 (ε0 > 0). One system is populated... Consider two different systems each with three identical non-interacting particles. Both have single particle states with energies ε0, 3ε0 and 5ε0 (ε0 > 0). One system is populated by spin -1/2 fermions and the other by bosons. What is the value of EF - EB where EF and EB are the ground state energies of the fermionic and bosonic systems respectively? Read more

Steps to Solve

1. Identify Single Particle Energy States The given energy states for particles are $\epsilon_0$, $3\epsilon_0$, and $5\epsilon_0$.

2. Calculate Ground State Energy for Fermions ($E_F$) For a system of fermions, the Pauli exclusion principle states that no two identical fermions can occupy the same quantum state. Thus, the lowest energy states will be filled first. The three fermions will occupy the states:

• First fermion: $\epsilon_0$
• Second fermion: $3\epsilon_0$
• Third fermion: $5\epsilon_0$

So, the total ground state energy for fermions is: $$ E_F = \epsilon_0 + 3\epsilon_0 + 5\epsilon_0 = 9\epsilon_0 $$

3. Calculate Ground State Energy for Bosons ($E_B$) In contrast, bosons can occupy the same quantum state. Therefore, to achieve the lowest energy configuration, they can all occupy the lowest energy state:

• All three bosons occupy the state $\epsilon_0$.

Thus, the total ground state energy for bosons is: $$ E_B = \epsilon_0 + \epsilon_0 + \epsilon_0 = 3\epsilon_0 $$

4. Calculate the Difference in Energy ($E_F - E_B$) Now, we find the difference between the ground state energies of fermions and bosons: $$ E_F - E_B = 9\epsilon_0 - 3\epsilon_0 = 6\epsilon_0 $$