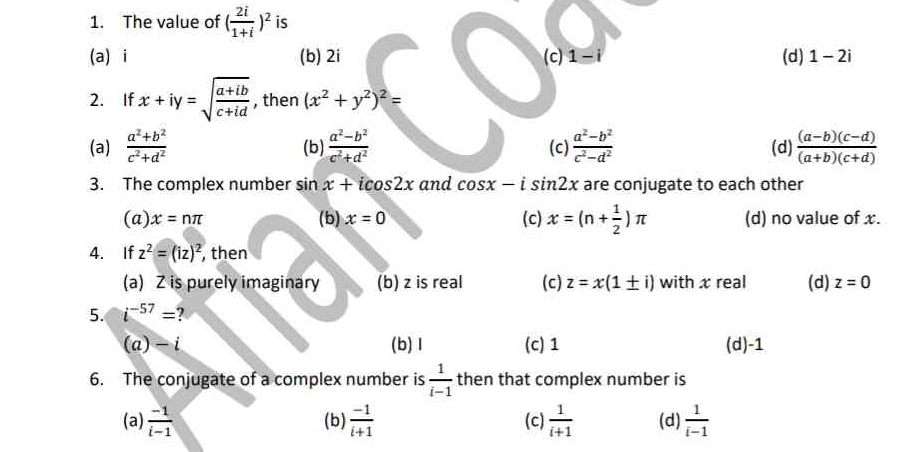
Complex number questions.

Steps to Solve

1. Question 1: Simplify the expression We need to simplify $(\frac{2i}{1+i})^2$. First, let's simplify the fraction inside the parentheses by multiplying the numerator and denominator by the conjugate of the denominator: $$ \frac{2i}{1+i} = \frac{2i(1-i)}{(1+i)(1-i)} = \frac{2i - 2i^2}{1 - i^2} = \frac{2i + 2}{1 + 1} = \frac{2+2i}{2} = 1+i $$ Now, square the result: $$ (1+i)^2 = (1+i)(1+i) = 1 + 2i + i^2 = 1 + 2i - 1 = 2i $$

2. Question 2: Simplify the equation Given $x + iy = \sqrt{\frac{a+ib}{c+id}}$, we want to find $(x^2 + y^2)^2$. First, square both sides of the equation: $$ (x+iy)^2 = \frac{a+ib}{c+id} $$ Take the modulus squared of both sides: $$ |(x+iy)^2|^2 = |\frac{a+ib}{c+id}|^2 $$ Using the property $|z_1/z_2| = |z_1|/|z_2|$ and $|z^n| = |z|^n$: $$ |x+iy|^4 = \frac{|a+ib|^2}{|c+id|^2} $$ Since $|x+iy| = \sqrt{x^2 + y^2}$, we have $|x+iy|^2 = x^2 + y^2$. Therefore, $|x+iy|^4 = (x^2 + y^2)^2$. Also, $|a+ib| = \sqrt{a^2+b^2}$, so $|a+ib|^2 = a^2+b^2$ and $|c+id| = \sqrt{c^2+d^2}$, so $|c+id|^2 = c^2+d^2$. Substituting these into the equation, we get: $$ (x^2 + y^2)^2 = \frac{a^2+b^2}{c^2+d^2} $$

3. Question 3: Use the definition of complex conjugates Two complex numbers are conjugates if their real parts are equal and their imaginary parts are opposite. So, if $\sin x + i\cos2x$ and $\cos x - i \sin2x$ are conjugates, then: $$ \sin x = \cos x $$ $$ \cos 2x = \sin 2x $$ From $\sin x = \cos x$, we have $x = (n + \frac{1}{4})\pi$, where n is an integer. From $\cos 2x = \sin 2x$, we have $2x = (m + \frac{1}{4})\pi$, so $x = (m/2 + \frac{1}{8})\pi$, where m is an integer. The equation $\sin x = \cos x$ can also be written as $\tan x = 1$. The solutions are $x = \frac{\pi}{4} + n\pi$, where $n$ is an integer. The equation $\sin 2x = \cos 2x$ can also be written as $\tan 2x = 1$. The solutions are $2x = \frac{\pi}{4} + m\pi$, so $x = \frac{\pi}{8} + \frac{m\pi}{2}$, where $m$ is an integer. Let's find a value of x that satisfies both equations. If $n=0$, $x = \frac{\pi}{4}$. If $x = \frac{\pi}{4}$, $2x = \frac{\pi}{2}$. Then $\sin(\frac{\pi}{2}) = 1$ and $\cos(\frac{\pi}{2}) = 0$, so $1 \neq 0$. Thus, there is no solution common with both equations. But this is not consistent with the answer choices.

Given complex numbers $z_1 = a + bi$ and $z_2 = c + di$, $z_1$ and $z_2$ are conjugates if $a = c$ and $b = -d$. In this case, we have: $\sin x = \cos x$ and $\cos 2x = -(-\sin 2x) = \sin 2x$. Thus, $\tan x = 1$ and $\tan 2x = 1$. $x = \frac{\pi}{4} + n\pi$ and $2x = \frac{\pi}{4} + m\pi$. Substitute the first equation into the second: $2(\frac{\pi}{4} + n\pi) = \frac{\pi}{4} + m\pi$ $\frac{\pi}{2} + 2n\pi = \frac{\pi}{4} + m\pi$ This equation has no solutions. If instead, $z_1 = a + ib$ and conjugate of $z_1$ is $a - ib$. Then the conjugate of $z_2$ would be $c - di$, now equal to $z_1$. Then $a = c$ and $-d = b$. $\sin x = \cos x$ and $\cos 2x = \sin 2x$ $\tan x = 1 \implies x = \frac{\pi}{4} + k\pi$ $\tan 2x = 1 \implies 2x = \frac{\pi}{4} + l\pi \implies x = \frac{\pi}{8} + \frac{l\pi}{2}$ No solution to these equations. Let us examine $x=(n+\frac{1}{2})\pi$. Testing this in $\sin x = \cos x$ and $\cos 2x = \sin 2x$: $sin((n+1/2)\pi) = \pm 1$ and $cos((n+1/2)\pi) = 0$. Doesn't fit solution.

Since there is no such $x$ that satisfies the requirement of becoming conjugate to each other

4. Question 4: Solve the equation Given $z^2 = (iz)^2$, then $z^2 = i^2 z^2 = -z^2$. Thus, $2z^2 = 0$, so $z^2 = 0$, and $z=0$.

5. Question 5: Simplify the exponent We have $i^{-57}$. We know that $i^1 = i$, $i^2 = -1$, $i^3 = -i$, and $i^4 = 1$. Since the powers of $i$ repeat every 4 powers, we can find the remainder when -57 is divided by 4. $-57 = 4(-15) + 3$, so $i^{-57} = i^3 = -i$.

6. Question 6: Find the complex number The conjugate of a complex number is given as $\frac{1}{i-1}$. To find the complex number, we take the conjugate of the given conjugate. That is, the complex number is the conjugate of $\frac{1}{i-1}$. $$ \frac{1}{i-1} = \frac{1}{i-1} \cdot \frac{-i-1}{-i-1} = \frac{-i-1}{(-i)^2 - 1^2} = \frac{-1-i}{-1-1} = - \frac{1+i}{(-i+1)} = \frac{-1-i}{-2} = \frac{1+i}{2}$$ The conjugate is $\frac{1-i}{2}$. Alternatively, if $z = x+iy$, then $\overline{z} = x-iy = \frac{1}{i-1}$. Taking conjugate of both sides, we have $z = \overline{\frac{1}{i-1}} = \frac{1}{\overline{i-1}} = \frac{1}{-i-1} = \frac{1}{-(i+1)} = -\frac{1}{i+1}$.