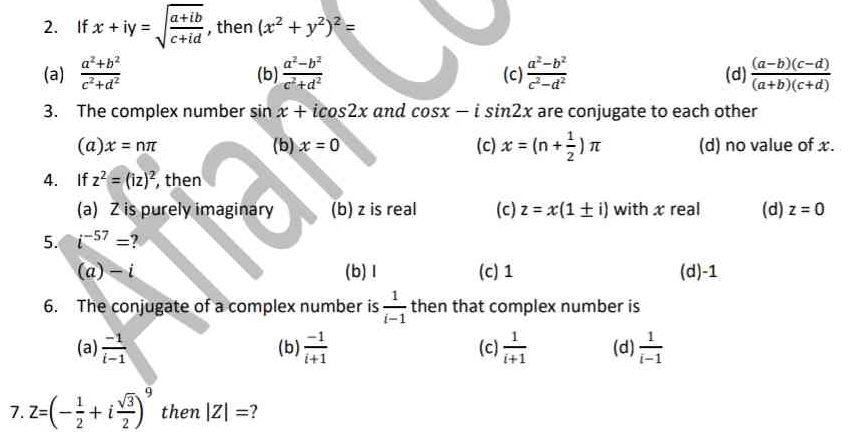
Complex number questions.

Question image

Understand the Problem

The image presents a series of complex number questions, covering topics such as:

  1. Finding the magnitude squared of a complex number given its square root representation.
  2. Determining the condition for two complex numbers to be conjugates.
  3. Analyzing the properties of a complex number under specific transformations.
  4. Simplifying powers of the imaginary unit i.
  5. Finding the complex number given it's conjugate.
  6. Finding the magnitude of a complex number raised to a power.

Answer

2. (a) $\frac{a^2+b^2}{c^2+d^2}$ 3. (d) no value of x. 4. (d) z = 0 5. (a) -i 6. (b) $\frac{-1}{i+1}$ 7. 1
Answer for screen readers
  1. (a) $\frac{a^2+b^2}{c^2+d^2}$
  2. (d) no value of x.
  3. (d) z = 0
  4. (a) -i
  5. (b) $\frac{-1}{i+1}$
  6. 1

Steps to Solve

  1. Solve for question 2 Given $x + iy = \sqrt{\frac{a+ib}{c+id}}$, we want to find $(x^2 + y^2)^2$.

First, we know that $x^2 + y^2 = |x + iy|^2$. So we need to find $|x + iy|$. $$ |x + iy| = \left| \sqrt{\frac{a+ib}{c+id}} \right| = \sqrt{\left| \frac{a+ib}{c+id} \right|} = \sqrt{\frac{|a+ib|}{|c+id|}} = \sqrt{\frac{\sqrt{a^2+b^2}}{\sqrt{c^2+d^2}}} = \left( \frac{a^2+b^2}{c^2+d^2} \right)^{1/4} $$ Therefore, $x^2 + y^2 = \left( \frac{a^2+b^2}{c^2+d^2} \right)^{1/2}$ $$ (x^2 + y^2)^2 = \left[ \left( \frac{a^2+b^2}{c^2+d^2} \right)^{1/2} \right]^2 = \frac{a^2+b^2}{c^2+d^2} $$

  1. Solve for question 3 Given that $\sin x + i\cos 2x$ and $\cos x - i\sin 2x$ are conjugates. If two complex numbers are conjugates, their real parts are equal, and their imaginary parts are the negative of each other. Therefore: $$ \sin x = \cos x $$ $$ \cos 2x = \sin 2x $$ From $\sin x = \cos x$, we have $x = n\pi + \frac{\pi}{4}$, where $n$ is an integer.

From $\cos 2x = \sin 2x$, we have $2x = m\pi + \frac{\pi}{4}$, where $m$ is an integer. Then $x = \frac{m\pi}{2} + \frac{\pi}{8}$. Equating both values of x, $n\pi + \frac{\pi}{4} = \frac{m\pi}{2} + \frac{\pi}{8}$ $n + \frac{1}{4} = \frac{m}{2} + \frac{1}{8}$ $n = \frac{m}{2} - \frac{1}{8}$ $8n = 4m - 1$ Since $n$ and $m$ are integers, $8n$ is an integer, $4m$ is an integer, but $4m-1$ is an integer. This can always be solved for integers. However, if $x=\frac{\pi}{4}$, $\sin x + i \cos 2x = \frac{\sqrt{2}}{2} + i(0) = \frac{\sqrt{2}}{2}$ $\cos x - i \sin 2x = \frac{\sqrt{2}}{2} - i(1) = \frac{\sqrt{2}}{2} - i$ These are not conjugates.

Therefore, there is no value of $x$ for which these complex numbers are conjugates.

  1. Solve for question 4 Given $z^2 = (iz)^2$, then $z^2 = i^2 z^2 = -z^2$ $z^2 = -z^2$ implies $2z^2 = 0$, so $z^2 = 0$. Therefore, $z = 0$.

  2. Solve for question 5 We want to find $i^{-57}$. We know that $i^2 = -1, i^3 = -i, i^4 = 1$. $i^{-57} = \frac{1}{i^{57}} = \frac{1}{i^{56} \cdot i} = \frac{1}{(i^4)^{14} \cdot i} = \frac{1}{1^{14} \cdot i} = \frac{1}{i} = \frac{1}{i} \cdot \frac{-i}{-i} = \frac{-i}{-i^2} = \frac{-i}{-(-1)} = \frac{-i}{1} = -i$

  3. Solve for question 6 Let $z$ be the complex number and $\bar{z}$ be its conjugate. Given $\bar{z} = \frac{1}{i-1}$. We want to find $z$. $z = \overline{(\frac{1}{i-1})} = \frac{1}{\overline{(i-1)}} = \frac{1}{\bar{i} - \bar{1}} = \frac{1}{-i - 1} = \frac{1}{-(i+1)} = \frac{-1}{i+1}$

  4. Solve for question 7 Given $z = \left(-\frac{1}{2} + i\frac{\sqrt{3}}{2}\right)^9$. We want to find $|z|$. $|z| = \left| \left(-\frac{1}{2} + i\frac{\sqrt{3}}{2}\right)^9 \right| = \left| -\frac{1}{2} + i\frac{\sqrt{3}}{2} \right|^9$ Here, $-\frac{1}{2} + i\frac{\sqrt{3}}{2} = \cos \left(\frac{2\pi}{3}\right) + i \sin \left(\frac{2\pi}{3}\right) = e^{i(2\pi/3)}$ $\left| -\frac{1}{2} + i\frac{\sqrt{3}}{2} \right| = \sqrt{\left(-\frac{1}{2}\right)^2 + \left(\frac{\sqrt{3}}{2}\right)^2} = \sqrt{\frac{1}{4} + \frac{3}{4}} = \sqrt{\frac{4}{4}} = \sqrt{1} = 1$ Then $|z| = 1^9 = 1$

  1. (a) $\frac{a^2+b^2}{c^2+d^2}$
  2. (d) no value of x.
  3. (d) z = 0
  4. (a) -i
  5. (b) $\frac{-1}{i+1}$
  6. 1

More Information

Here is some additional information about complex numbers:

  • Complex numbers are of the form $a + bi$, where $a$ and $b$ are real numbers and $i$ is the imaginary unit, defined as $i = \sqrt{-1}$.
  • The magnitude (or modulus) of a complex number $z = a + bi$ is given by $|z| = \sqrt{a^2 + b^2}$.
  • The conjugate of a complex number $z = a + bi$ is $\bar{z} = a - bi$.
  • Euler's Formula: $e^{ix} = \cos x + i \sin x$.

Tips

  • Forgetting to take the square root when finding the magnitude of complex numbers.
  • Making mistakes in algebraic manipulations, especially when dealing with conjugates and square roots.
  • Forgetting the cyclical nature of powers of i: $i^1 = i, i^2 = -1, i^3 = -i, i^4 = 1, i^5 = i$, and so on.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!