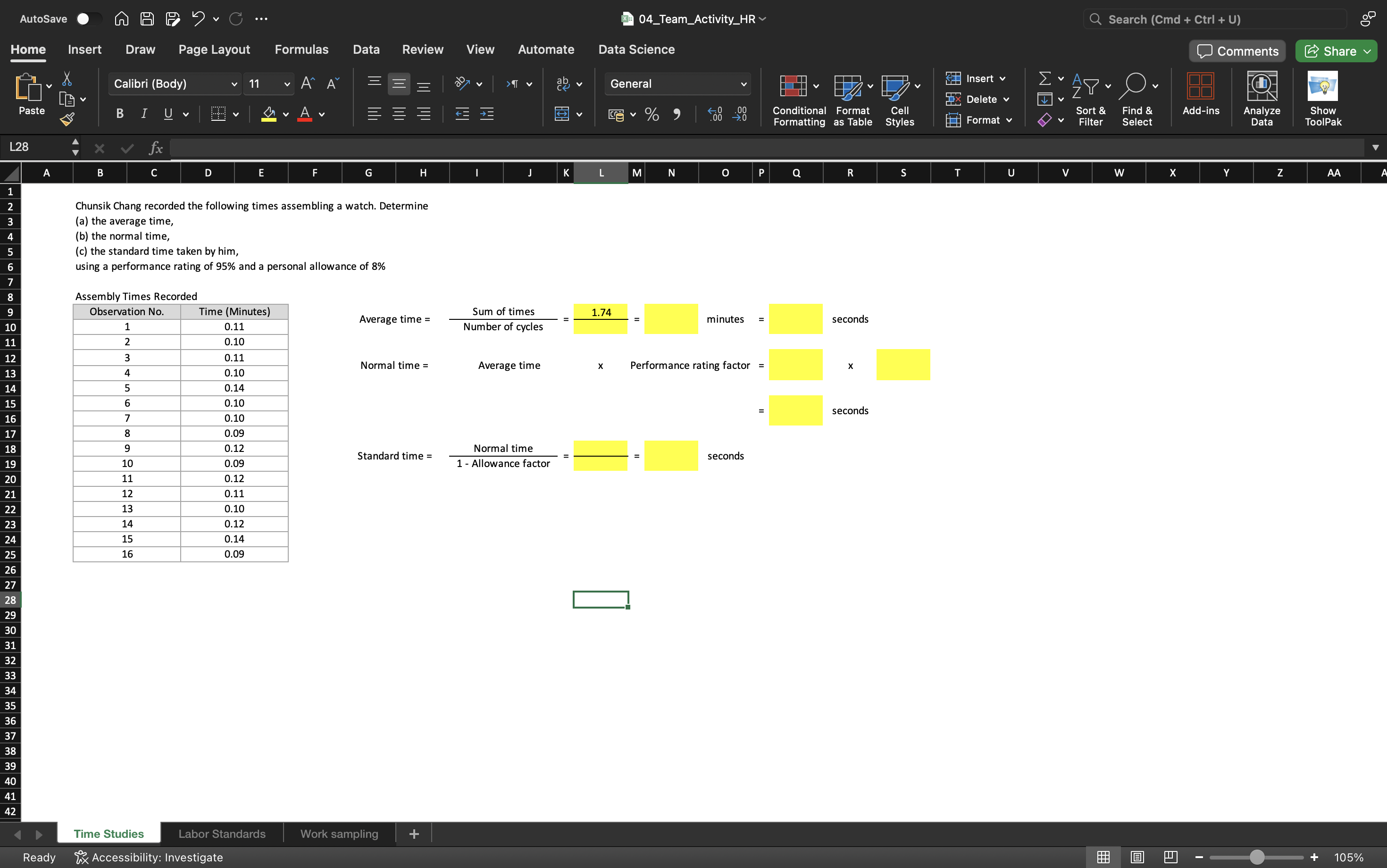
Chunsik Chang recorded the following times assembling a watch. Determine (a) the average time, (b) the normal time, (c) the standard time taken by him, using a performance rating o... Chunsik Chang recorded the following times assembling a watch. Determine (a) the average time, (b) the normal time, (c) the standard time taken by him, using a performance rating of 95% and a personal allowance of 8%.
Understand the Problem
The question requires calculating the average time, normal time, and standard time for assembling a watch based on provided observation times and given performance and allowance factors.
Answer
Average Time: $6.525$ seconds, Normal Time: $6.199$ seconds, Standard Time: $6.738$ seconds.
Answer for screen readers
- Average Time: $6.525$ seconds
- Normal Time: $6.199$ seconds
- Standard Time: $6.738$ seconds
Steps to Solve
- Calculate the Average Time
To find the average time, sum all the recorded assembly times and divide by the number of observations.
The recorded times (in minutes) are as follows: $$ \text{Times} = [0.11, 0.10, 0.11, 0.10, 0.14, 0.10, 0.09, 0.12, 0.09, 0.12, 0.12, 0.11, 0.10, 0.12, 0.14, 0.09] $$
The sum of the times is: $$ \text{Sum} = 0.11 + 0.10 + 0.11 + 0.10 + 0.14 + 0.10 + 0.09 + 0.12 + 0.09 + 0.12 + 0.12 + 0.11 + 0.10 + 0.12 + 0.14 + 0.09 = 1.74 \text{ minutes} $$
Divide the sum by the number of observations (16): $$ \text{Average Time} = \frac{1.74 \text{ minutes}}{16} \approx 0.10875 \text{ minutes} \approx 6.525 \text{ seconds} $$
- Calculate the Normal Time
Normal time is calculated using the formula: $$ \text{Normal Time} = \text{Average Time} \times \text{Performance Rating Factor} $$
Given the performance rating factor of 95% (or 0.95): $$ \text{Normal Time} = 0.10875 \text{ minutes} \times 0.95 \approx 0.1033125 \text{ minutes} $$
Convert normal time to seconds: $$ \text{Normal Time in Seconds} = 0.1033125 \times 60 \approx 6.19875 \text{ seconds} $$
- Calculate the Standard Time
Standard time is found using the formula: $$ \text{Standard Time} = \frac{\text{Normal Time}}{1 - \text{Allowance Factor}} $$
Given the allowance factor of 8% (or 0.08): $$ \text{Standard Time} = \frac{0.1033125 \text{ minutes}}{1 - 0.08} = \frac{0.1033125}{0.92} $$
Convert the standard time to seconds: $$ \text{Standard Time in Seconds} \approx \frac{0.1033125 \times 60}{0.92} \approx 6.73817 \text{ seconds} $$
- Average Time: $6.525$ seconds
- Normal Time: $6.199$ seconds
- Standard Time: $6.738$ seconds
More Information
The calculations of average, normal, and standard time are essential in time and motion studies to understand efficiency and productivity in an assembly process. They allow for better planning and allocation of resources.
Tips
- Forgetting to convert minutes to seconds, which can lead to inaccuracies in timing.
- Not properly applying the performance rating factor and allowance, which could lead to incorrect normal and standard times.
AI-generated content may contain errors. Please verify critical information