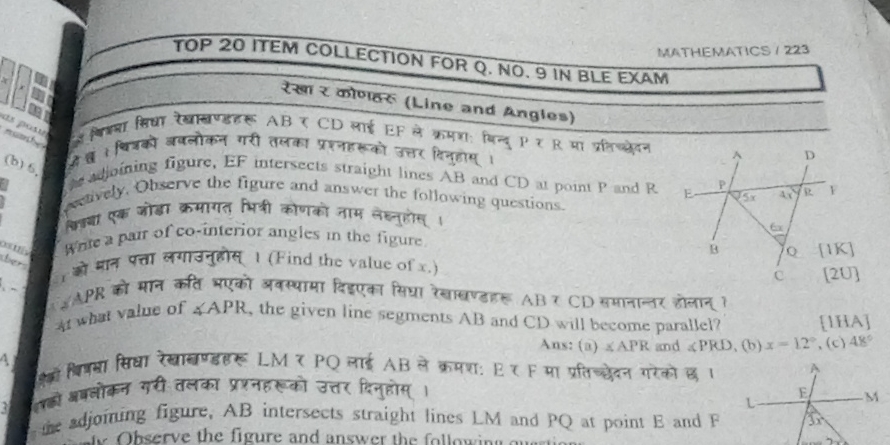
चित्रमा सह-भित्री कोणहरूको जोडा लेख्नुहोस्। x को मान पत्ता लगाउनुहोस्। कोण APR को मान कति भएमा AB र CD समानान्तर हुन्छन्? चित्रमा सह-भित्री कोणहरूको जोडा लेख्नुहोस्। x को मान पत्ता लगाउनुहोस्। कोण APR को मान कति भएमा AB र CD समानान्तर हुन्छन्? Read more

Steps to Solve

1. Identify a pair of co-interior angles

Co-interior angles are angles on the same side of the transversal and inside the two lines. In the figure, $\angle APR$ and $\angle PRQ$ are co-interior angles.

2. Find the value of x

Since $\angle APR$ and $\angle PRQ$ form a straight line, their sum is $180^\circ$. Therefore,

$5x + 6x = 180$

$11x = 180$

$x = \frac{180}{11} = 16.36$

3. Determine the value of $\angle APR$ for AB and CD to be parallel

For lines AB and CD to be parallel, the co-interior angles $\angle APR$ and $\angle PRD$ must be supplementary (add up to $180^\circ$). So, $\angle APR + \angle PRQ = 180^\circ$ if AB || CD. That is, $\angle APR$ and $\angle PRD$ have to add to $180^\circ$. Also, if AB || CD then $\angle APR = \angle CRQ$, which are corresponding angles. $\angle CRQ = 6x$ so $\angle APR$ must also equal $6x$ since $\angle CRQ$ and $\angle PRD$ are supplementary. If $\angle APR = \angle CRQ$, then $\angle APR = 6x = 6 * \frac{180}{11} = \frac{1080}{11}$. We want $\angle APR + \angle PRD = 180$, or $6x + 4x = 10x = 180$, so $x = 18$. To find the value of $\angle APR = 5x$, we can write: $\angle APR + \angle PRD = 180^\circ$

$5x + 4x = 180$

$9x = 180$

$x = 20$ Therefore, $APR = 5x = 5 \times 20 = 100$