Calculate the MU needed to deliver 200 cGy to a point 10 cm deep in a phantom with an off-axis distance of 3 cm, given the parameters from the linear accelerator calibration.
Understand the Problem
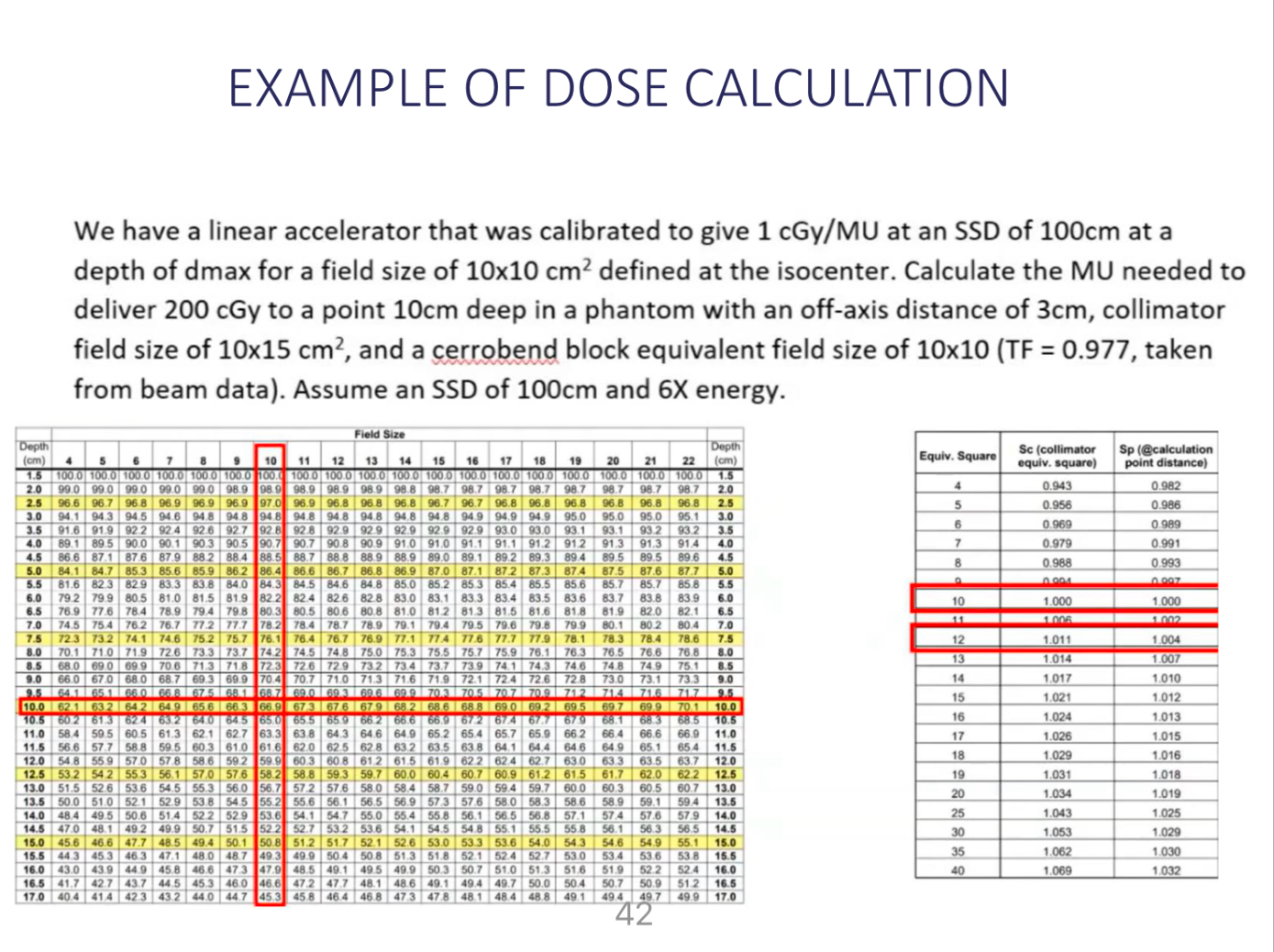
The question is asking us to calculate the monitor units (MU) needed to deliver a specific dose based on provided parameters and data, such as depth, field size, off-axis distance, and calibration values. This involves using the given information and referring to the dose calculation tables to find the appropriate factors for the calculation.
Answer
$296$
Answer for screen readers
The final answer for the monitor units (MU) needed is approximately $296$ MU.
Steps to Solve
- Identify Key Values Given the scenario, we need to gather the key values:
- Desired dose: 200 cGy
- Depth: 10 cm
- Off-axis distance: 3 cm
- Collimator field size: 10 cm x 15 cm
- Equivalent field size: 10 cm x 10 cm
- Sc (collimator equivalent square) factor and dose factors as per the table.
- Determine the Dose from MU Calculation From the calibration, we know: $$ \text{Dose delivered} = \text{MU} \times \text{Calibration factor} $$
Given that the calibration factor is 1 cGy/MU, we can express the dose in terms of MU: $$ 200 \text{ cGy} = \text{MU} \times 1 \text{ cGy/MU} $$
This allows us to simplify the equation: $$ \text{MU} = 200 $$
- Apply Correction Factors Next, we must apply the correction factors from the provided tables to account for:
- Depth dose (DD) at 10 cm.
- Collimator equivalent square correction factor (Sc).
- Tissue phantom ratio (TF).
From the dose calculation table for depth = 10 cm and field size 10x10 cm, we find: Depth dose (at 10 cm) = 67.4% (from the table).
Using the Sc factor from the right table at 10 cm:
- Sc = 1.000
- Calculate Final MU Now we adjust for depth and field size: $$ \text{MU} = \frac{200 \text{ cGy}}{0.674 \times 1.000} $$
Calculating: $$ \text{MU} = \frac{200}{0.674} \approx 296.3 $$
- Rounding Typically, you would round the MU to the nearest whole number for practical application: $$ \text{MU} \approx 296 $$
The final answer for the monitor units (MU) needed is approximately $296$ MU.
More Information
The calculation involves applying depth dose correction, collimator correction, and considering the specific field sizes to ensure proper dose delivery. In clinical practice, accurately calculating monitor units is crucial to avoid underdosing or overdosing patients.
Tips
- Failing to correctly interpret data from the dose calculation tables.
- Neglecting to apply all necessary correction factors, especially those related to depth and field size.
- Rounding errors before the final calculation rather than after.
AI-generated content may contain errors. Please verify critical information