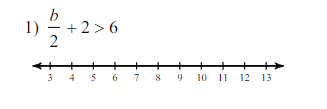
b/2 + 2 > 6
Understand the Problem
The question is asking us to solve the inequality involving the variable b, specifically b/2 + 2 > 6, and represent the solution on a number line. We need to isolate b to find its range of values that satisfy the inequality.
Answer
$b > 8$
Answer for screen readers
The solution to the inequality is $b > 8$.
Steps to Solve
- Subtract 2 from both sides
To isolate the term with $b$, subtract 2 from both sides of the inequality:
$$ \frac{b}{2} + 2 - 2 > 6 - 2 $$
This simplifies to:
$$ \frac{b}{2} > 4 $$
- Multiply both sides by 2
To eliminate the fraction, multiply both sides by 2. Since 2 is positive, the direction of the inequality will not change:
$$ 2 \cdot \frac{b}{2} > 2 \cdot 4 $$
This simplifies to:
$$ b > 8 $$
- Represent the solution on a number line
To represent the solution $b > 8$ on a number line, draw an open circle on the number 8 (indicating that 8 is not included) and shade the area to the right of 8.
The solution to the inequality is $b > 8$.
More Information
This inequality means that any value greater than 8 will satisfy the inequality. It can represent many real-world situations, such as thresholds or limits.
Tips
- A common mistake is to forget to reverse the inequality sign when multiplying or dividing by a negative number, which doesn’t apply here since 2 is positive.
- Another mistake is not representing the solution correctly on a number line; remember to use an open circle for $b > 8$.
AI-generated content may contain errors. Please verify critical information