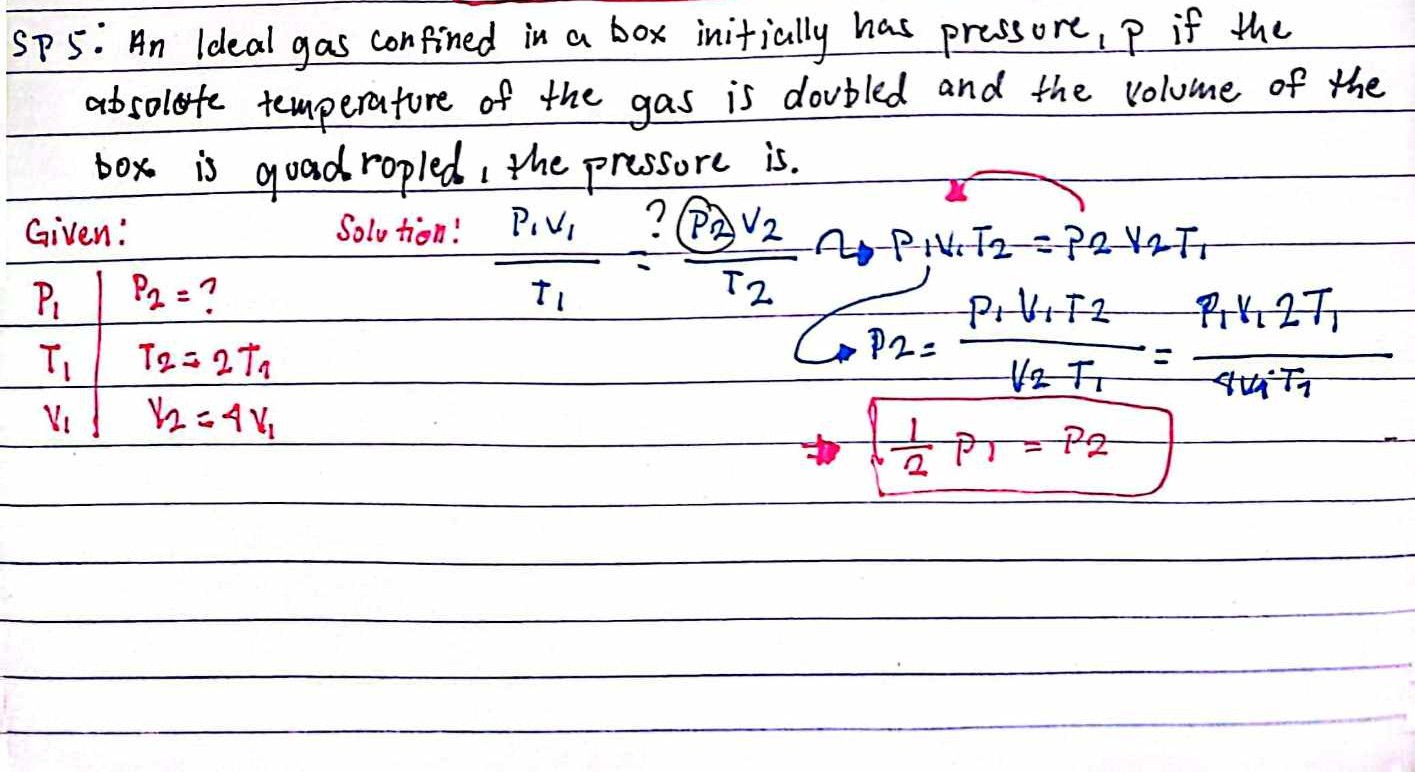
An ideal gas confined in a box initially has pressure P1. If the absolute temperature of the gas is doubled and the volume of the box is quadrupled, what is the pressure P2?
Understand the Problem
The question asks to determine the final pressure (P2) of an ideal gas in a box after its absolute temperature is doubled and the volume is quadrupled. It uses the formula relating pressure, volume, and temperature for an ideal gas.
Answer
The final pressure \( P_2 \) is $$ P_2 = \frac{1}{2} P_1 $$
Answer for screen readers
The final pressure ( P_2 ) is
$$ P_2 = \frac{1}{2} P_1 $$
Steps to Solve
- Identify the Initial Conditions
We have the initial pressure ( P_1 ), temperature ( T_1 ), and volume ( V_1 ) of the gas. The final temperature and volume are given as ( T_2 = 2 T_1 ) and ( V_2 = 4 V_1 ).
- Use the Ideal Gas Law
The ideal gas law is expressed as:
$$ P_1 V_1 = n R T_1 $$
$$ P_2 V_2 = n R T_2 $$
Where ( n ) is the number of moles and ( R ) is the gas constant.
- Relate Initial and Final States
According to the ideal gas law, we can write:
$$ \frac{P_1 V_1}{T_1} = \frac{P_2 V_2}{T_2} $$
- Substitute Known Values
Substituting ( T_2 ) and ( V_2 ):
$$ \frac{P_1 V_1}{T_1} = \frac{P_2 (4 V_1)}{2 T_1} $$
- Simplify the Equation
This simplifies to:
$$ P_1 V_1 = \frac{P_2 \cdot 4 V_1}{2} $$
Cross-multiplying gives:
$$ 2 P_1 = 4 P_2 $$
- Solve for Final Pressure ( P_2 )
Rearranging the equation results in:
$$ P_2 = \frac{1}{2} P_1 $$
Thus, the final pressure ( P_2 ) is half of the initial pressure ( P_1 ).
The final pressure ( P_2 ) is
$$ P_2 = \frac{1}{2} P_1 $$
More Information
This result illustrates the relationship between pressure, volume, and temperature for an ideal gas. When the temperature doubles and the volume quadruples, the pressure is reduced by half, showcasing how gases behave under changing conditions according to gas laws.
Tips
- Not properly substituting the values for ( T_2 ) and ( V_2 ).
- Forgetting to account for the changes in both volume and temperature, leading to incorrect final pressure calculations.
- Miscounting factors in the simplification, which could yield a different relationship.
AI-generated content may contain errors. Please verify critical information