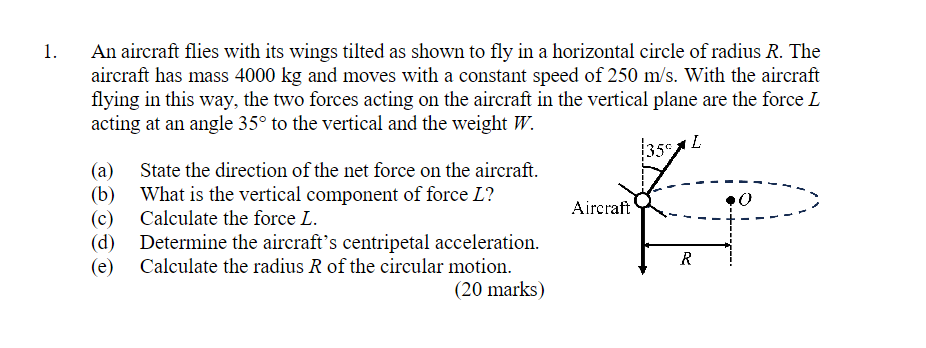
An aircraft flies with its wings tilted as shown to fly in a horizontal circle of radius R. The aircraft has mass 4000 kg and moves with a constant speed of 250 m/s. With the aircr... An aircraft flies with its wings tilted as shown to fly in a horizontal circle of radius R. The aircraft has mass 4000 kg and moves with a constant speed of 250 m/s. With the aircraft flying in this way, the two forces acting on the aircraft in the vertical plane are the force L acting at an angle 35° to the vertical and the weight W. (a) State the direction of the net force on the aircraft. (b) What is the vertical component of force L? (c) Calculate the force L. (d) Determine the aircraft's centripetal acceleration. (e) Calculate the radius R of the circular motion. Read more

Steps to Solve

1. Direction of the net force

Since the aircraft is moving in a horizontal circle, the net force must be directed towards the center of the circle. This force is the centripetal force, which is necessary to keep the aircraft moving in a circular path.

2. Vertical component of force L

The vertical component of the lift force $L$ must balance the weight $W$ of the aircraft for it to maintain a constant altitude. The weight $W$ can be calculated using the formula $W = mg$, where $m$ is the mass of the aircraft and $g$ is the acceleration due to gravity (approximately $9.8 , m/s^2$). The vertical component of $L$ is $L\cos(35^\circ)$. Therefore, $L\cos(35^\circ) = W = mg$.

3. Calculate force L

Using the equation from the previous step, we have $L\cos(35^\circ) = mg$. We can solve for $L$ by dividing both sides by $\cos(35^\circ)$:

$L = \frac{mg}{\cos(35^\circ)}$ Substituting the given values $m = 4000 , kg$ and $g = 9.8 , m/s^2$, we can calculate $L$.

4. Centripetal acceleration

The centripetal acceleration $a_c$ is related to the net force $F_{net}$ and mass $m$ by the equation $F_{net} = ma_c$. The net force in this case is the horizontal component of the lift force $L$, which is given by $L\sin(35^\circ)$. Therefore, $L\sin(35^\circ) = ma_c$, and we can solve for $a_c$:

$a_c = \frac{L\sin(35^\circ)}{m}$.

5. Radius of the circular motion

The centripetal acceleration is also related to the speed $v$ and the radius $R$ of the circular motion by the equation $a_c = \frac{v^2}{R}$. We can solve for $R$ using the calculated value of $a_c$ and the given speed $v = 250 , m/s$:

$R = \frac{v^2}{a_c}$