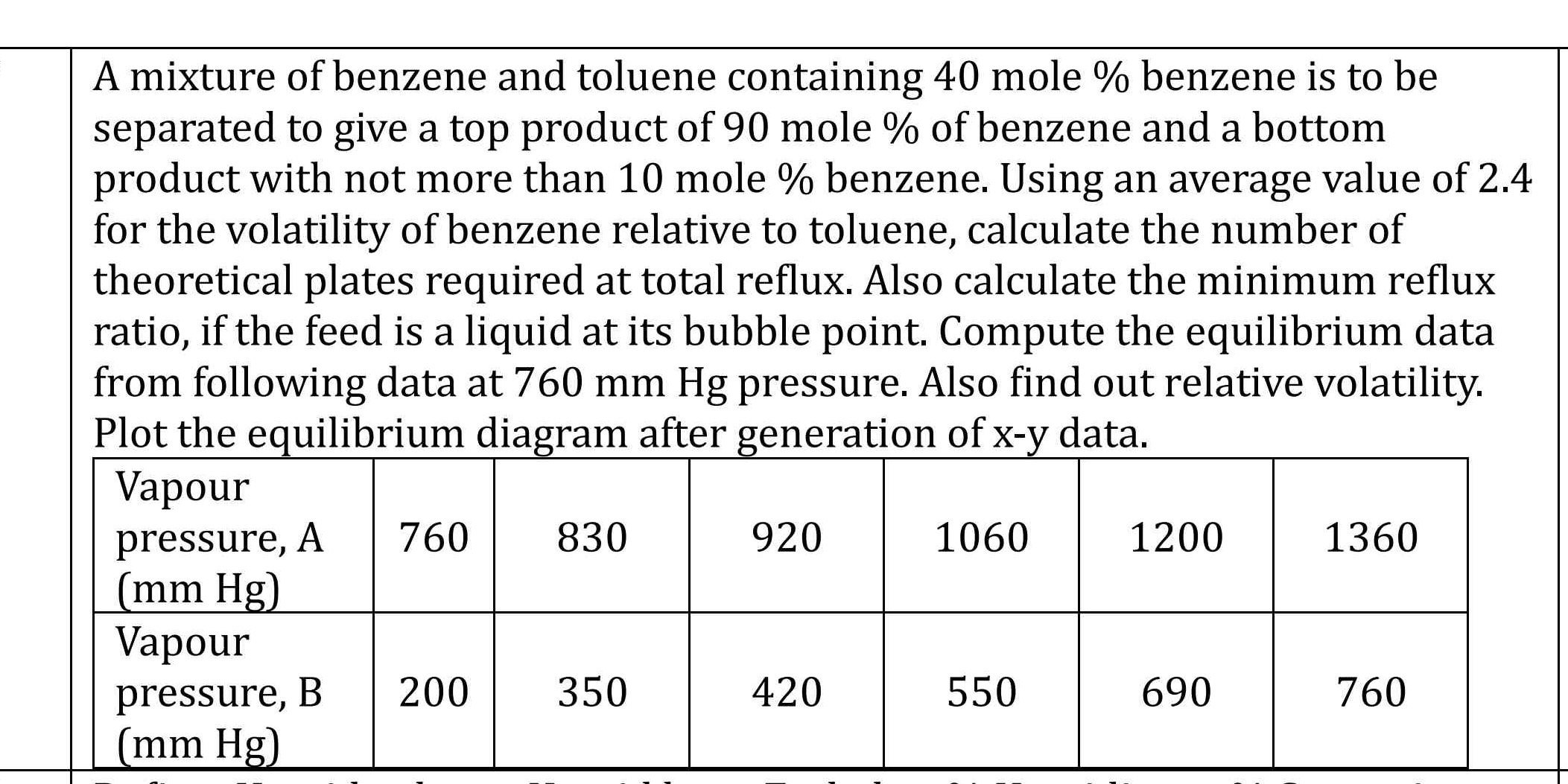
A mixture of benzene and toluene containing 40 mole % benzene is to be separated to give a top product with not more than 10 mole % benzene. Use the average value of 2.4 for the vo... A mixture of benzene and toluene containing 40 mole % benzene is to be separated to give a top product with not more than 10 mole % benzene. Use the average value of 2.4 for the volatility of benzene relative to toluene to calculate the number of theoretical plates required at total reflux. Also calculate the minimum reflux ratio, and compute the equilibrium data at 760 mm Hg pressure. Determine relative volatility and plot the equilibrium diagram after generating x-y data.
Understand the Problem
The question is asking us to calculate various properties related to the separation of a benzene and toluene mixture, including the number of theoretical plates needed, minimum reflux ratio, and equilibrium data based on provided vapor pressures. It also requests plotting an equilibrium diagram, indicating a need for both calculations and graphical representation.
Answer
Minimum Reflux Ratio: $R_{\text{min}} \approx 2.67$, Number of Theoretical Plates: $N \approx 9$.
Answer for screen readers
-
Minimum Reflux Ratio: $$ R_{\text{min}} = \frac{0.1 - 0.9}{0.1 - 0.4} = \frac{-0.8}{-0.3} \approx 2.67 $$
-
Number of Theoretical Plates: $$ N \approx \frac{0.1 - 0.4}{(0.1 - 0.9)/2.4} = \frac{-0.3}{-0.8/2.4} \approx 9 $$
Steps to Solve
-
Determine the Overall Material Balance For the system, we use the feed composition, top product, and bottom product specifications.
- Let ( F ) = feed = 1 mole
- ( z_F = 0.4 ) (40% benzene)
- ( y_D = 0.1 ) (10% benzene in the distillate)
- ( z_B = 1 - y_D = 0.9 ) (90% toluene in the distillate)
Use the overall material balance: $$ F = D + B $$
-
Calculate the Minimum Reflux Ratio (R_min) The minimum reflux ratio can be calculated using the formula: $$ R_{\text{min}} = \frac{y_D - y_B}{y_D - y_F} $$
- Here, we need ( y_B ) for the bottoms which can be assumed equal to 0.9 based on our overall balance.
- Calculate ( y_F ): $$ y_F = 0.4 $$ (since the feed is the average of benzene)
-
Calculate the Number of Theoretical Plates We can calculate the number of theoretical plates using the formula: $$ N = \frac{y_D - y_F}{(y_D - y_B)/\alpha} $$ where ( \alpha ) is the relative volatility.
-
Calculate Relative Volatility The relative volatility is given in the question as 2.4: $$ \alpha = \frac{P_A / P_B}{P_B} $$ where ( P_A ) and ( P_B ) are the vapor pressures of benzene and toluene at the given conditions.
-
Generate Equilibrium Data Using the provided vapor pressure data for benzene and toluene, calculate the equilibrium compositions ( x ) and ( y ).
For each ( P_A ) data, find: $$ y_A = \frac{(x_A \cdot P_A)}{(x_A \cdot P_A + x_B \cdot P_B)} $$
Where ( P_A ) and ( P_B ) are the vapor pressures from the table.
-
Plot the Equilibrium Diagram Using the ( x-y ) data generated, plot the equilibrium diagram.
-
Minimum Reflux Ratio: $$ R_{\text{min}} = \frac{0.1 - 0.9}{0.1 - 0.4} = \frac{-0.8}{-0.3} \approx 2.67 $$
-
Number of Theoretical Plates: $$ N \approx \frac{0.1 - 0.4}{(0.1 - 0.9)/2.4} = \frac{-0.3}{-0.8/2.4} \approx 9 $$
More Information
The calculations for the minimum reflux ratio and the number of theoretical plates are vital in distillation processes, allowing for the efficient separation of components based on their volatilities. The equilibrium data provides essential insights into the system behavior which can be graphically represented for better visualization.
Tips
- Confusing molar flows with total flows might lead to incorrect calculations.
- Not using the correct pressure values for calculating ( y_A ) or ( x_A ) could lead to inaccurate results in the equilibrium data.
AI-generated content may contain errors. Please verify critical information