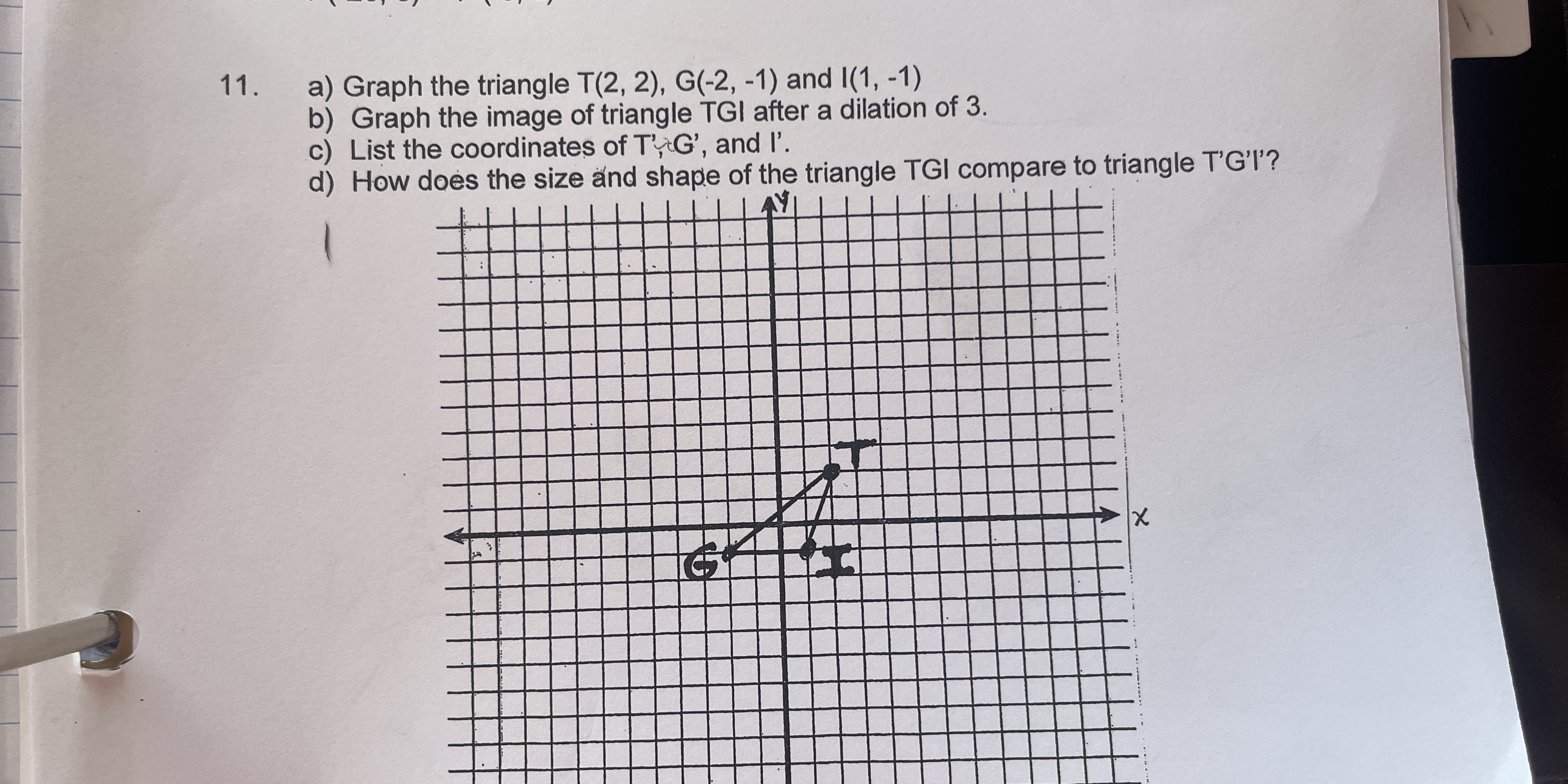
a) Graph the triangle T(2, 2), G(-2, -1) and I(1, -1). b) Graph the image of triangle TGI after a dilation of 3. c) List the coordinates of T', G', and I'. d) How does the size an... a) Graph the triangle T(2, 2), G(-2, -1) and I(1, -1). b) Graph the image of triangle TGI after a dilation of 3. c) List the coordinates of T', G', and I'. d) How does the size and shape of the triangle TGI compare to triangle T'G'I'?
Understand the Problem
The question involves geometric transformations of a triangle on a coordinate plane. Specifically, it asks to graph a triangle given its vertices, perform a dilation on the triangle, determine the coordinates of the transformed triangle, and compare the size and shape of the original and transformed triangles.
Answer
a) Triangle TGI is graphed with vertices T(2, 2), G(-2, -1), and I(1, -1). b) Triangle T'G'I' is graphed with vertices obtained after dilation. c) $T'(6, 6)$, $G'(-6, -3)$, $I'(3, -3)$ d) Triangle T'G'I' is larger and similar to triangle TGI.
Answer for screen readers
a) Triangle TGI is graphed with vertices T(2, 2), G(-2, -1), and I(1, -1). b) Triangle T'G'I' is graphed with vertices obtained after dilation. c) The coordinates of the vertices of the dilated triangle are: $T'(6, 6)$ $G'(-6, -3)$ $I'(3, -3)$ d) Triangle T'G'I' is larger than triangle TGI, and its sides are three times as long. The two triangles are similar.
Steps to Solve
- Graph triangle TGI
Plot the points T(2, 2), G(-2, -1), and I(1, -1) on the coordinate plane and connect them to form triangle TGI.
- Perform the dilation
To dilate triangle TGI by a factor of 3, multiply the coordinates of each vertex by 3. This gives us the new vertices T', G', and I'. $T'(23, 23) = T'(6, 6)$ $G'(-23, -13) = G'(-6, -3)$ $I'(13, -13) = I'(3, -3)$
- Graph the dilated triangle T'G'I'
Plot the points T'(6, 6), G'(-6, -3), and I'(3, -3) on the coordinate plane and connect them to form triangle T'G'I'.
- List the coordinates of T', G', and I'
The coordinates are: T'(6, 6) G'(-6, -3) I'(3, -3)
- Compare the size and shape
Triangle T'G'I' is larger than triangle TGI. The side lengths of T'G'I' are 3 times the side lengths of triangle TGI. The shape of the triangles are similar, meaning they have the same angles.
a) Triangle TGI is graphed with vertices T(2, 2), G(-2, -1), and I(1, -1). b) Triangle T'G'I' is graphed with vertices obtained after dilation. c) The coordinates of the vertices of the dilated triangle are: $T'(6, 6)$ $G'(-6, -3)$ $I'(3, -3)$ d) Triangle T'G'I' is larger than triangle TGI, and its sides are three times as long. The two triangles are similar.
More Information
A dilation is a transformation that changes the size of a figure but not its shape. A dilation by a factor of $k$ multiplies all the side lengths of the figure by $k$.
Tips
- Incorrectly multiplying the coordinates during dilation.
- Misplotting points on the coordinate plane.
- Confusing dilation with other types of transformations, such as translation or rotation.
AI-generated content may contain errors. Please verify critical information