A full wave bridge junction diode rectifier uses a load resistance of 1200 ohms. The internal resistance of each diode is 9 ohms. Find the efficiency.
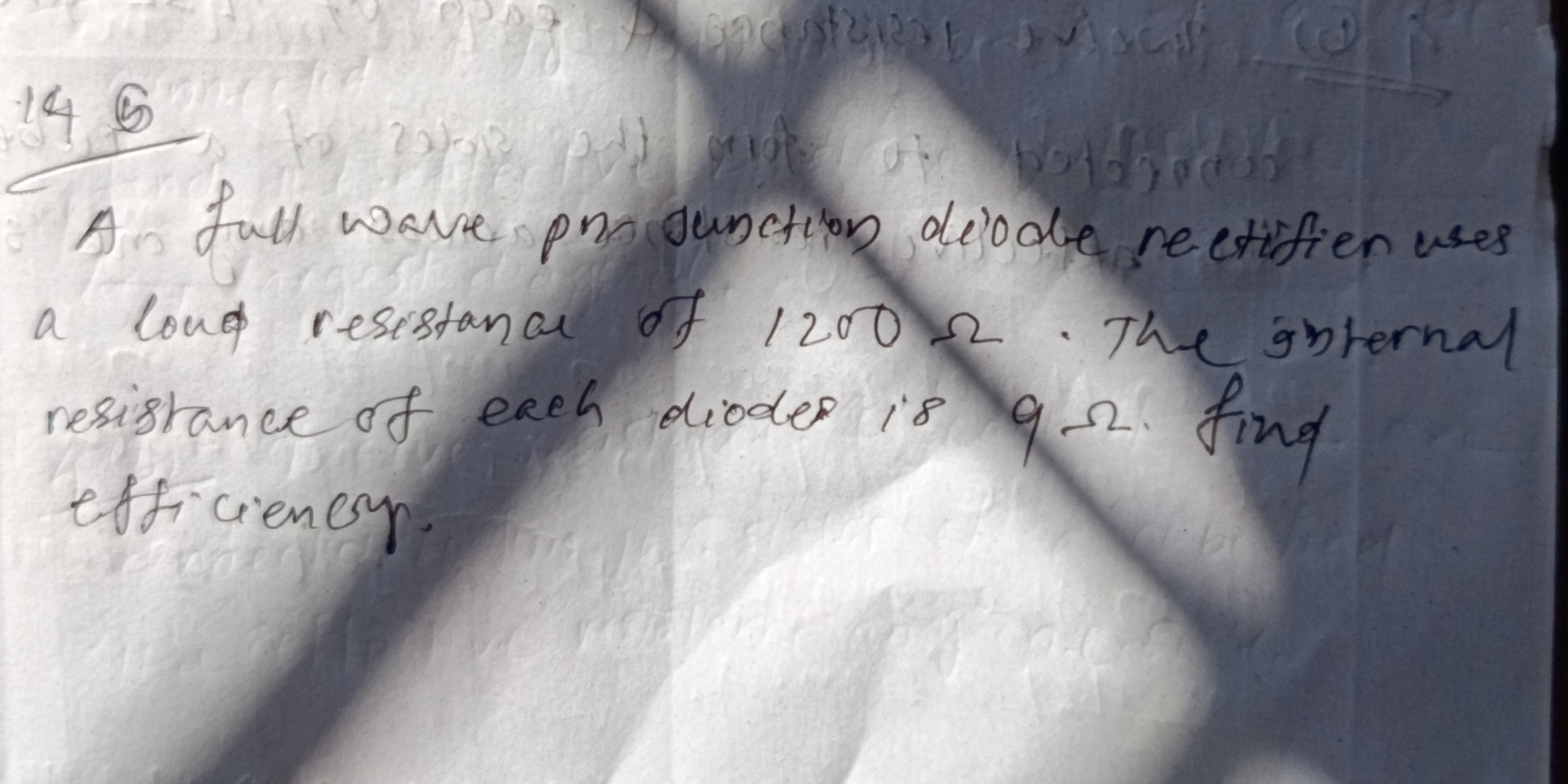
Understand the Problem
The question is asking to calculate the efficiency of a full-wave bridge rectifier that uses a load resistance of 1200 ohms and has diodes with an internal resistance of 9 ohms each. We will find the efficiency using the given values.
Answer
The efficiency of the rectifier is approximately $98.52\%$.
Answer for screen readers
The efficiency of the full-wave bridge rectifier is approximately
$$ \eta \approx 98.52% $$
Steps to Solve
-
Understand the components
The full-wave bridge rectifier consists of four diodes in configuration. The equivalent resistance of the two conducting diodes (since two diodes conduct at a time) is given by:
$$ R_d = 2 \times 9 , \Omega = 18 , \Omega $$ -
Determine total resistance
The total resistance in the circuit can be found by adding the load resistance ($R_L$) and the equivalent resistance of the diodes ($R_d$):
$$ R_{total} = R_L + R_d = 1200 , \Omega + 18 , \Omega = 1218 , \Omega $$ -
Calculate the output voltage
Assuming the input voltage ($V_s$) is the peak voltage of the AC supply, the output voltage across the load resistance ($V_{out}$) is then approximately:
$$ V_{out} = V_s - 2V_f $$
where $V_f$ is the forward voltage drop across each diode. Assuming $V_f \approx 0.7 , V$, the equation becomes:
$$ V_{out} = V_s - 2(0.7) = V_s - 1.4 , V $$ -
Calculate load current
The load current ($I_L$) can be calculated using Ohm's law:
$$ I_L = \frac{V_{out}}{R_L} = \frac{V_s - 1.4}{1200} $$ -
Determine output power ($P_{out}$)
The output power can be expressed as:
$$ P_{out} = I_L^2 \cdot R_L $$
Substituting $I_L$ we have:
$$ P_{out} = \left(\frac{V_s - 1.4}{1200}\right)^2 \cdot 1200 $$ -
Determine input power ($P_{in}$)
The power dissipated in the internal diode resistance can be calculated as:
$$ P_{in} = I_L^2 \cdot R_{total} = \left(\frac{V_s - 1.4}{1200}\right)^2 \cdot 1218 $$ -
Calculate efficiency
Finally, the efficiency ($\eta$) can be calculated as:
$$ \eta = \frac{P_{out}}{P_{in}} \times 100% $$
Since $P_{out}$ and $P_{in}$ have a common term, we can simplify efficiency calculation into:
$$ \eta = \frac{R_L}{R_{total}} \times 100% $$
Substituting the values gives:
$$ \eta = \frac{1200}{1218} \times 100% $$
The efficiency of the full-wave bridge rectifier is approximately
$$ \eta \approx 98.52% $$
More Information
The efficiency of a rectifier indicates how well it converts input AC power to DC power. In this case, the high efficiency indicates a relatively effective conversion process, which is typical for full-wave rectifiers.
Tips
- Not considering the voltage drop across the diodes when calculating the output voltage.
- Forgetting to include both resistances (load and diode resistance) when calculating total resistance.
- Misapplying Ohm's law in current and power calculations.
AI-generated content may contain errors. Please verify critical information