7√7 - 2√7
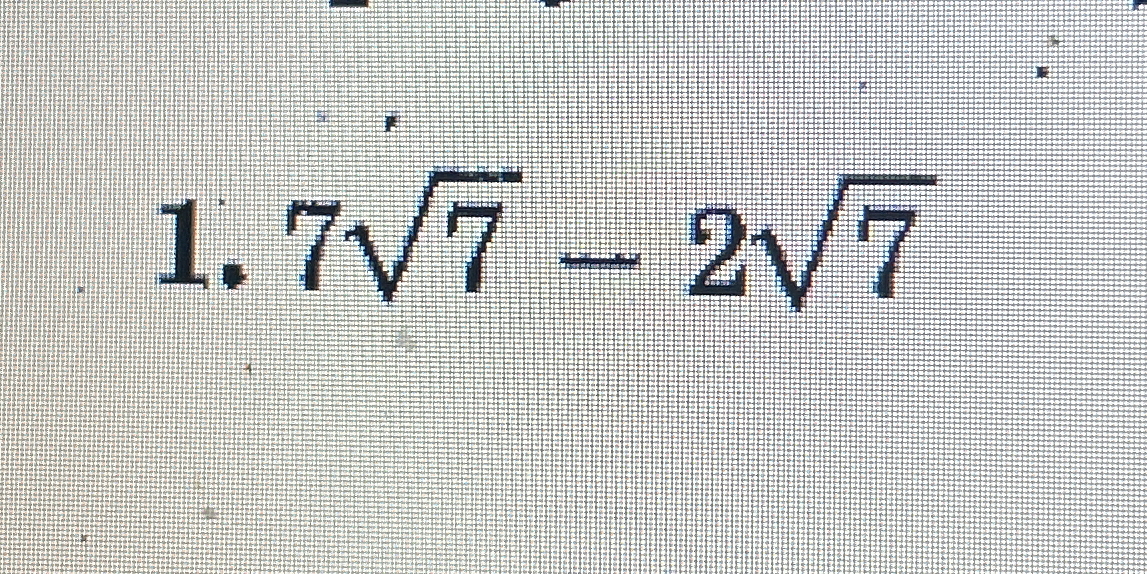
Understand the Problem
The question involves simplifying the expression 7√7 - 2√7. It requires combining like terms involving square roots.
Answer
The simplified expression is \( 5\sqrt{7} \).
Answer for screen readers
The simplified expression is ( 5\sqrt{7} ).
Steps to Solve
- Identify Like Terms
The expression consists of two terms that both involve $\sqrt{7}$. The terms are $7\sqrt{7}$ and $-2\sqrt{7}$.
- Combine Like Terms
To combine the like terms, we'll add their coefficients. This gives us:
$$ (7 - 2)\sqrt{7} $$
- Perform the Subtraction
Calculate the coefficients:
$$ 7 - 2 = 5 $$
So the expression simplifies to:
$$ 5\sqrt{7} $$
The simplified expression is ( 5\sqrt{7} ).
More Information
In this expression, the square root of 7 remains, but the coefficients have been simplified by combining like terms.
Tips
- A common mistake is treating the square root term as if it were a separate variable. It’s important to remember that you can only combine terms that have the same radical.
AI-generated content may contain errors. Please verify critical information
Thank you for voting!
