(5a²b³c⁴)(6a³b⁴c²)
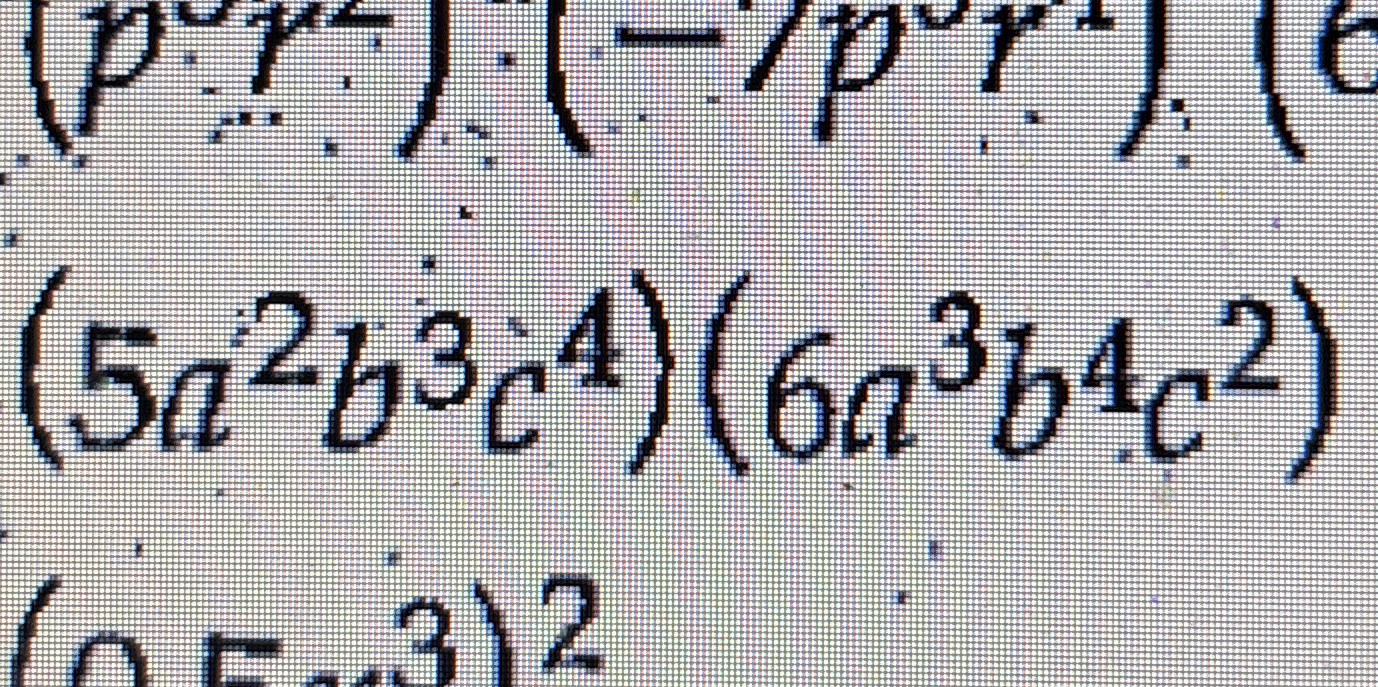
Understand the Problem
The question involves multiplying two algebraic expressions, (5a²b³c⁴) and (6a³b⁴c²), to find the product. We will combine like terms and simplify the expression.
Answer
$$ 30a^{5}b^{7}c^{6} $$
Answer for screen readers
The product of the two expressions is:
$$ 30a^{5}b^{7}c^{6} $$
Steps to Solve
- Multiply the coefficients
Multiply the numerical coefficients:
$5 \times 6 = 30$
- Combine like terms for $a$
Add the exponents of $a$:
$2 + 3 = 5$
So, $a^2 \times a^3 = a^5$
- Combine like terms for $b$
Add the exponents of $b$:
$3 + 4 = 7$
So, $b^3 \times b^4 = b^7$
- Combine like terms for $c$
Add the exponents of $c$:
$4 + 2 = 6$
So, $c^4 \times c^2 = c^6$
- Combine all parts together
Combine the results of the coefficients and variables into one expression:
$$ 30a^{5}b^{7}c^{6} $$
The product of the two expressions is:
$$ 30a^{5}b^{7}c^{6} $$
More Information
This expression represents the product of two algebraic terms with different variables raised to various powers. The coefficients are multiplied together while the exponents are summed for like bases.
Tips
- Forgetting to add the exponents for one of the variables.
- Miscalculating the coefficient multiplication.
- Not applying the rules for exponents properly.
AI-generated content may contain errors. Please verify critical information