-4/9 - (-1/4)
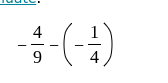
Understand the Problem
The question is asking to simplify the expression shown in the image, which involves fractions and parentheses.
Answer
The simplified expression is $-\frac{7}{36}$.
Answer for screen readers
The simplified expression is: $$ -\frac{7}{36} $$
Steps to Solve
-
Rewrite the expression
Start with the given expression: $$ -\frac{4}{9} - \left(-\frac{1}{4}\right) $$
-
Simplify the double negative
The expression inside the parentheses has a negative sign. When you subtract a negative, it becomes addition: $$ -\frac{4}{9} + \frac{1}{4} $$
-
Find a common denominator
The denominators are 9 and 4. The least common multiple (LCM) of 9 and 4 is 36. Rewrite each fraction with the common denominator: $$ -\frac{4}{9} = -\frac{4 \times 4}{9 \times 4} = -\frac{16}{36} $$ $$ \frac{1}{4} = \frac{1 \times 9}{4 \times 9} = \frac{9}{36} $$
-
Combine the fractions
Now combine the two fractions: $$ -\frac{16}{36} + \frac{9}{36} = \frac{-16 + 9}{36} = \frac{-7}{36} $$
The simplified expression is: $$ -\frac{7}{36} $$
More Information
This process illustrates how to handle negative signs in algebra and how to find a common denominator when adding or subtracting fractions.
Tips
- Forgetting that subtracting a negative becomes addition.
- Incorrectly finding the common denominator; remember to use the least common multiple.
AI-generated content may contain errors. Please verify critical information