4.32 - 12.751 = ? 9.37 + (-4.63) = ?

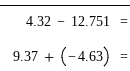
Understand the Problem

The question consists of two mathematical problems: one is a subtraction and the other is an addition involving a negative number. We will solve both expressions step by step.

Answer

1. $4.32 - 12.751 = -8.431$ 2. $9.37 + (-4.63) = 4.74$

Steps to Solve

Subtraction of the first expression

To solve $4.32 - 12.751$, we align the numbers vertically for easier subtraction:

   4.320
- 12.751

Next, we can proceed from right to left, borrowing as needed:

Starting from the thousandths place: $0 - 1$ needs borrowing, which turns our $4.320$ into $4.319$ and the $0$ becomes $10$. Now, $10 - 1 = 9$.
In the hundredths place: $2 - 5$, borrow again, resulting in $12 - 5 = 7$ after borrowing.
For the tenths place: $2 - 7$, again borrow. Now we calculate $12 - 7 = 5$.
Finally, in the units place: $3 - 2 = 1$ after borrowing.
The outcome is $-8.431$.

So, $4.32 - 12.751 = -8.431$.

Addition of the second expression

To solve $9.37 + (-4.63)$, we can rewrite this as $9.37 - 4.63$.

Align the numbers for simplification:

   9.370
-  4.630

Now, perform the subtraction:

In the thousandths place: $0 - 0 = 0$.
In the hundredths place: $7 - 3 = 4$.
In the tenths place: $3 - 6$ requires borrowing, so we have $13 - 6 = 7$ after borrowing.
In the units place: $8 - 4 = 5$.

Thus, $9.37 + (-4.63) = 4.74$.

The answers are:

$4.32 - 12.751 = -8.431$
$9.37 + (-4.63) = 4.74$

More Information

When subtracting a larger number from a smaller number, the result is negative. In the second expression, adding a negative number is equivalent to subtraction. It’s important to align decimals correctly for clear calculations.

Tips

Forgetting to borrow when required, leading to incorrect results in subtraction.
Misinterpreting addition with negatives as simply adding numbers rather than subtracting.

Thank you for voting!