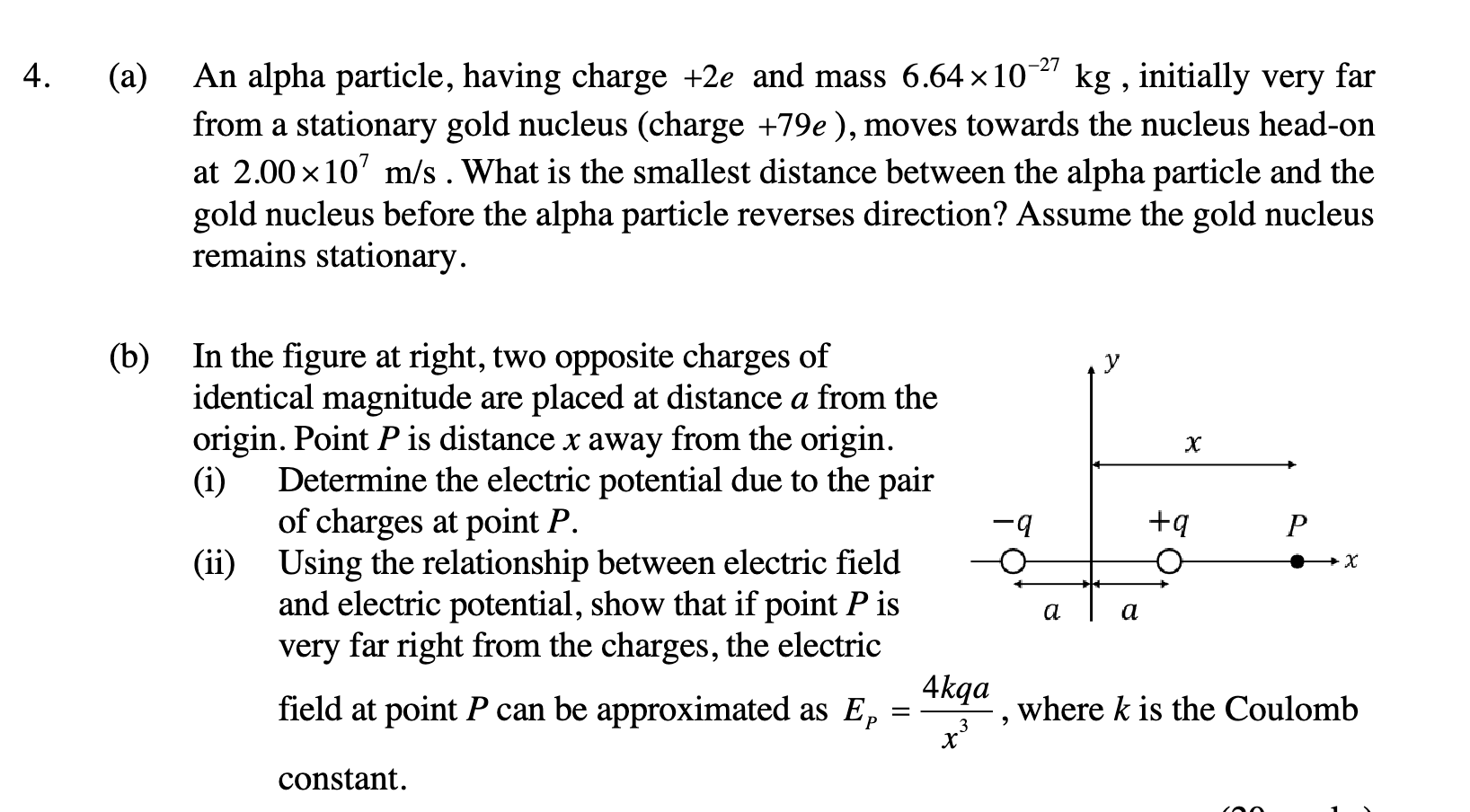
4. (a) An alpha particle, having charge +2e and mass 6.64 x 10^-27 kg, initially very far from a stationary gold nucleus (charge +79e), moves towards the nucleus head-on at 2.00 x... 4. (a) An alpha particle, having charge +2e and mass 6.64 x 10^-27 kg, initially very far from a stationary gold nucleus (charge +79e), moves towards the nucleus head-on at 2.00 x 10^7 m/s. What is the smallest distance between the alpha particle and the gold nucleus before the alpha particle reverses direction? Assume the gold nucleus remains stationary. (b) In the figure at right, two opposite charges of identical magnitude are placed at distance a from the origin. Point P is distance x away from the origin. (i) Determine the electric potential due to the pair of charges at point P. (ii) Using the relationship between electric field and electric potential, show that if point P is very far right from the charges, the electric field at point P can be approximated as Ep = 4kqa/x^3, where k is the Coulomb constant. Read more

Steps to Solve

1. Part (a): Conservation of Energy

At the point of closest approach, all the initial kinetic energy of the alpha particle is converted into electrostatic potential energy. Thus:

$KE_{initial} = PE_{final}$

$ \frac{1}{2}mv^2 = \frac{k q_1 q_2}{r} $

where:

• $m$ is the mass of the alpha particle
• $v$ is the initial velocity of the alpha particle
• $k$ is Coulomb's constant ($8.99 \times 10^9 Nm^2/C^2$)
• $q_1$ is the charge of the alpha particle ($2e = 2 \times 1.602 \times 10^{-19} C$)
• $q_2$ is the charge of the gold nucleus ($79e = 79 \times 1.602 \times 10^{-19} C$)
• $r$ is the smallest distance between the particles

2. Solving for r in Part (a)

Rearrange the equation to solve for $r$:

$ r = \frac{k q_1 q_2}{\frac{1}{2}mv^2} = \frac{2kq_1q_2}{mv^2}$

Plug in the given values:

$r = \frac{2 \times 8.99 \times 10^9 \times (2 \times 1.602 \times 10^{-19}) \times (79 \times 1.602 \times 10^{-19})}{(6.64 \times 10^{-27}) \times (2.00 \times 10^7)^2}$

3. Calculate r in Part (a)

$r \approx 2.74 \times 10^{-14} m$

4. Part (b)(i): Electric Potential

The electric potential at point P due to a point charge is given by $V = \frac{kq}{r}$, where $r$ is the distance from the charge to the point P. The total potential is the sum of the potentials due to each charge.

Distance from $+q$ to P is $x-a$. Distance from $-q$ to P is $x+a$.

The electric potential at P is:

$V_p = \frac{kq}{x-a} + \frac{k(-q)}{x+a} = kq(\frac{1}{x-a} - \frac{1}{x+a})$

5. Simplify the expression for $V_p$

$V_p = kq(\frac{(x+a)-(x-a)}{(x-a)(x+a)}) = kq(\frac{2a}{x^2 - a^2})$

$V_p = \frac{2kqa}{x^2 - a^2}$

6. Part (b)(ii): Electric Field Approximation

The electric field E is related to the electric potential V by $E = -\frac{dV}{dx}$.

$E_p = -\frac{d}{dx} (\frac{2kqa}{x^2 - a^2}) = -2kqa \frac{d}{dx} (x^2 - a^2)^{-1} $

7. Differentiate with respect to x

Using the chain rule: $\frac{d}{dx} (x^2 - a^2)^{-1} = -1 (x^2 - a^2)^{-2} (2x) = \frac{-2x}{(x^2 - a^2)^2} $

$E_p = -2kqa(\frac{-2x}{(x^2 - a^2)^2}) = \frac{4kqax}{(x^2 - a^2)^2} $

8. Approximation for large x

If $x$ is very large, $x >> a$, then $x^2 - a^2 \approx x^2$. Thus:

$E_p \approx \frac{4kqax}{(x^2)^2} = \frac{4kqax}{x^4} = \frac{4kqa}{x^3}$