-3/4 - (-7/6)
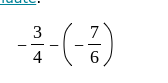
Understand the Problem
The question is asking us to simplify the expression which involves fractions. The main approach to solve this will include combining the fractions and simplifying them further if possible.
Answer
The result is $\frac{5}{12}$.
Answer for screen readers
The simplified expression is $\frac{5}{12}$.
Steps to Solve
-
Rewrite the Expression We start by rewriting the expression:
$$ -\frac{3}{4} - \left( -\frac{7}{6} \right) = -\frac{3}{4} + \frac{7}{6} $$
This simplifies our calculations since subtracting a negative is the same as adding. -
Find a Common Denominator The denominators are 4 and 6.
The least common multiple (LCM) of 4 and 6 is 12. -
Convert Fractions to a Common Denominator We convert each fraction:
$$ -\frac{3}{4} = -\frac{3 \times 3}{4 \times 3} = -\frac{9}{12} $$
$$ \frac{7}{6} = \frac{7 \times 2}{6 \times 2} = \frac{14}{12} $$ -
Combine the Fractions Now that both fractions have a common denominator, we can combine them:
$$ -\frac{9}{12} + \frac{14}{12} = \frac{-9 + 14}{12} = \frac{5}{12} $$ -
Final Simplification The final result is already simplified as:
$$ \frac{5}{12} $$
The simplified expression is $\frac{5}{12}$.
More Information
This type of problem is common in basic arithmetic and pre-algebra, focusing on fractions and their operations. Understanding how to manipulate negative signs is also crucial in mathematical operations.
Tips
- Confusing subtraction of a negative with addition directly without rewriting it. Always remember that $ -(-x) = +x$.
- Failing to convert to a common denominator correctly. Always check that both fractions are expressed over the same base before combining.
AI-generated content may contain errors. Please verify critical information