3² × 3⁴ × 3⁸
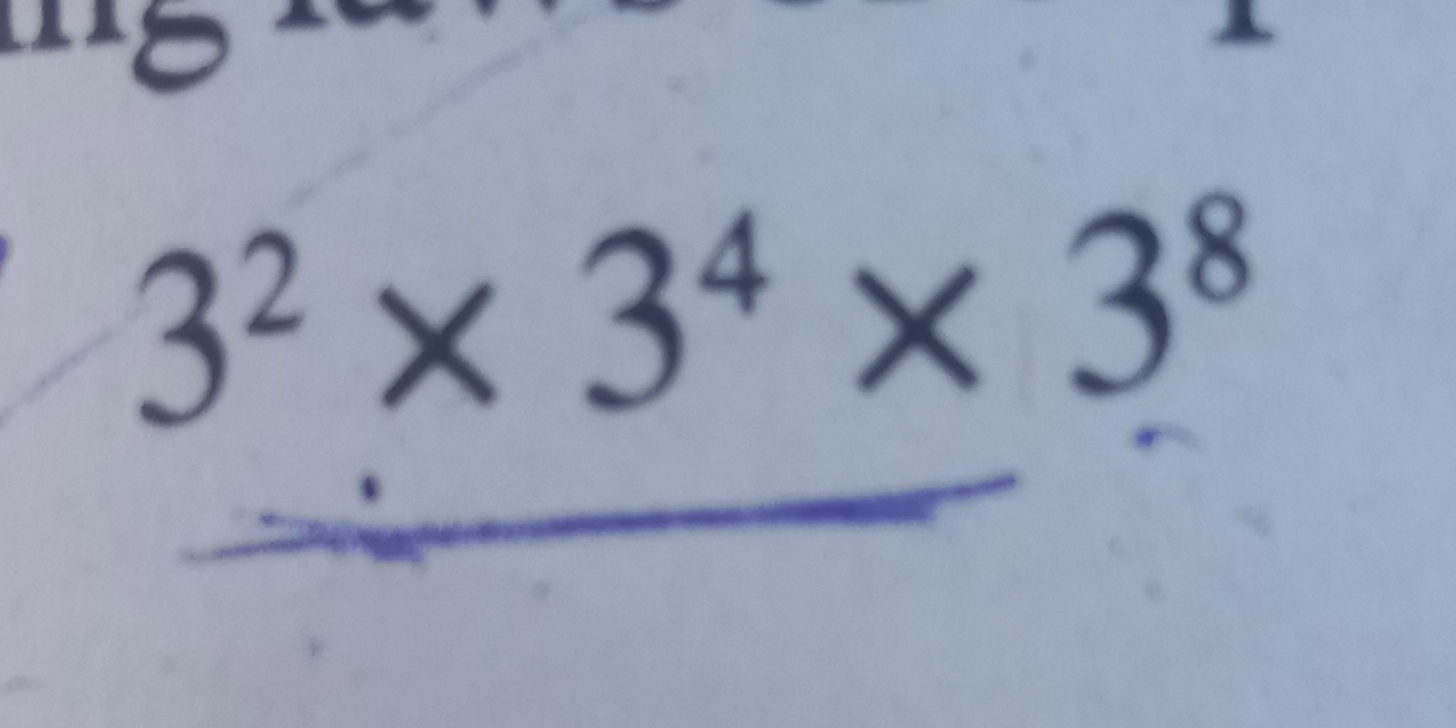
Understand the Problem
The question involves using the laws of exponents to simplify the expression involving powers of 3. Specifically, it is asking for the simplification of the product 3² × 3⁴ × 3⁸.
Answer
The simplified expression is \( 3^{14} \).
Answer for screen readers
The simplified expression is ( 3^{14} ).
Steps to Solve
-
Apply the Laws of Exponents According to the laws of exponents, when multiplying with the same base, we add the exponents. In this case, we can rewrite the expression: $$ 3^2 \times 3^4 \times 3^8 = 3^{2 + 4 + 8} $$
-
Calculate the Sum of the Exponents Now, we add the exponents: $$ 2 + 4 + 8 = 14 $$
-
Rewrite the Expression Substituting back into the expression gives us: $$ 3^{14} $$
The simplified expression is ( 3^{14} ).
More Information
The expression ( 3^{14} ) represents a large number. In actual value, ( 3^{14} = 4782969 ). This simplification is useful in various mathematical contexts, such as combinatorics and algebra.
Tips
- Miscalculating the sum of the exponents. Always double-check the arithmetic when adding the exponents together.
- Forgetting to apply the law of exponents correctly and treating the base as if it were different.
AI-generated content may contain errors. Please verify critical information