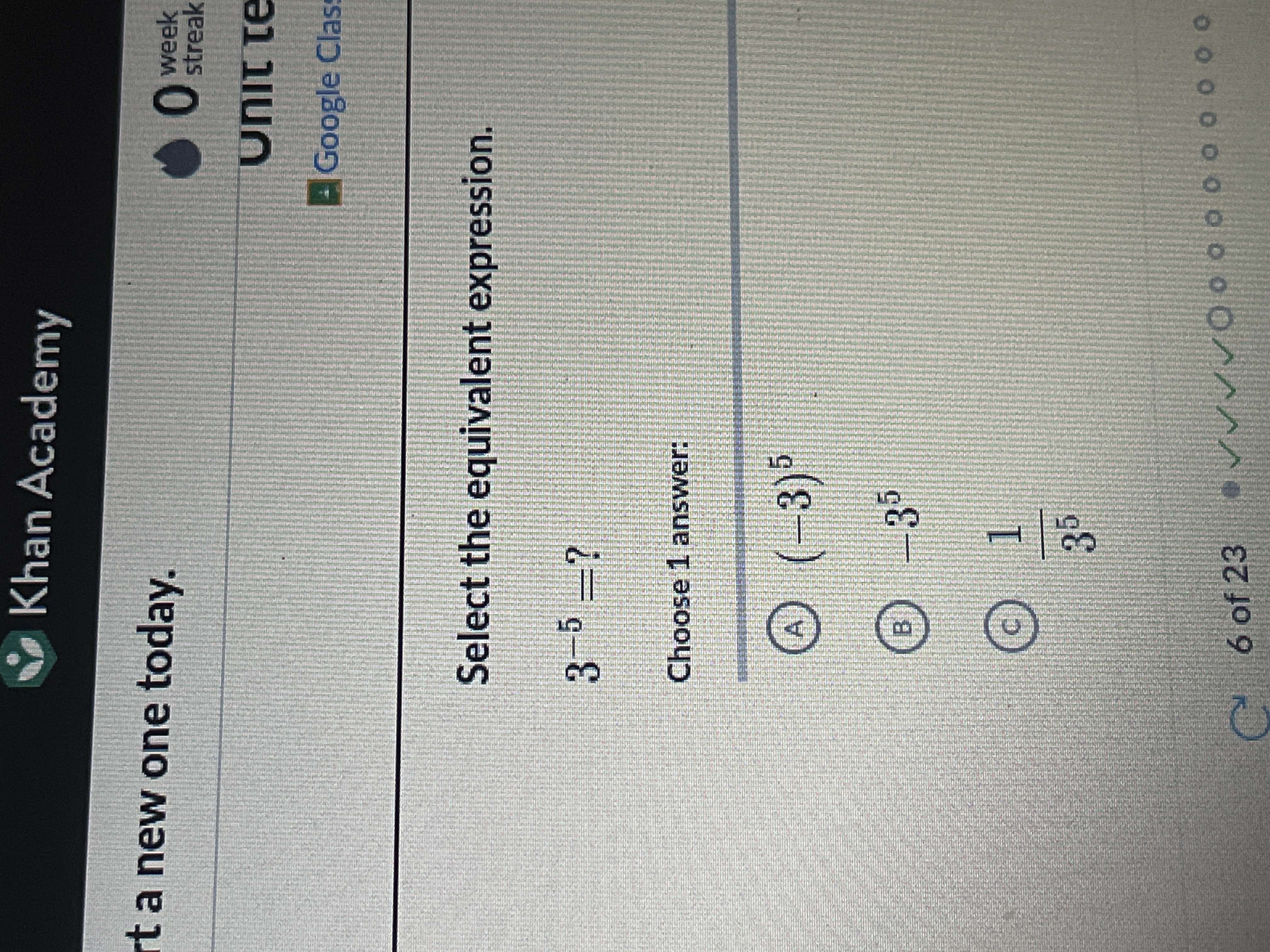
3^-5 = ?
Understand the Problem
The question is asking for an equivalent expression for the exponential expression 3^{-5}. This requires knowledge of exponent rules.
Answer
The equivalent expression is $\frac{1}{3^5}$.
Answer for screen readers
The equivalent expression for $3^{-5}$ is $\frac{1}{3^5}$.
Steps to Solve
- Understanding Negative Exponents
The expression $3^{-5}$ can be rewritten using the rule for negative exponents, which states that $a^{-n} = \frac{1}{a^n}$.
- Applying the Rule
Using the rule, we rewrite $3^{-5}$ as: $$ 3^{-5} = \frac{1}{3^5} $$
- Identifying the Equivalent Expression
Now that we have $3^{-5}$ expressed as $\frac{1}{3^5}$, we can compare this to the choices given in the options to find the equivalent expression.
The equivalent expression for $3^{-5}$ is $\frac{1}{3^5}$.
More Information
The expression $\frac{1}{3^5}$ indicates the reciprocal of $3$ raised to the fifth power. When calculating this, you would compute $3^5 = 243$, so $\frac{1}{3^5} = \frac{1}{243}$.
Tips
Common mistakes include misapplying the negative exponent rule, such as thinking $3^{-5} = -3^5$ instead of $\frac{1}{3^5}$. Always remember that a negative exponent indicates a reciprocal, not a negative sign outside the base.
AI-generated content may contain errors. Please verify critical information