∫ 1/√x dx
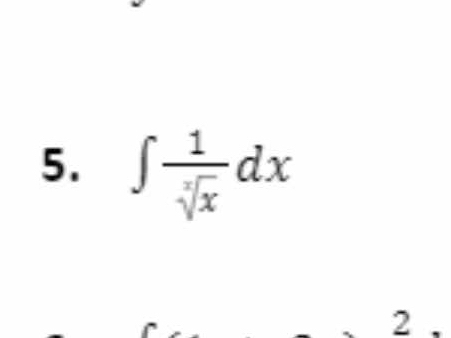
Understand the Problem
The question is asking to evaluate the integral of the function 1/(√x) with respect to x. We will analyze the integral and apply the appropriate rules of integration to find the result.
Answer
$$ 2\sqrt{x} + C $$
Answer for screen readers
$$ \int \frac{1}{\sqrt{x}} , dx = 2\sqrt{x} + C $$
Steps to Solve
- Rewrite the Integral
The integral can be rewritten using an exponent. The expression ( \frac{1}{\sqrt{x}} ) can be expressed as ( x^{-\frac{1}{2}} ).
Thus, the integral becomes: $$ \int x^{-\frac{1}{2}} , dx $$
- Apply the Power Rule of Integration
The power rule states that for any function of the form ( x^n ), the integral is given by: $$ \int x^n , dx = \frac{x^{n+1}}{n+1} + C $$ where ( C ) is the constant of integration and ( n \neq -1 ).
Here, ( n = -\frac{1}{2} ). Therefore, we increase the exponent by 1: $$ -\frac{1}{2} + 1 = \frac{1}{2} $$
Now, apply the formula: $$ \int x^{-\frac{1}{2}} , dx = \frac{x^{\frac{1}{2}}}{\frac{1}{2}} + C $$
- Simplify the Expression
The fraction ( \frac{x^{\frac{1}{2}}}{\frac{1}{2}} ) simplifies to: $$ 2x^{\frac{1}{2}} $$
Putting it all together, we have: $$ \int \frac{1}{\sqrt{x}} , dx = 2\sqrt{x} + C $$
$$ \int \frac{1}{\sqrt{x}} , dx = 2\sqrt{x} + C $$
More Information
This result indicates the area under the curve ( \frac{1}{\sqrt{x}} ) with respect to ( x ). The constant ( C ) represents an arbitrary constant of integration, as integration can yield multiple functions differing by a constant.
Tips
- Forgetting the constant of integration: Always remember to add ( + C ) when integrating.
- Incorrect exponent handling: Make sure the exponent is correctly increased when applying the power rule (i.e., from ( -\frac{1}{2} ) to ( \frac{1}{2} )).
