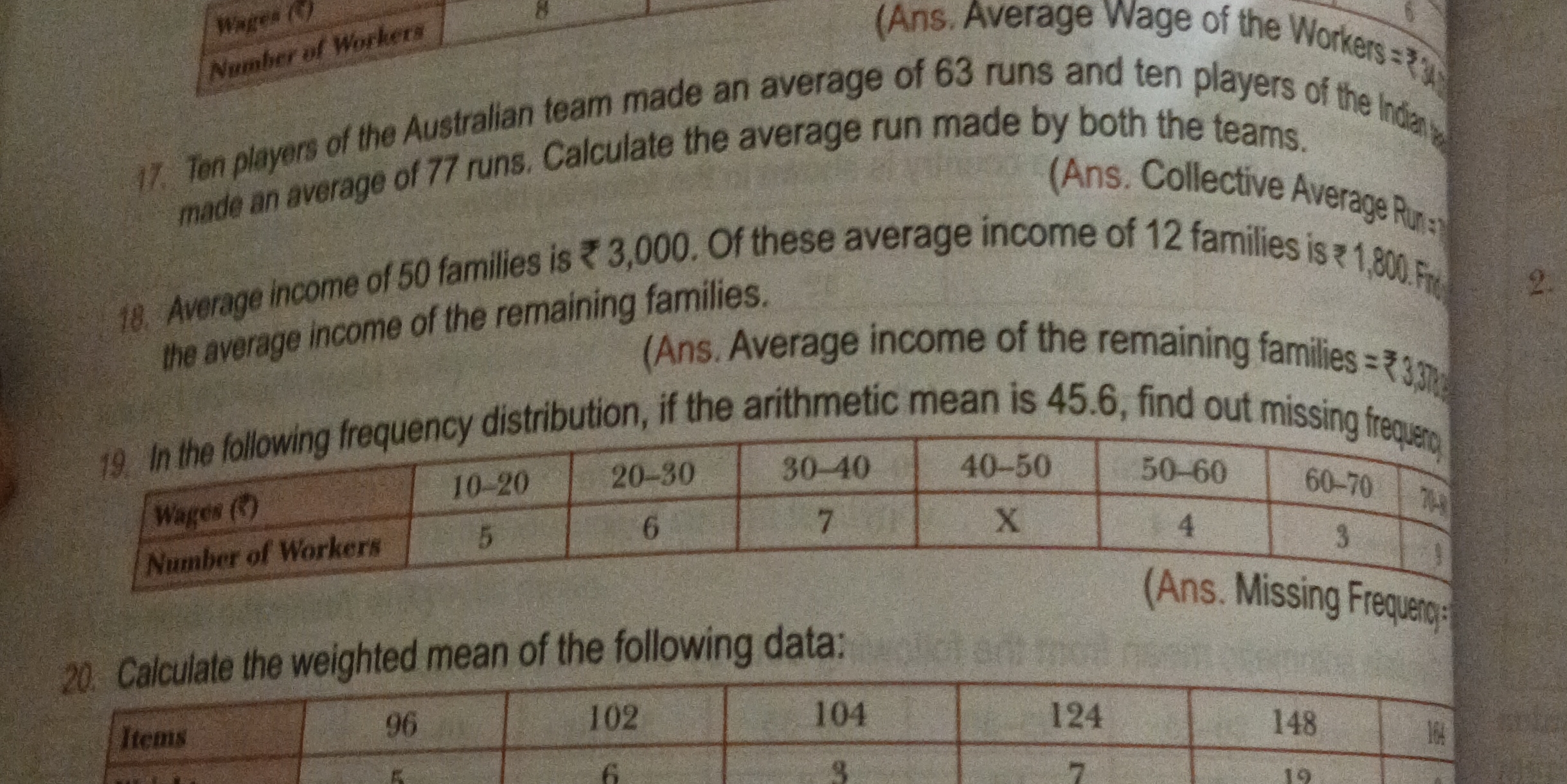
19. In the following frequency distribution, if the arithmetic mean is 45.6, find out missing frequency: Wages: 10-20, 20-30, 30-40, 40-50, 50-60, 60-70 Number of Workers: 5, 6, 7,... 19. In the following frequency distribution, if the arithmetic mean is 45.6, find out missing frequency: Wages: 10-20, 20-30, 30-40, 40-50, 50-60, 60-70 Number of Workers: 5, 6, 7, X, 4, 3 20. Calculate the weighted mean of the following data: Items: 96, 102, 104, 124, 148, 164 Weights: 5, 6, 3, 7, 9, 2 Read more

Steps to Solve

1. Find midpoints of class intervals

The midpoints (x) of the class intervals are: 10-20: $x_1 = (10+20)/2 = 15$ 20-30: $x_2 = (20+30)/2 = 25$ 30-40: $x_3 = (30+40)/2 = 35$ 40-50: $x_4 = (40+50)/2 = 45$ 50-60: $x_5 = (50+60)/2 = 55$ 60-70: $x_6 = (60+70)/2 = 65$

2. Write down the frequencies

The frequencies (f) are: 5, 6, 7, X, 4, 3.

3. Calculate $\sum fx$ expression

$\sum fx = (15 \times 5) + (25 \times 6) + (35 \times 7) + (45 \times X) + (55 \times 4) + (65 \times 3)$ $\sum fx = 75 + 150 + 245 + 45X + 220 + 195$ $\sum fx = 885 + 45X$

4. Calculate $\sum f$ expression

$\sum f = 5 + 6 + 7 + X + 4 + 3$ $\sum f = 25 + X$

5. Use the arithmetic mean formula

The formula for the arithmetic mean is: Mean = $\frac{\sum fx}{\sum f}$ Given the mean is 45.6, we have: $45.6 = \frac{885 + 45X}{25 + X}$

6. Solve for X

Multiply both sides by $(25+X)$: $45.6(25 + X) = 885 + 45X$ $1140 + 45.6X = 885 + 45X$ $45.6X - 45X = 885 - 1140$ $0.6X = -255$ $X = \frac{-255}{0.6} = -425$

7. Solve problem 20. Calculate $\sum{wf}$ and $\sum{f}$ Calculate $\sum{wf}$ $\sum{wf} = (965) + (1026) + (1043) + (1247) + (14812) + (16419) $ $\sum{wf} = 480 + 612 + 312 + 868 + 1776 + 3116 = 7164$ Calculate $\sum{f}$ $\sum{f} = 5 + 6 + 3 + 7 + 12 + 19 = 52$

8. Solve for the weighted mean Weighted mean = $\frac{\sum{wf}}{\sum{f}}$ Weighted mean = $\frac{7164}{52} = 137.769$ Rounded to 2 decimals places: 137.77