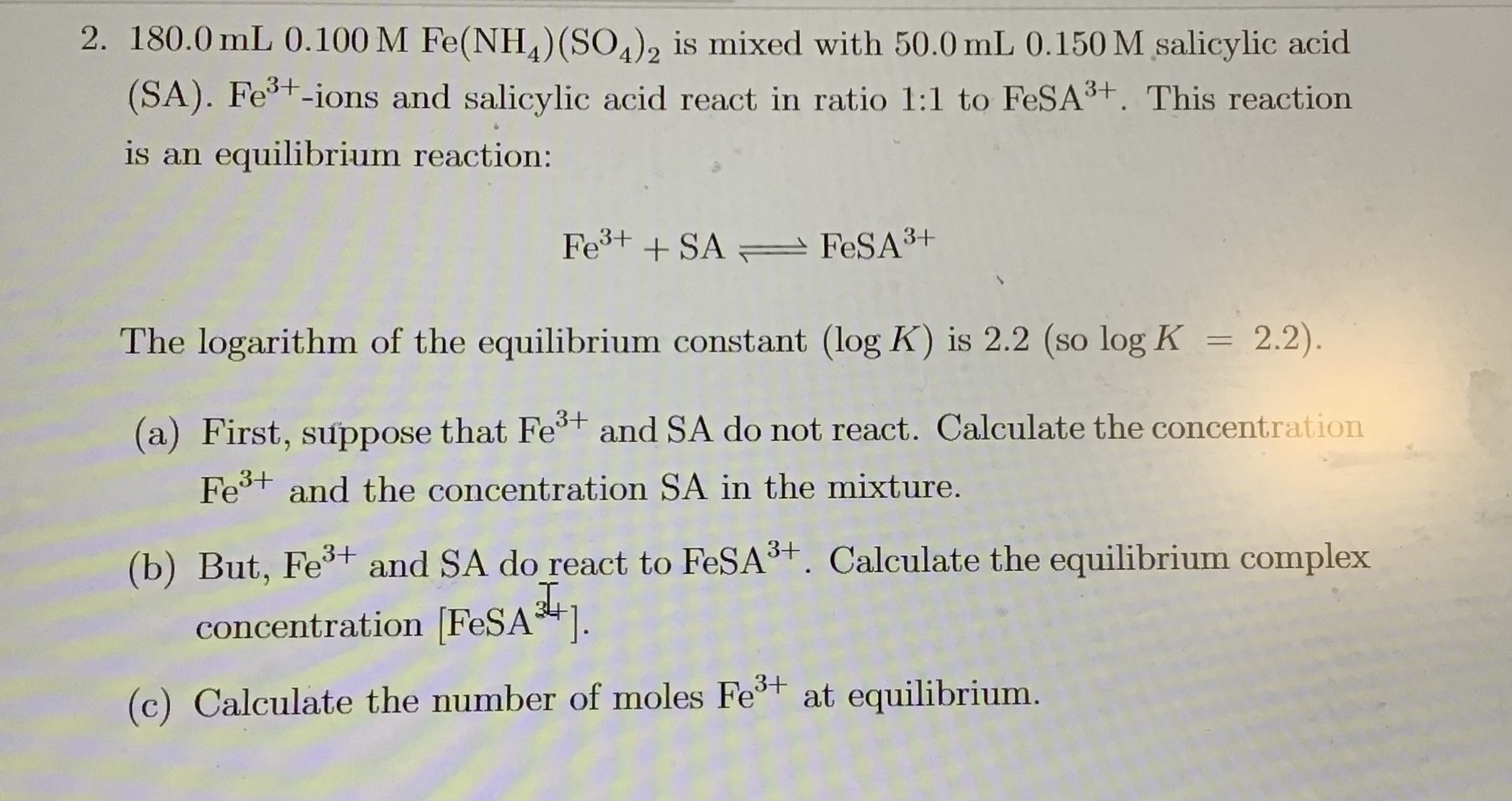
180.0 mL of 0.100 M Fe(NH4)(SO4)2 is mixed with 50.0 mL of 0.150 M salicylic acid (SA). Fe3+ ions and salicylic acid react in a 1:1 ratio to FeSA3+. The reaction is an equilibrium... 180.0 mL of 0.100 M Fe(NH4)(SO4)2 is mixed with 50.0 mL of 0.150 M salicylic acid (SA). Fe3+ ions and salicylic acid react in a 1:1 ratio to FeSA3+. The reaction is an equilibrium reaction: Fe3+ + SA <=> FeSA3+ The logarithm of the equilibrium constant (log K) is 2.2 (so log K = 2.2). (a) First, suppose that Fe3+ and SA do not react. Calculate the concentration of Fe3+ and the concentration of SA in the mixture. (b) But, Fe3+ and SA do react to FeSA3+. Calculate the equilibrium complex concentration [FeSA3+]. (c) Calculate the number of moles of Fe3+ at equilibrium.
Understand the Problem
The question describes a chemical reaction between Fe3+ ions and salicylic acid (SA) to form a complex FeSA3+. The reaction is an equilibrium, and the problem provides the logarithm of the equilibrium constant (log K). The problem is broken down into three parts:
(a) Calculate the initial concentrations of Fe3+ and SA assuming no reaction occurs. (b) Calculate the equilibrium concentration of the FeSA3+ complex, given that the reaction does occur. (c) Calculate the number of moles of Fe3+ at equilibrium.
Answer
(a) $[Fe^{3+}] = 0.0783 \, M$, $[SA] = 0.0326 \, M$ (b) $[FeSA^{3+}] = 0.0326 \, M$ (c) $moles \, Fe^{3+} = 0.0105 \, moles$
Answer for screen readers
(a) $[Fe^{3+}] = 0.0783 , M$ $[SA] = 0.0326 , M$ (b) $[FeSA^{3+}] = 0.0326 , M$ (c) $moles , Fe^{3+} = 0.0105 , moles$
Steps to Solve
- Calculate the initial moles of $Fe^{3+}$
The initial moles of $Fe^{3+}$ can be calculated by multiplying the volume and molarity of the $Fe(NH_4)(SO_4)_2$ solution. Make sure the volume is in Liters.
$moles_{Fe^{3+}} = Volume \times Molarity = 0.180 L \times 0.100 M = 0.018 , moles$
- Calculate the initial moles of $SA$
Similarly, calculate the initial moles of salicylic acid by multiplying the volume and molarity of the SA solution. Make sure the volume is in Liters.
$moles_{SA} = Volume \times Molarity = 0.050 L \times 0.150 M = 0.0075 , moles$
- Calculate the total volume of the mixture
The total volume is the sum of the volumes of the two solutions.
$V_{total} = 180.0 , mL + 50.0 , mL = 230.0 , mL = 0.230 , L$
- Calculate the initial concentrations of $Fe^{3+}$ and $SA$
Divide the number of moles of each species by the total volume to find the initial concentrations.
$[Fe^{3+}]_0 = \frac{0.018 , moles}{0.230 , L} = 0.07826 , M$
$[SA]_0 = \frac{0.0075 , moles}{0.230 , L} = 0.03261 , M$
- Set up an ICE table
To calculate the equilibrium concentration of $FeSA^{3+}$, we'll use an ICE (Initial, Change, Equilibrium) table:
| $Fe^{3+}$ | $SA$ | $FeSA^{3+}$ | |
|---|---|---|---|
| Initial (I) | 0.07826 | 0.03261 | 0 |
| Change (C) | -x | -x | +x |
| Equilibrium (E) | 0.07826-x | 0.03261-x | x |
- Write the expression for the equilibrium constant $K$
Given that $log , K = 2.2$, we can find $K$ by taking the antilog:
$K = 10^{2.2} = 158.49$
The equilibrium constant expression is:
$K = \frac{[FeSA^{3+}]}{[Fe^{3+}][SA]} = \frac{x}{(0.07826 - x)(0.03261 - x)} = 158.49$
- Solve for x
Rearrange the equation to solve for x:
$x = 158.49 \times (0.07826 - x)(0.03261 - x)$
$x = 158.49 \times (0.00255 - 0.07826x - 0.03261x + x^2)$
$x = 0.4048 - 12.39x - 5.17x + 158.49x^2$
$158.49x^2 - 18.56x + 0.4048 = 0$
Solving the quadratic equation using the quadratic formula, we get:
$x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$
$x = \frac{17.56 \pm \sqrt{(-17.56)^2 - 4 \times 158.49 \times 0.4048}}{2 \times 158.49}$
$x = \frac{17.56 \pm \sqrt{308.35 - 256.13}}{316.98}$
$x = \frac{17.56 \pm \sqrt{52.22}}{316.98}$
$x = \frac{17.56 \pm 7.23}{316.98}$
We have two possible values for x:
$x_1 = \frac{17.56 + 7.23}{316.98} = \frac{24.79}{316.98} = 0.0782$ $x_2 = \frac{17.56 - 7.23}{316.98} = \frac{10.33}{316.98} = 0.0326$
Since $x$ cannot be greater than the initial concentration of SA (0.03261 M), we choose the smaller value: $x = 0.0326 , M$
- Calculate the equilibrium concentration of $FeSA^{3+}$
$[FeSA^{3+}] = x = 0.0326 , M$
- Calculate the equilibrium concentrations of $Fe^{3+}$
$[Fe^{3+}]_{eq} = 0.07826 - x = 0.07826 - 0.0326 = 0.04566 , M$
- Calculate the number of moles of $Fe^{3+}$ at equilibrium
$moles_{Fe^{3+}} = [Fe^{3+}]{eq} \times V{total} = 0.04566 \times 0.23 = 0.0105 , moles$
(a) $[Fe^{3+}] = 0.0783 , M$ $[SA] = 0.0326 , M$ (b) $[FeSA^{3+}] = 0.0326 , M$ (c) $moles , Fe^{3+} = 0.0105 , moles$
More Information
The equilibrium strongly favors the formation of the complex, as indicated by the relatively large equilibrium constant ($K = 158.49$). This means that nearly all of the limiting reactant (SA) is converted to the complex $FeSA^{3+}$.
Tips
- Forgetting to convert volumes to liters: A common mistake is to use volumes in milliliters directly in calculations, which leads to incorrect concentrations and moles.
- Choosing the wrong root for x: When solving the quadratic equation, it's important to check if the calculated value of $x$ is physically possible. In this case, $x$ cannot be larger than the initial concentration of the reactants.
- Incorrectly setting up the ICE table: Ensure the change in concentration is represented correctly with the appropriate signs (+ or -).
- Not accounting for the total volume: Failing to calculate the correct concentrations after mixing the two solutions will lead to significant errors.
- Using approximations inappropriately: Approximating when solving for x can be inaccurate if the equilibrium constant is not sufficiently small.
AI-generated content may contain errors. Please verify critical information