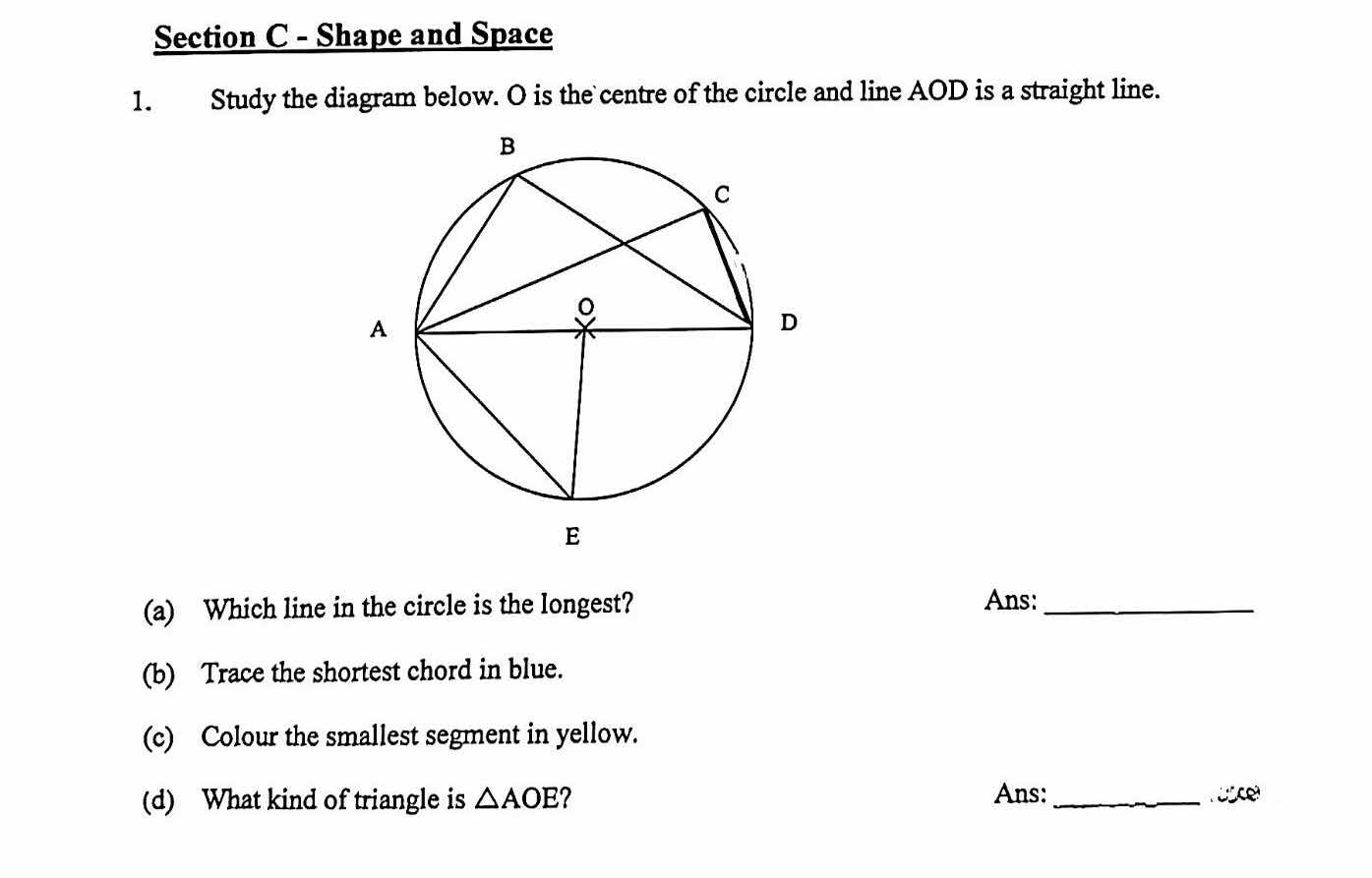
1. Study the diagram below. O is the centre of the circle and line AOD is a straight line. (a) Which line in the circle is the longest? (b) Trace the shortest chord in blue. (c) Co... 1. Study the diagram below. O is the centre of the circle and line AOD is a straight line. (a) Which line in the circle is the longest? (b) Trace the shortest chord in blue. (c) Colour the smallest segment in yellow. (d) What kind of triangle is ΔAOE?
Understand the Problem
The question is asking to study a diagram of a circle and respond to several tasks related to its properties, such as identifying the longest line, tracing a chord, coloring a segment, and determining the type of triangle formed.
Answer
The longest line is $AD$, the shortest chord is $AE$, the smallest segment is the minor segment opposite $AE$, and triangle $AOE$ is isosceles.
Answer for screen readers
(a) The longest line is $AD$.
(b) The shortest chord is $AE$.
(c) The smallest segment is the minor segment opposite chord $AE$.
(d) Triangle $AOE$ is isosceles.
Steps to Solve
-
Identify the Longest Line The longest line in a circle is the diameter. In this diagram, the diameter is the line segment $AD$, as it passes through the center $O$.
-
Determine the Shortest Chord A chord is a line segment whose endpoints lie on the circle. The shortest chord is the line segment $AE$, as it is the perpendicular bisector to the diameter at point $O$.
-
Identify the Smallest Segment The segments within a circle are defined by arcs. The smallest segment formed by the chord $AE$ is the minor segment opposite to this chord, which is the area of the circle between the chord and the circumference.
-
Classify Triangle AOE To classify triangle $AOE$, we check the angles. Since $O$ is the center and $AE$ is a chord, triangle $AOE$ is isosceles (with $OA = OE$) because both are radii of the circle.
(a) The longest line is $AD$.
(b) The shortest chord is $AE$.
(c) The smallest segment is the minor segment opposite chord $AE$.
(d) Triangle $AOE$ is isosceles.
More Information
In a circle, the diameter is always the longest line. Chords are lines connecting points on the circle, and the shortest chord can be found perpendicular to the diameter. The area of segments varies according to their position in relation to the circle's chords.
Tips
- Confusing a chord with a secant line.
- Misidentifying the longest line in a circle (it is always the diameter).
- Forgetting that an isosceles triangle has at least two equal sides, which is true for triangles formed by radii.
AI-generated content may contain errors. Please verify critical information