1. Evaluate the limit of f(x) as x approaches 1 from the left. 2. Identify the type of discontinuity (removable, jump essential, or infinite essential) of g(x) = 1/f(x) at x = -2.... 1. Evaluate the limit of f(x) as x approaches 1 from the left. 2. Identify the type of discontinuity (removable, jump essential, or infinite essential) of g(x) = 1/f(x) at x = -2. 3. True or False: f satisfies the conditions of the Intermediate Value Theorem on the interval [0, 1]. 4. Fill in the blank: The line with equation _____ is an asymptote of f because the limit of f(x) as x approaches positive infinity is 1.
Understand the Problem
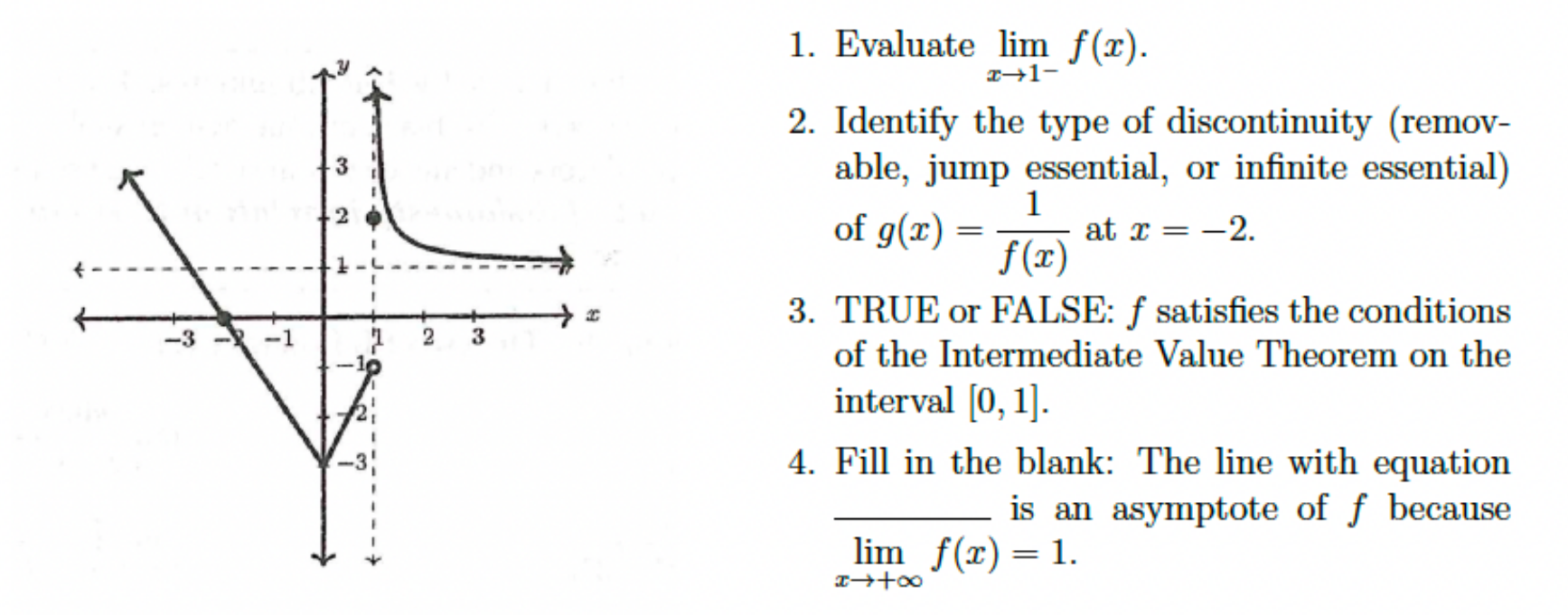
The image presents four calculus questions related to a function represented graphically. The questions involve evaluating a limit, identifying a type of discontinuity, verifying the Intermediate Value Theorem, and determining an asymptote.
Answer
1. -1 2. Infinite essential 3. FALSE 4. $y = 1$
Answer for screen readers
- -1
- Infinite essential
- FALSE
- $y = 1$
Steps to Solve
- Evaluate the limit as $x$ approaches 1 from the left
We need to find the value that $f(x)$ approaches as $x$ gets closer to 1 from values less than 1. Looking at the graph, as $x$ approaches 1 from the left, $f(x)$ approaches -1.
- Identify the discontinuity of $g(x) = \frac{1}{f(x)}$ at $x = -2$
At $x = -2$, the value of $f(x)$ is zero. This means $g(x) = \frac{1}{f(x)} = \frac{1}{0}$, which tends to infinity. Therefore, $g(x)$ has an infinite essential discontinuity at $x = -2$.
- Check if $f$ satisfies the Intermediate Value Theorem on $[0, 1]$
The Intermediate Value Theorem (IVT) requires the function to be continuous on the closed interval $[0, 1]$. From the graph, we can see that $f(x)$ is discontinuous at $x = 1$ as it has a "hole". Therefore, $f$ does not satisfy the conditions of the IVT on $[0, 1]$. The statement is FALSE
- Determine the asymptote of $f$ as $x$ approaches infinity
Given that $\lim_{x \to +\infty} f(x) = 1$, this means that as $x$ goes to positive infinity, the function $f(x)$ approaches 1. This indicates a horizontal asymptote at $y = 1$.
- -1
- Infinite essential
- FALSE
- $y = 1$
More Information
The Intermediate Value Theorem is a key concept in calculus that links the continuity of a function with the values it takes within an interval. The existence of asymptotes helps to describe the end behavior of functions.
Tips
- For the limit, confusing the left-hand limit with the right-hand limit.
- Incorrectly identifying the type of discontinuity due to misinterpreting the behavior of $g(x)$ when $f(x)$ approaches zero.
- Forgetting the continuity requirement for the Intermediate Value Theorem.
- Confusing vertical and horizontal asymptotes.
AI-generated content may contain errors. Please verify critical information