Podcast
Questions and Answers
What does the term $a_i$ represent in the expression $h(τ) = ∑ aᵢδ(τ - τᵢ)$?
What does the term $a_i$ represent in the expression $h(τ) = ∑ aᵢδ(τ - τᵢ)$?
- Values of $τ$ at which the delta function is centered
- The coefficients associated with each delta function (correct)
- A constant value unrelated to the summation
- The total number of terms in the summation
What role does the delta function $δ(τ - τ_i)$ play in the expression?
What role does the delta function $δ(τ - τ_i)$ play in the expression?
- To represent continuous data over $τ$
- To sum all the terms over a continuous range
- To exponentially decay values at certain points
- To provide a way to pick values at discrete points (correct)
If $τ_i$ are distinct values, what happens to $h(τ)$ as the number of terms in the summation increases?
If $τ_i$ are distinct values, what happens to $h(τ)$ as the number of terms in the summation increases?
- It converges to a uniform distribution
- It remains constant regardless of $τ$
- It will show an increasing density of points (correct)
- It becomes a piecewise function with more discontinuities
Which of the following statements is true about the expression $h(τ)$?
Which of the following statements is true about the expression $h(τ)$?
In which scenarios would the expression $h(τ)$ equal zero?
In which scenarios would the expression $h(τ)$ equal zero?
Flashcards
Impulse Train
Impulse Train
A mathematical function that represents a series of impulses, each with a specific weight and location on the time axis.
Dirac Delta Function
Dirac Delta Function
The individual impulses that make up the impulse train, each represented by a Dirac delta function.
Impulse Location
Impulse Location
The location of each impulse in the impulse train, represented by the variable 'τᵢ'.
Impulse Weight
Impulse Weight
Signup and view all the flashcards
Impulse Train Function
Impulse Train Function
Signup and view all the flashcards
Study Notes
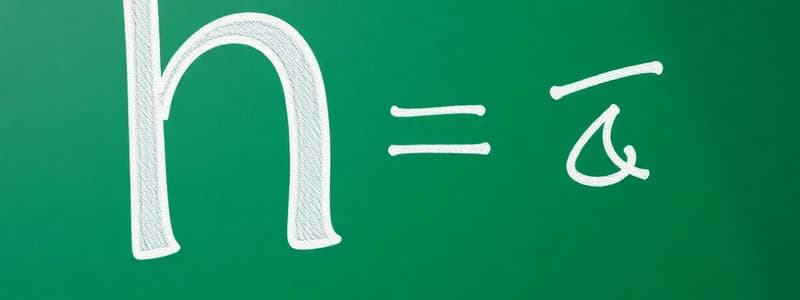
Function h(τ)
- h(τ) is defined as a summation
- The summation is over all values of i
- Each term in the summation is a_iδ(τ - τᵢ)
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.
Description
Explore the function h(τ) defined as a summation over values of i. Understand each term in the summation, specifically how a_i and δ(τ - τᵢ) interact within the function. This quiz will test your comprehension of the concepts involved in this mathematical function.
