Podcast
Questions and Answers
What is the mean (µ) of the random numbers generated by RNG2?
What is the mean (µ) of the random numbers generated by RNG2?
- 20
- 200
- 130 (correct)
- 50
What percentage of numbers generated by RNG2 are less than or equal to 90?
What percentage of numbers generated by RNG2 are less than or equal to 90?
- 99.6%
- 97.7% (correct)
- 95.4%
- 68.2%
Which range is specified for the random numbers generated by RNG2?
Which range is specified for the random numbers generated by RNG2?
- [80 to 130]
- [60 to 200] (correct)
- [1 to 100]
- [50 to 150]
What standard deviation (Std) is used by RNG2 in generating random numbers?
What standard deviation (Std) is used by RNG2 in generating random numbers?
In the context of RNG2, how would you describe the position of the number 80 in relation to the generated numbers?
In the context of RNG2, how would you describe the position of the number 80 in relation to the generated numbers?
What is the expected duration calculated from the values 10 and 20?
What is the expected duration calculated from the values 10 and 20?
What is the formula for variance given a uniform distribution?
What is the formula for variance given a uniform distribution?
What is the probability density function (pdf) for a random variable X that follows a uniform distribution between a and b?
What is the probability density function (pdf) for a random variable X that follows a uniform distribution between a and b?
What percentage of values falls within two standard deviations of the mean in a normal distribution?
What percentage of values falls within two standard deviations of the mean in a normal distribution?
What is the expected value $E[X]$ for a uniform distribution defined between a and b?
What is the expected value $E[X]$ for a uniform distribution defined between a and b?
What does the standard deviation indicate in a normal distribution?
What does the standard deviation indicate in a normal distribution?
Which of the following characteristics is NOT true about the normal distribution?
Which of the following characteristics is NOT true about the normal distribution?
What is the formula for the variance $Var(X)$ of a uniformly distributed random variable?
What is the formula for the variance $Var(X)$ of a uniformly distributed random variable?
Given a task duration uniformly distributed between 10 and 20 days, what is the probability that the task will be completed within 15 days?
Given a task duration uniformly distributed between 10 and 20 days, what is the probability that the task will be completed within 15 days?
What is the standard deviation formula derived from the PERT method?
What is the standard deviation formula derived from the PERT method?
In a normal distribution, how is the mean represented?
In a normal distribution, how is the mean represented?
What is the expected duration of a task uniformly distributed between 10 and 20 days?
What is the expected duration of a task uniformly distributed between 10 and 20 days?
If the duration of a task is 10 to 20 days, what is the probability that it will be completed in 30 days?
If the duration of a task is 10 to 20 days, what is the probability that it will be completed in 30 days?
What does a higher standard deviation signify in relation to a data set?
What does a higher standard deviation signify in relation to a data set?
In modelling a project task duration uniformly between 10 and 20 days, what would be the variance?
In modelling a project task duration uniformly between 10 and 20 days, what would be the variance?
What is the probability of completing the task in less than 5 days given the minimum duration is 10 days?
What is the probability of completing the task in less than 5 days given the minimum duration is 10 days?
What is the main characteristic of a uniform distribution?
What is the main characteristic of a uniform distribution?
Which formula represents the expected value of a uniform distribution?
Which formula represents the expected value of a uniform distribution?
What is the variance formula for a continuous uniform distribution?
What is the variance formula for a continuous uniform distribution?
What describes the probability of a variable taking a value less than a specific value x in a uniform distribution?
What describes the probability of a variable taking a value less than a specific value x in a uniform distribution?
Which of the following illustrates the discrete uniform distribution?
Which of the following illustrates the discrete uniform distribution?
In which type of uniform distribution is the concept of continuous outcomes typically applied in project scheduling?
In which type of uniform distribution is the concept of continuous outcomes typically applied in project scheduling?
What is a common misconception about uniform distribution?
What is a common misconception about uniform distribution?
What probabilistic approach would lead to biased estimation in project duration allocation?
What probabilistic approach would lead to biased estimation in project duration allocation?
What statistical distribution is introduced in Week 05?
What statistical distribution is introduced in Week 05?
What method is covered in Week 06 following the Probabilistic Approach to Project Network Diagram?
What method is covered in Week 06 following the Probabilistic Approach to Project Network Diagram?
In which week is the Knowledge Test scheduled?
In which week is the Knowledge Test scheduled?
Which of these components is weighted the heaviest in the assessments?
Which of these components is weighted the heaviest in the assessments?
Which approach is deemed suitable for simple projects as per the overview?
Which approach is deemed suitable for simple projects as per the overview?
What is a topic discussed in Week 05 alongside Uniform Distribution?
What is a topic discussed in Week 05 alongside Uniform Distribution?
When is the Group Assignment Presentation due?
When is the Group Assignment Presentation due?
What type of analysis is introduced in Week 11?
What type of analysis is introduced in Week 11?
What is the estimated duration for the task if the company can tolerate up to 15.9% risk?
What is the estimated duration for the task if the company can tolerate up to 15.9% risk?
When the company cannot afford a risk over 2.3%, what is the estimated duration for the task?
When the company cannot afford a risk over 2.3%, what is the estimated duration for the task?
What is the mean (μ) duration of the task in the project?
What is the mean (μ) duration of the task in the project?
What does a standard deviation (σ) of 2 days indicate in the context of task duration?
What does a standard deviation (σ) of 2 days indicate in the context of task duration?
If a company tolerates a 30% risk, can the estimated duration be found using the same method?
If a company tolerates a 30% risk, can the estimated duration be found using the same method?
How do you calculate a Z-score?
How do you calculate a Z-score?
If a student scores 16 out of 20 and another scores 30 out of 40, which method provides a fair comparison?
If a student scores 16 out of 20 and another scores 30 out of 40, which method provides a fair comparison?
What is the standard deviation (σ) value in the task's duration distribution?
What is the standard deviation (σ) value in the task's duration distribution?
Flashcards
Probabilistic Project Network Diagram
Probabilistic Project Network Diagram
A project network diagram that considers the uncertainty in task durations, using probability distributions like uniform and normal distributions.
Uniform Distribution
Uniform Distribution
A probability distribution where all values within a specific range are equally likely.
Normal Distribution
Normal Distribution
A bell-shaped probability distribution where the mean (average), median, and mode are equal.
Z-score
Z-score
Signup and view all the flashcards
Z-table
Z-table
Signup and view all the flashcards
Project Variance
Project Variance
Signup and view all the flashcards
Project Standard Deviation
Project Standard Deviation
Signup and view all the flashcards
Deterministic Approach
Deterministic Approach
Signup and view all the flashcards
Expected Value (Uniform)
Expected Value (Uniform)
Signup and view all the flashcards
Variance (Uniform)
Variance (Uniform)
Signup and view all the flashcards
Probability (Uniform)
Probability (Uniform)
Signup and view all the flashcards
Continuous Uniform Distribution
Continuous Uniform Distribution
Signup and view all the flashcards
Discrete Uniform Distribution
Discrete Uniform Distribution
Signup and view all the flashcards
Minimum Value (a)
Minimum Value (a)
Signup and view all the flashcards
Maximum Value (b)
Maximum Value (b)
Signup and view all the flashcards
Expected Duration
Expected Duration
Signup and view all the flashcards
Variance
Variance
Signup and view all the flashcards
Standard Deviation
Standard Deviation
Signup and view all the flashcards
PERT
PERT
Signup and view all the flashcards
Mean (μ)
Mean (μ)
Signup and view all the flashcards
Standard Deviation (σ)
Standard Deviation (σ)
Signup and view all the flashcards
Compare RNG outputs
Compare RNG outputs
Signup and view all the flashcards
How to compare RNG outputs?
How to compare RNG outputs?
Signup and view all the flashcards
Uniform Distribution PDF
Uniform Distribution PDF
Signup and view all the flashcards
Expected Value (E[X])
Expected Value (E[X])
Signup and view all the flashcards
Expected Value (E[X^2])
Expected Value (E[X^2])
Signup and view all the flashcards
Variance (Var[X])
Variance (Var[X])
Signup and view all the flashcards
Uniform distribution's range
Uniform distribution's range
Signup and view all the flashcards
Expected duration of a task
Expected duration of a task
Signup and view all the flashcards
Variance of task duration
Variance of task duration
Signup and view all the flashcards
Probability of task completion in a given timeframe
Probability of task completion in a given timeframe
Signup and view all the flashcards
Project Task Duration
Project Task Duration
Signup and view all the flashcards
Risk Tolerance
Risk Tolerance
Signup and view all the flashcards
Estimated Duration
Estimated Duration
Signup and view all the flashcards
Study Notes
PMGT3623 Scheduling - Week 5
- Topic: Probabilistic Approach to Project Network Diagram - Part II
- Topics Covered: Uniform Distribution, Normal Distribution (Z-score and Z-table), Project Variance and Standard Deviation
- Uniform Distribution: Possible outcomes are equally likely. All outcomes in a specified range have an equal chance of occurring. A simple example is rolling a fair die. The probability of any score is 1/6. There are discrete and continuous uniform distributions; in project scheduling, the focus is continuous.
- Expected Value (E): For a variable uniformly distributed between a minimum value (a) and a maximum value (b), the expected value is (a + b) / 2.
- Variance (Var): For a uniform distribution, variance is (b - a)² / 12.
- Probability (P(x ≤ X)): The probability of a variable taking a value less than a specific value (x) is (x - a) / (b - a), where a ≤ x ≤ b.
- Probability Density Function (pdf): The pdf of a uniformly distributed random variable X follows f(x) = 1/(b-a) for a ≤ x ≤ b.
- Project Variance and Standard Deviation (PERT):
- Standard deviation (σ) = (P - O) / 6
- Variance = (P - O)² / 36
- Where P = Optimistic time, O=Pessimistic time
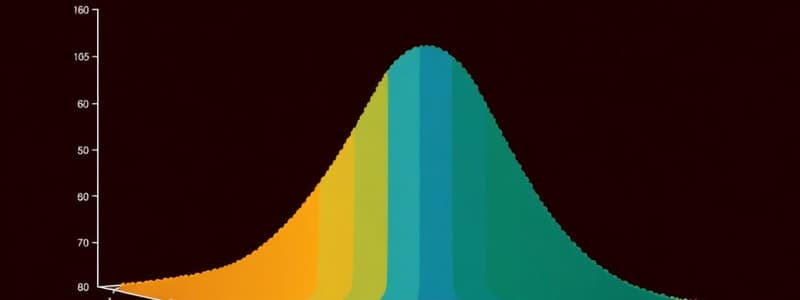
- Normal Distribution: Often called the bell curve. Most values cluster around the mean. Frequency of values reduces symmetrically away from the mean. Defined by mean (μ) and standard deviation (σ). Mean defines the center of the distribution; standard deviation determines its spread.
- Standard Deviation: Measures how much members of a group differ from the mean value.
Quick Overview (Last Two Weeks)
- Deterministic vs. Probabilistic Approaches: A deterministic approach to task duration is suitable for simple projects. In complex projects, a probabilistic approach is more accurate as it accounts for the variability in task durations. A deterministic approach often leads to biased estimation.
- Probability Theory: Basic concepts were covered allowing for more accurate estimation.
- Probabilistic Estimation Techniques: Expected Value and PERT (Program Evaluation and Review Technique)
- PERT Complexity: PERT is more complex than Expected Value and uses the Beta distribution.
- Emphasized Topics for this week: Uniform distribution, Normal distribution, and Z-score concepts.
Additional Exercises and Examples
- Real-world example: Example involving a project with a single task (Task A) with duration uniformly distributed between 10 and 20 days. Calculation of expected duration, variance, and probability of completing the task within specific timeframes.
- Importance of Z-scores: How to use Z-scores to compare values from different normal distributions. Converting raw values to standard units for comparison.
- Z-table: A table providing probabilities corresponding to different Z-scores, used for converting Z-scores to percentages and vice-versa.
- Project Variance and Standard Deviation Exercise: Examples and calculation of the expected time, variance and standard deviation for single-task projects. Determining the probability for differing project completion timings.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.
Related Documents
Description
This quiz covers the probabilistic approach to project network diagrams, focusing on uniform and normal distributions. Key concepts include expected value, variance, and how to calculate probabilities using the uniform distribution. Test your understanding of these essential statistical methods used in project scheduling.
