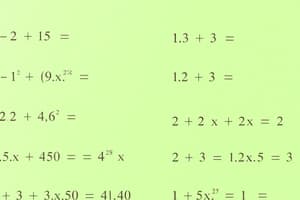Podcast
Questions and Answers
Which represents the solution set of $5(x + 5) < 85$?
Which represents the solution set of $5(x + 5) < 85$?
x < 12
A taxi service charges a flat fee of $1.25 and $0.75 per mile. If Henri has $14.00, which of the following shows the number of miles he can afford to ride in the taxi?
A taxi service charges a flat fee of $1.25 and $0.75 per mile. If Henri has $14.00, which of the following shows the number of miles he can afford to ride in the taxi?
- 16 miles (correct)
- 18 miles
- 20 miles
- All of the above
Which graph shows the solution set of the inequality $2.9(x + 8) < 26.1$?
Which graph shows the solution set of the inequality $2.9(x + 8) < 26.1$?
- Graph C
- Graph B
- Graph A
- Graph D (correct)
For what number of hours does Grayson charge more than Ian if $h$ represents the number of hours?
For what number of hours does Grayson charge more than Ian if $h$ represents the number of hours?
Flashcards
Solution to 5(x + 5) < 85
Solution to 5(x + 5) < 85
x is less than 12. This is the range of values for which the inequality 5(x + 5) < 85 is true.
Taxi ride affordability
Taxi ride affordability
Henri can afford to ride 16 miles in the taxi with $14.00, considering the flat fee and per-mile charge.
Solution to 2.9(x + 8) < 26.1
Solution to 2.9(x + 8) < 26.1
The solution set represents all values of x that satisfy the inequality. Solve for x to find the range.
What 'h' represents
What 'h' represents
Signup and view all the flashcards
Study Notes
Inequalities Overview
- Solution set for the inequality 5(x + 5) < 85 simplifies to x < 12.
- Represents a linear inequality indicating all values less than 12.
Taxi Service Fare Calculation
- Taxi service has a base charge of $1.25 plus $0.75 for each mile.
- Henri has a total budget of $14.00 for taxi fare.
- To determine the maximum miles he can afford, the inequality to solve would involve the flat fee and cost per mile.
Graphing Inequalities
- The solution set of the inequality 2.9(x + 8) < 26.1 must be represented on a graph.
- Visual representation helps in understanding the range of values that satisfy the inequality (option D indicates the correct graph).
Comparison of Tax Preparation Costs
- Grayson charges $35 per hour plus a $35 administration fee.
- Ian charges $45 per hour with a $15 administration fee.
- To find when Grayson becomes more expensive than Ian, set up the inequality involving hours (h) worked and solve for h.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.