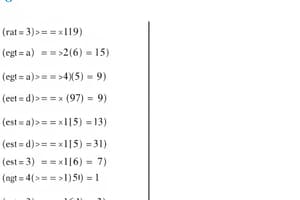Podcast
Questions and Answers
What can be said about the set L(A) of all linear combinations of elements of a set A?
What can be said about the set L(A) of all linear combinations of elements of a set A?
- It is a subspace. (correct)
- It is not a subspace.
- It is only closed under addition.
- It is not closed under addition and multiplication by scalars.
Why does L(A) include each ai?
Why does L(A) include each ai?
- Because L(A) contains all linear combinations of elements of A. (correct)
- Because L(A) is closed under addition.
- Because L(A) is closed under multiplication by scalars.
- Because L(A) is a subspace.
What can be said about a subspace W that includes A?
What can be said about a subspace W that includes A?
- It contains each linear combination L Xiai. (correct)
- It does not contain each linear combination L Xiai.
- It cannot contain any linear combinations L Xiai.
- It only contains some linear combinations L Xiai.
How can a sum of two linear combinations of elements of A be rewritten?
How can a sum of two linear combinations of elements of A be rewritten?
What does it mean for a set A to span a vector space V?
What does it mean for a set A to span a vector space V?
Is the function space on an infinite set A always finite-dimensional?
Is the function space on an infinite set A always finite-dimensional?
What is the space of all mappings f: A -> W?
What is the space of all mappings f: A -> W?
What is Hom(V, W)?
What is Hom(V, W)?
What is the theorem 3.2 stating?
What is the theorem 3.2 stating?
Why is Hom(V, W) a subspace?
Why is Hom(V, W) a subspace?
What is the theorem 3.3 stating?
What is the theorem 3.3 stating?
What is the result of the composition of linear maps?
What is the result of the composition of linear maps?
What is the value of L~ at t, given the coefficients xi and functions Ii?
What is the value of L~ at t, given the coefficients xi and functions Ii?
What is the image of ~1 under the linear mapping T?
What is the image of ~1 under the linear mapping T?
What is the set of all real-valued functions on IR?
What is the set of all real-valued functions on IR?
What is the theorem that states that every linear mapping from IRn to W is of a certain form?
What is the theorem that states that every linear mapping from IRn to W is of a certain form?
What is the coefficient of et in the linear combination L~?
What is the coefficient of et in the linear combination L~?
What is the spanning set for IRn?
What is the spanning set for IRn?
What is the property of the linear combination map T?
What is the property of the linear combination map T?
What is the result of T(x + y) in terms of T(x) and T(y)?
What is the result of T(x + y) in terms of T(x) and T(y)?
What is the significance of the theorem in this context?
What is the significance of the theorem in this context?
What is the notation La for?
What is the notation La for?
What is the skeleton of a linear mapping T?
What is the skeleton of a linear mapping T?
What is the relationship between the map a -> La and the set of all linear maps T from IR to W?
What is the relationship between the map a -> La and the set of all linear maps T from IR to W?
What is the topic of Chapter 3 in the table of contents?
What is the topic of Chapter 3 in the table of contents?
What is the title of the chapter that deals with 'Bases Dimension The dual space'?
What is the title of the chapter that deals with 'Bases Dimension The dual space'?
What comes after 'Chapter 3' in the table of contents?
What comes after 'Chapter 3' in the table of contents?
What is the topic of the chapter marked with a *?
What is the topic of the chapter marked with a *?
How many chapters are there in the table of contents?
How many chapters are there in the table of contents?
Flashcards are hidden until you start studying