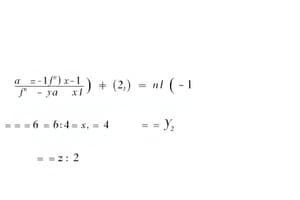Podcast
Questions and Answers
When evaluating a definite integral as the limit of a sum, what does 'f(x)' represent?
When evaluating a definite integral as the limit of a sum, what does 'f(x)' represent?
- The value of the definite integral
- The number of intervals
- The width of the intervals
- A continuous real valued function (correct)
If an interval [a, b] is divided into 'n' equal parts of width 'h', what does 'h' represent?
If an interval [a, b] is divided into 'n' equal parts of width 'h', what does 'h' represent?
- The number of subdivisions
- The width of the intervals (correct)
- The value of the definite integral
- The number of intervals
In the context of evaluating definite integrals as limits of sums, what does 'n' represent?
In the context of evaluating definite integrals as limits of sums, what does 'n' represent?
- The value of the definite integral
- The number of intervals
- The width of the intervals
- The number of subdivisions (correct)
What is being evaluated when considering definite integral as the limit of a sum?
What is being evaluated when considering definite integral as the limit of a sum?
What happens to the accuracy of the evaluation as the number of subdivisions 'n' increases?
What happens to the accuracy of the evaluation as the number of subdivisions 'n' increases?
In the context of evaluating definite integrals as limits of sums, what does 'h' represent?
In the context of evaluating definite integrals as limits of sums, what does 'h' represent?
What does 'n' represent when an interval [a, b] is divided into 'n' equal parts of width 'h'?
What does 'n' represent when an interval [a, b] is divided into 'n' equal parts of width 'h'?
When evaluating definite integrals as limits of sums, what is the significance of increasing the number of subdivisions 'n'?
When evaluating definite integrals as limits of sums, what is the significance of increasing the number of subdivisions 'n'?
What is being evaluated when considering definite integral as the limit of a sum?
What is being evaluated when considering definite integral as the limit of a sum?
In the context of evaluating definite integrals as limits of sums, what does 'f(x)' represent?
In the context of evaluating definite integrals as limits of sums, what does 'f(x)' represent?
In the context of evaluating definite integrals as limits of sums, what does 'h' represent?
In the context of evaluating definite integrals as limits of sums, what does 'h' represent?
What is the significance of increasing the number of subdivisions 'n' when evaluating a definite integral as the limit of a sum?
What is the significance of increasing the number of subdivisions 'n' when evaluating a definite integral as the limit of a sum?
What does 'n' represent when an interval [a, b] is divided into 'n' equal parts of width 'h'?
What does 'n' represent when an interval [a, b] is divided into 'n' equal parts of width 'h'?
When considering definite integral as the limit of a sum, what does 'f(x)' represent?
When considering definite integral as the limit of a sum, what does 'f(x)' represent?
What happens to the accuracy of the evaluation as the number of subdivisions 'n' increases?
What happens to the accuracy of the evaluation as the number of subdivisions 'n' increases?
Flashcards are hidden until you start studying
Study Notes
Evaluating Definite Integrals as Limits of Sums
- 'f(x)' represents the function being integrated.
- When an interval [a, b] is divided into 'n' equal parts, 'h' represents the width of each part, given by (b-a)/n.
- 'n' represents the number of equal parts or subdivisions of the interval [a, b].
- When evaluating a definite integral as the limit of a sum, the area under the curve of the function f(x) between points a and b is being evaluated.
- As the number of subdivisions 'n' increases, the accuracy of the evaluation also increases, providing a better approximation of the area under the curve.
- Increasing the number of subdivisions 'n' improves the accuracy of the evaluation by providing more precise sums and reducing the width of each part, allowing for a more accurate estimation of the area under the curve.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.