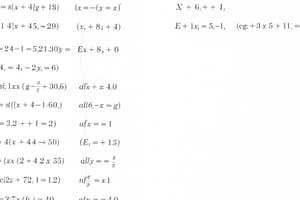Podcast
Questions and Answers
What is a function?
What is a function?
A relation in which every input has exactly one output.
What is domain?
What is domain?
The set of all possible inputs for a function
What is range?
What is range?
The set of all possible outputs for a function.
An open circle includes the point.
An open circle includes the point.
A closed circle includes the point
A closed circle includes the point
What does the () mean in interval notation?
What does the () mean in interval notation?
What does the [] mean in interval notation?
What does the [] mean in interval notation?
What does the U mean in interval notation?
What does the U mean in interval notation?
Is this equation in interval or inequality notation? - (2,4)U[7,9)
Is this equation in interval or inequality notation? - (2,4)U[7,9)
When using interval notation you always write your answers from _____ to ________.
When using interval notation you always write your answers from _____ to ________.
Linear functions must be ______.
Linear functions must be ______.
Match the formula to its form
Match the formula to its form
When is point slope form best to use?
When is point slope form best to use?
When is slope intercept form best to use?
When is slope intercept form best to use?
When is standard form best to use?
When is standard form best to use?
How would you rearrange the equation 3x + 5y = 10 to solve for y?
How would you rearrange the equation 3x + 5y = 10 to solve for y?
Identify the slope and y-intercept of the line given by the equation y = -1/3x - 6.
Identify the slope and y-intercept of the line given by the equation y = -1/3x - 6.
What is the standard form of the equation for the line with slope -2 passing through the point (-4, -1)?
What is the standard form of the equation for the line with slope -2 passing through the point (-4, -1)?
For the equation y - 6 = -(x + 2), identify the slope and the point it passes through.
For the equation y - 6 = -(x + 2), identify the slope and the point it passes through.
What is the relationship between the equations y = -4 and 3x + 5y = 10 when graphed?
What is the relationship between the equations y = -4 and 3x + 5y = 10 when graphed?
What is the slope of the line that passes through the points (-1,4) and (1,6)?
What is the slope of the line that passes through the points (-1,4) and (1,6)?
Write the equation of a line with an x-intercept of 3 and a y-intercept of -2.
Write the equation of a line with an x-intercept of 3 and a y-intercept of -2.
What is the equation of the line with a slope of 2 that passes through the point (2,7)?
What is the equation of the line with a slope of 2 that passes through the point (2,7)?
Determine the slope and y-intercept of the line with the equation $6y + 10 = 3x$.
Determine the slope and y-intercept of the line with the equation $6y + 10 = 3x$.
Create the equation of a line that has the slope of the line $6y + 10 = 3x$ and the y-intercept of 4.
Create the equation of a line that has the slope of the line $6y + 10 = 3x$ and the y-intercept of 4.
Find the domain and range of the following graph using interval notation.
Find the domain and range of the following graph using interval notation.
Convert (-2,3] from interval notation to inequality notation
Convert (-2,3] from interval notation to inequality notation
Find the domain and range using interval notation.
Find the domain and range using interval notation.
Find the domain and range using interval notation.
Find the domain and range using interval notation.
Follow the image.
Follow the image.
Point slope form:
Point slope form:
Slope intercept form
Slope intercept form
Standard form (A = reciprocal of x-intercept, vice versa)
Standard form (A = reciprocal of x-intercept, vice versa)
Convert y=-5x+21 into standard form.
Convert y=-5x+21 into standard form.
Write the particular equation of the line that contains (3,7) and has a slope of 11.
Write the particular equation of the line that contains (3,7) and has a slope of 11.
Convert y-7=11(x-3) into standard form.
Convert y-7=11(x-3) into standard form.
write the particular equation of the line that contains (-2,-3) and has a slope of 3/5
write the particular equation of the line that contains (-2,-3) and has a slope of 3/5
Find domain and range using interval notation
Find domain and range using interval notation
Describe the equation of parallel lines.
Describe the equation of parallel lines.
Describe the equation of perpendicular lines
Describe the equation of perpendicular lines
What is a perpendicular bisector?
What is a perpendicular bisector?
What is the perpendicular bisector formula?
What is the perpendicular bisector formula?
Write the particular equation of the line that contains (4,1) and is perpendicular to the graph of 5x-7y=44
Write the particular equation of the line that contains (4,1) and is perpendicular to the graph of 5x-7y=44
What is the value of A that would make the lines 6x-8y=11 and Ax+4y=11 parallel?
What is the value of A that would make the lines 6x-8y=11 and Ax+4y=11 parallel?
Find the value of k so that 6y=kx+10 and 1/5y=1/10x-2 are parallel
Find the value of k so that 6y=kx+10 and 1/5y=1/10x-2 are parallel
Find the value of A so that would make the lines 4x-3y=10 and Ax+4y=7 perpendicular.
Find the value of A so that would make the lines 4x-3y=10 and Ax+4y=7 perpendicular.
Write an equation of the perpendicular bisector of the segment that joins the point (4,-8) and (10,12)
Write an equation of the perpendicular bisector of the segment that joins the point (4,-8) and (10,12)
Flashcards are hidden until you start studying
Study Notes
Lines and Slopes
- To find the equation of a line passing through two points, use the slope formula ( m = \frac{y_2 - y_1}{x_2 - x_1} ).
- The points (-1, 4) and (1, 6) give a slope of ( m = \frac{6 - 4}{1 - (-1)} = \frac{2}{2} = 1 ).
- The equation can be expressed in point-slope form: ( y - y_1 = m(x - x_1) ), leading to ( y - 4 = 1(x + 1) ) or simplified to ( y = x + 5 ).
x-intercept and y-intercept
- A line's x-intercept is where it crosses the x-axis (y=0); the given x-intercept is 3, so the point is (3,0).
- A line's y-intercept is where it crosses the y-axis (x=0); the given y-intercept is -2, so the point is (0,-2).
- Using intercepts, the line can be expressed with the intercept form: ( \frac{x}{3} + \frac{y}{-2} = 1 ), or simplified to ( 2x + 3y = 6 ).
Point-Slope Form
- A line with a slope of 2 and passing through (2, 7) can be derived from the point-slope formula.
- The slope is defined as ( m = 2 ) and the line equation is ( y - 7 = 2(x - 2) ).
- Simplifying results in the equation: ( y = 2x + 3 ).
Honors Problem
- The equation ( 6y + 10 = 3x ) can be rearranged to find its slope and y-intercept.
- Rearranging gives standard form ( y = \frac{1}{2}x - \frac{5}{3} ); thus, the slope is ( \frac{1}{2} ) and the y-intercept is ( -\frac{5}{3} ).
- Creating a new line requires maintaining the same slope ( \frac{1}{2} ) but changing the y-intercept to 4: the new line equation is ( y = \frac{1}{2}x + 4 ).
Graph Analysis of Line Segments
- The line has two marked points: (-2, 2) and (2, 0).
- The line extends indefinitely to the left due to an arrow at the end.
- The line continues indefinitely to the right, also indicated by an arrow.
Domain and Range
-
Domain: Represents all possible x-values of the line, expressed in interval notation as (−∞,1)∪[2,∞)(-\infty, 1) \cup [2, \infty)(−∞,1)∪[2,∞).
- Includes all x-values less than 1, and all x-values from 2 onward.
- The interval notation indicates that 1 is excluded while 2 is included.
-
Range: Represents all possible y-values of the line, which is noted as (−∞,0]∪∪(-\infty, 0] \cup \cup(−∞,0]∪∪ (incomplete).
- Includes all y-values up to 0; specific upper limit needs clarification.
- Indicates that the value of y cannot exceed 0.
Important Considerations
- The inclusion of arrows signifies that the line continues past the marked points.
- Interval notation is essential in determining boundaries and the nature of inclusivity/exclusivity of endpoints.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.