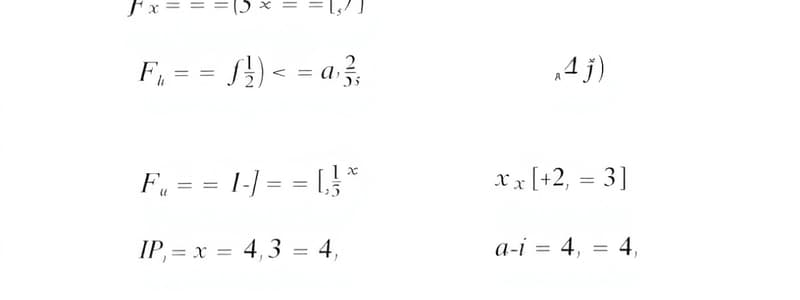Algebraic Equations Explained

• Algebraic Equations

  • Algebraic equations involve variables, constants, and mathematical operations, aiming to find the value(s) of the variable(s) that satisfy the equation.
  • A linear equation is a type of algebraic equation where the highest power of the variable is 1; it can be written in the form ax + b = 0, where a and b are constants.
  • Solving algebraic equations involves isolating the variable on one side of the equation by performing the same operations on both sides to maintain equality.
  • The order of operations (PEMDAS/BODMAS) is crucial when simplifying expressions within equations: Parentheses/Brackets, Exponents/Orders, Multiplication and Division, Addition and Subtraction.
  • Equations can have one solution, no solution (inconsistent), or infinitely many solutions (identity).
  • The distributive property, a(b + c) = ab + ac, is often used to eliminate parentheses when solving equations.
  • Combining like terms simplifies equations by grouping terms with the same variable and exponent.
  • To solve equations with fractions, multiplying both sides by the least common denominator (LCD) can eliminate the fractions.
  • Cross-multiplication is a shortcut for solving proportions (equations with two ratios).
  • Checking the solution by substituting it back into the original equation is essential to ensure accuracy.
  • Some equations may require factoring to solve, especially quadratic equations.
  • Word problems can be translated into algebraic equations by identifying key information and assigning variables appropriately.
  • Graphing linear equations can visually represent the solution set.
  • Systems of linear equations involve two or more equations with the same variables; solutions can be found through substitution, elimination, or graphing.
  • Inequalities are similar to equations but use inequality symbols (>, <, ≥, ≤); solving them involves similar techniques, but multiplying or dividing by a negative number reverses the inequality sign.
  • Absolute value equations require considering both positive and negative cases for the expression inside the absolute value.

• Pythagorean Theorem

  • The Pythagorean Theorem applies to right-angled triangles only.
  • It states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides (legs).
  • Mathematically, the theorem is expressed as a² + b² = c², where 'a' and 'b' are the lengths of the legs, and 'c' is the length of the hypotenuse.
  • The hypotenuse is always the longest side of the right-angled triangle.
  • The theorem can be used to find the length of an unknown side of a right-angled triangle if the lengths of the other two sides are known.
  • To find the hypotenuse (c), calculate c = √(a² + b²).
  • To find a leg (a or b), rearrange the formula: a = √(c² - b²) or b = √(c² - a²).
  • Pythagorean triples are sets of three positive integers that satisfy the Pythagorean Theorem (e.g., 3, 4, 5).
  • The converse of the Pythagorean Theorem states that if a² + b² = c² holds true for the sides of a triangle, then the triangle is a right-angled triangle.
  • The Pythagorean Theorem is used in various real-world applications, including construction, navigation, and surveying.
  • When solving problems, it is crucial to correctly identify the hypotenuse and the legs of the right-angled triangle.
  • Units of measurement (e.g., cm, m, inches) must be consistent when applying the theorem.
  • The theorem can be used to determine if a triangle is acute (c² < a² + b²) or obtuse (c² > a² + b²).
  • Problems involving the theorem may require simplifying square roots.
  • The theorem can be extended to three dimensions, relating the diagonal of a rectangular prism to its length, width, and height.

Cell Biology

• Basic Cell Structure
  • Cells are the basic structural and functional units of all known living organisms.
  • The cell theory states that all living organisms are composed of one or more cells, the cell is the basic unit of life, and all cells arise from pre-existing cells.
  • There are two main types of cells: prokaryotic and eukaryotic.
  • Prokaryotic cells lack a nucleus and other membrane-bound organelles (e.g., bacteria and archaea).
  • Eukaryotic cells have a nucleus and other membrane-bound organelles (e.g., plants, animals, fungi, and protists).
  • Key cell structures include the cell membrane, cytoplasm, nucleus, ribosomes, mitochondria, endoplasmic reticulum (ER), Golgi apparatus, lysosomes, and vacuoles.
  • The cell membrane is a selectively permeable barrier that controls the movement of substances in and out of the cell.
  • The cytoplasm is the gel-like substance within the cell where organelles are located.
  • The nucleus contains the cell's genetic material (DNA) and controls cell activities.
  • Ribosomes are responsible for protein synthesis.
  • Mitochondria are the powerhouses of the cell, producing energy through cellular respiration.
  • The endoplasmic reticulum (ER) is involved in protein and lipid synthesis; there are two types: rough ER (with ribosomes) and smooth ER (without ribosomes).
  • The Golgi apparatus processes and packages proteins and lipids.
  • Lysosomes contain enzymes for breaking down cellular waste and debris.
  • Vacuoles store water, nutrients, and waste products.
  • Plant cells have additional structures, including a cell wall (provides support and protection) and chloroplasts (site of photosynthesis).

Cell Processes

- Cellular respiration is the process by which cells convert glucose into energy (ATP) in the presence of oxygen.
- Photosynthesis is the process by which plants and some bacteria use sunlight, water, and carbon dioxide to produce glucose and oxygen.
- Cell division is the process by which cells reproduce; there are two main types: mitosis (for growth and repair) and meiosis (for sexual reproduction).
- Mitosis results in two identical daughter cells, while meiosis results in four genetically different daughter cells (gametes).
- DNA replication is the process by which DNA makes copies of itself before cell division.
- Transcription is the process by which DNA is used as a template to create RNA.
- Translation is the process by which RNA is used to create proteins.
- Osmosis is the movement of water across a semipermeable membrane from an area of high water concentration to an area of low water concentration.
- Diffusion is the movement of molecules from an area of high concentration to an area of low concentration.
- Active transport requires energy to move substances across the cell membrane against their concentration gradient.
- Endocytosis is the process by which cells engulf substances from their surroundings.
- Exocytosis is the process by which cells release substances to their surroundings.

Forces and Motion

• Basic Concepts
  • Force is an interaction that, when unopposed, will change the motion of an object.
  • Motion is the act or process of changing position or orientation in space and time.
  • Newton's Laws of Motion describe the relationship between forces and motion.
  • Speed is the rate at which an object is moving; it is the distance traveled per unit of time (speed = distance/time).
  • Velocity is speed with a direction; it is a vector quantity.
  • Acceleration is the rate at which an object's velocity changes over time; it can be positive (speeding up) or negative (slowing down).

Newton's Laws of Motion

- Newton's First Law (Law of Inertia): An object at rest stays at rest, and an object in motion stays in motion with the same speed and in the same direction unless acted upon by a force.
- Inertia is the tendency of an object to resist changes in its state of motion; it is proportional to the object's mass.
- Newton's Second Law: The acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass (F = ma).
- Force is measured in newtons (N), mass is measured in kilograms (kg), and acceleration is measured in meters per second squared (m/s²).
- Newton's Third Law: For every action, there is an equal and opposite reaction.
- Forces always come in pairs, acting on different objects.

Types of Forces

- Gravity is the force of attraction between objects with mass; it is responsible for weight.
- Friction is a force that opposes motion between surfaces in contact; it can be static (preventing motion) or kinetic (opposing motion).
- Air resistance is a force that opposes the motion of objects through the air.
- Tension is the force transmitted through a string, rope, cable, or wire when it is pulled tight by forces acting from opposite ends.
- Applied force is a force that is applied to an object by a person or another object.
- Normal force is the force exerted by a surface on an object in contact with it; it is perpendicular to the surface.
- Balanced forces result in no change in motion (net force = 0).
- Unbalanced forces result in a change in motion (acceleration).

Periodic Table

• Organization
  • The periodic table is an arrangement of elements in order of increasing atomic number.
  • Elements with similar chemical properties are grouped together in vertical columns called groups or families.
  • Horizontal rows are called periods.
  • The periodic table is organized into blocks (s, p, d, f) based on the electron configurations of the elements.
  • Metals are typically located on the left side of the table, nonmetals on the right side, and metalloids (semimetals) along the staircase line between them.

Elements and Properties

- Each element is represented by its chemical symbol (e.g., H for hydrogen, O for oxygen).
- The atomic number of an element is the number of protons in the nucleus of an atom of that element.
- The atomic mass of an element is the average mass of the atoms of that element, typically measured in atomic mass units (amu).
- Metals are generally shiny, malleable, ductile, and good conductors of heat and electricity.
- Nonmetals are generally dull, brittle, and poor conductors of heat and electricity.
- Metalloids have properties of both metals and nonmetals.
- Group 1 elements (alkali metals) are highly reactive metals.
- Group 2 elements (alkaline earth metals) are also reactive metals, but less so than alkali metals.
- Group 17 elements (halogens) are highly reactive nonmetals.
- Group 18 elements (noble gases) are generally unreactive gases.
- Transition metals are located in the d-block and are known for their variable oxidation states and ability to form colored compounds.
- Lanthanides and actinides are located in the f-block and are often radioactive.
- Electronegativity is a measure of an atom's ability to attract electrons in a chemical bond; it generally increases across a period and decreases down a group.
- Ionization energy is the energy required to remove an electron from an atom; it generally increases across a period and decreases down a group.
- Atomic radius is the size of an atom; it generally decreases across a period and increases down a group.
- Elements in the same group tend to have similar valence electron configurations, which determine their chemical properties.