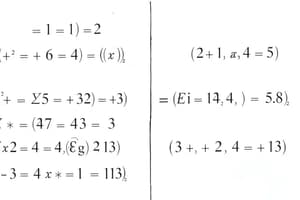Podcast
Questions and Answers
What is the process to solve a system by substitution?
What is the process to solve a system by substitution?
Substitute one equation into the other for y, solve, put into standard form, factor it, simplify with the 0 equation, and substitute the numbers back into the equation for y values.
How do you solve the system by graphing?
How do you solve the system by graphing?
Put two equations into y = form, zoom out to 6, and use the intersect feature.
What is the value of i^2?
What is the value of i^2?
-1
What is the product of (7-3i)(-4+9i)?
What is the product of (7-3i)(-4+9i)?
Signup and view all the answers
Simplify √-49, √-7, √-8.
Simplify √-49, √-7, √-8.
Signup and view all the answers
What is the solution to the equation x^2 + 64 = 0?
What is the solution to the equation x^2 + 64 = 0?
Signup and view all the answers
What is the complex conjugate of a number a + bi?
What is the complex conjugate of a number a + bi?
Signup and view all the answers
What is the quotient of (4 + 5i)/(2 - i)?
What is the quotient of (4 + 5i)/(2 - i)?
Signup and view all the answers
Find the complex conjugate of the complex number 1 - 2i.
Find the complex conjugate of the complex number 1 - 2i.
Signup and view all the answers
What do the points on a graph represent in terms of complex numbers?
What do the points on a graph represent in terms of complex numbers?
Signup and view all the answers
0 has __ values.
0 has __ values.
Signup and view all the answers
=0 has __ values.
=0 has __ values.
Signup and view all the answers
Study Notes
Solving Systems of Equations
- Substitution Method: Solve one equation for y, substitute into the other equation, then solve to find x values.
- Standard Form: Rearranging to set equations to =0 is essential for factoring and finding solutions.
- Graphing Method: Convert equations into y = form, zoom out to see intersections, and utilize intersection features to identify solutions.
Complex Numbers Operations
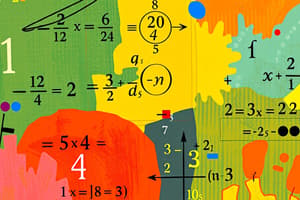
- Imaginary Unit: i² equals -1, fundamental for evaluating complex numbers.
- FOIL Method: Used for multiplying binomials, especially for product calculations like (7-3i)(-4+9i).
- Simplification of Imaginary Roots: Expressions like √-49, √-7, and √-8 simplify to forms involving i, specifically 7i, i√7, and 2i√2.
Solving Quadratic Equations
- Example: For x² + 64 = 0, isolate x² to find x values are +/- 8i.
- Complex Conjugates: Given a complex number a + bi, its conjugate is a - bi; essential when simplifying expressions.
Division of Complex Numbers
- Quotient Calculation: When dividing complex numbers like (4 + 5i)/(2 - i), multiply by the complex conjugate, FOIL the numerator, and simplify the denominator.
- Final Form: Results should always be expressed as a + bi.
Graphing and Complex Analysis
- Graph Interpretation: The x-coordinate indicates the real part, while the y-coordinate represents the imaginary part of the complex number.
- Zeros: The number zero has two values (x = 0 and x = 0), whereas the equation set to zero (=0) has a single solution.
Complex Conjugates
- For specific examples like 1 - 2i, the complex conjugate would be found as 1 + 2i.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.
Description
This quiz covers essential algebra topics such as solving systems of equations using substitution and graphing methods, as well as operations with complex numbers. It also examines the simplification of imaginary roots and the use of complex conjugates in quadratic equations. Test your understanding of these algebraic concepts.
